


 تاريخ الرياضيات
تاريخ الرياضيات
 الرياضيات في الحضارات المختلفة
الرياضيات في الحضارات المختلفة 
 الرياضيات المتقطعة
الرياضيات المتقطعة
 الجبر
الجبر
 الهندسة
الهندسة 
 المعادلات التفاضلية و التكاملية
المعادلات التفاضلية و التكاملية 
 التحليل
التحليل
 علماء الرياضيات
علماء الرياضيات |
Read More
Date: 6-9-2020
Date: 8-5-2020
Date: 1-9-2020
|
The goat problem (or bull-tethering problem) considers a fenced circular field of radius 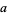 with a goat (or bull, or other animal) tied to a point on the interior or exterior of the fence by means of a tether of length
with a goat (or bull, or other animal) tied to a point on the interior or exterior of the fence by means of a tether of length 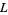 , and asks for the solution to various problems concerning how much of the field can be grazed.
, and asks for the solution to various problems concerning how much of the field can be grazed.

Tieing a goat to a point on the interior of the fence with radius 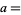 1 using a chain of length
1 using a chain of length  , consider the length of chain that must be used in order to allow the goat to graze exactly one half the area of the field. The answer is obtained by using the equation for a circle-circle intersection
, consider the length of chain that must be used in order to allow the goat to graze exactly one half the area of the field. The answer is obtained by using the equation for a circle-circle intersection
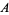 |
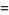 |
 |
(1) |
Taking 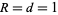 gives
gives
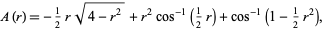 |
(2) |
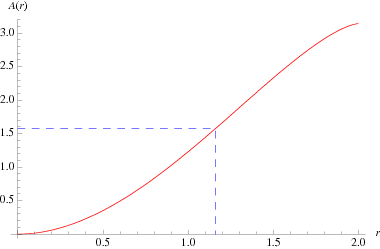
plotted above. Setting 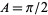 (i.e., half of
(i.e., half of 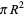 ) leads to the equation
) leads to the equation
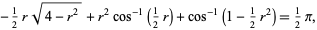 |
(3) |
which cannot be solved exactly, but which has approximate solution
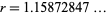 |
(4) |
(OEIS A133731).
Now instead consider tieing the goat to the exterior of the fence (or equivalently, to the exterior of a silo whose horizontal cross section is a circle) with radius  . Assume that
. Assume that  , so that the goat is not able to reach further around than the point on the fence opposite his starting point (Hoffman 1998, where we have replaced Hoffman's bull with a more prosaic goat). The goat may obviously graze inside the interior of a semicircle of radius
, so that the goat is not able to reach further around than the point on the fence opposite his starting point (Hoffman 1998, where we have replaced Hoffman's bull with a more prosaic goat). The goat may obviously graze inside the interior of a semicircle of radius  whose diameter is tangent to the fence. In addition, the goat may graze two area on either side of the semicircle that have the fence as the inner boundary and a circle evolute as the outer boundary. To find the area of this region, assume the fence is oriented so that the farthest point around the circumference that the goat can reach is at position
whose diameter is tangent to the fence. In addition, the goat may graze two area on either side of the semicircle that have the fence as the inner boundary and a circle evolute as the outer boundary. To find the area of this region, assume the fence is oriented so that the farthest point around the circumference that the goat can reach is at position 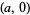 . Now, note that the equation for a circle involute is given by
. Now, note that the equation for a circle involute is given by
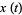 |
 |
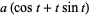 |
(5) |
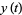 |
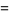 |
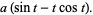 |
(6) |
From geometry, the goat will transition between being radially bound and being bound by pulling tangent to the circle at the point where 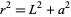 , so
, so
 |
 |
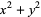 |
(7) |
 |
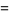 |
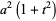 |
(8) |
 |
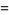 |
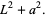 |
(9) |
Equating (8) and (9) and solving for  then shows that this occurs at parameter
then shows that this occurs at parameter 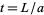 . The area of the involute portion that the goat can graze is then given by
. The area of the involute portion that the goat can graze is then given by
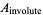 |
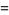 |
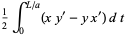 |
(10) |
 |
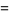 |
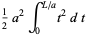 |
(11) |
 |
 |
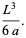 |
(12) |
Adding twice this area to the area of a semicircle of radius 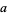 then gives the total area which the goat can graze as
then gives the total area which the goat can graze as
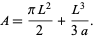 |
(13) |

The grazable areas are illustrated above for a number of ratios of 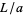 . Note that the case
. Note that the case 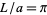 forms a curve that resembles, but is not equivalent to, a cardioid.
forms a curve that resembles, but is not equivalent to, a cardioid.
REFERENCES:
Hoffman, M. E. "The Bull and the Silo: An Application of Curvature." Amer. Math. Monthly 105, 55-58, 1998.
Sloane, N. J. A. Sequence A133731 in "The On-Line Encyclopedia of Integer Sequences."



|
|
|
|
تفوقت في الاختبار على الجميع.. فاكهة "خارقة" في عالم التغذية
|
|
|
|
|
|
|
أمين عام أوبك: النفط الخام والغاز الطبيعي "هبة من الله"
|
|
|
|
|
|
|
قسم شؤون المعارف ينظم دورة عن آليات عمل الفهارس الفنية للموسوعات والكتب لملاكاته
|
|
|