Algebraic Number 

If 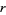 is a root of a nonzero polynomial equation

(1)

where the 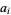s are integers (or equivalently, rational numbers) and  satisfies no similar equation of degree 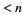, then  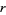is said to be an algebraic number of degree .

A number that is not algebraic is said to be transcendental. If 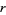 is an algebraic number and 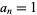, then it is called an algebraic integer.

In general, algebraic numbers are complex, but they may also be real. An example of a complex algebraic number is 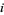, and an example of a real algebraic number is 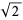, both of which are of degree 2.

The set of algebraic numbers is denoted 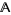 (Wolfram Language), or sometimes  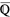(Nesterenko 1999), and is implemented in the Wolfram Language as Algebraics.

A number  can then be tested to see if it is algebraic in the Wolfram Language using the command Element[x, Algebraics]. Algebraic numbers are represented in the Wolfram Language as indexed polynomial roots by the symbol Root[f, n], where 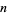 is a number from 1 to the degree of the polynomial (represented as a so-called "pure function") .

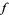Examples of some significant algebraic numbers and their degrees are summarized in the following table.

constant	degree
Conway's constant	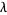71
Delian constant	3
disk covering problem	8
Freiman's constant	2
golden ratio	2
golden ratio conjugate	2
Graham's biggest little hexagon area	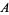10
hard hexagon entropy constant	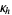24
heptanacci constant	7
hexanacci constant	6
i	2
Lieb's square ice constant	2
logistic map 3-cycle onset	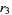2
logistic map 4-cycle onset	2
logistic map 5-cycle onset	22
logistic map 6-cycle onset	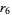40
logistic map 7-cycle onset	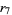114
logistic map 8-cycle onset	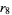12
logistic map 16-cycle onset	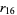240
pentanacci constant	5
plastic constant	3
Pythagoras's constant	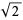2
silver constant	3
silver ratio	2
tetranacci constant	4
Theodorus's constant	2
tribonacci constant	3
twenty-vertex entropy constant	2
Wallis's constant	3

If, instead of being integers, the s in the above equation are algebraic numbers 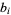, then any root of

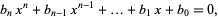(2)

is an algebraic number.

If 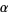 is an algebraic number of degree  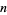satisfying the polynomial equation

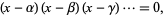(3)

then there are  other algebraic numbers 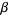, , 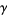... called the conjugates of 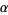. Furthermore, if  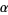satisfies any other algebraic equation, then its conjugates also satisfy the same equation (Conway and Guy 1996).

REFERENCES:

Conway, J. H. and Guy, R. K. "Algebraic Numbers." In The Book of Numbers. New York: Springer-Verlag, pp. 189-190, 1996.

Courant, R. and Robbins, H. "Algebraic and Transcendental Numbers." §2.6 in What Is Mathematics?: An Elementary Approach to Ideas and Methods, 2nd ed. Oxford, England: Oxford University Press, pp. 103-107, 1996.

Ferreirós, J. "The Emergence of Algebraic Number Theory." §3.3 in Labyrinth of Thought: A History of Set Theory and Its Role in Modern Mathematics. Basel, Switzerland: Birkhäuser, pp. 94-99, 1999.

Hancock, H. Foundations of the Theory of Algebraic Numbers, Vol. 1: Introduction to the General Theory. New York: Macmillan, 1931.

Hancock, H. Foundations of the Theory of Algebraic Numbers, Vol. 2: The General Theory. New York: Macmillan, 1932.

Koch, H. Number Theory: Algebraic Numbers and Functions. Providence, RI: Amer. Math. Soc., 2000.

Nagell, T. Introduction to Number Theory. New York: Wiley, p. 35, 1951.

Narkiewicz, W. Elementary and Analytic Number Theory of Algebraic Numbers. Warsaw: Polish Scientific Publishers, 1974.

Nesterenko, Yu. V. A Course on Algebraic Independence: Lectures at IHP 1999. Unpublished manuscript. 1999.

Wagon, S. "Algebraic Numbers." §10.5 in Mathematica in Action. New York: W. H. Freeman, pp. 347-353, 1991.

Wolfram, S. A New Kind of Science. Champaign, IL: Wolfram Media, p. 1168, 2002.