Diatomic Molecules in Three Dimensions

Consider the free rotation of a diatomic molecule consisting of two atoms of mass m₁ and m₂, respectively, separated by a distance a. Assume that the molecule is rigid with center of mass fixed.

a) Starting from the kinetic energy ε_k, where

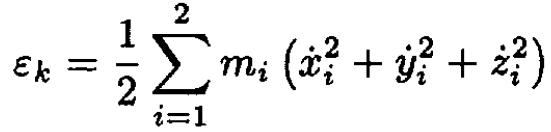
derive the kinetic energy of this system in spherical coordinates and show that

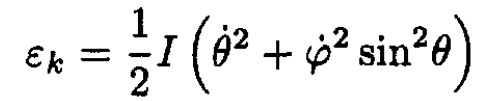
where I is the moment of inertia. Express I in terms of m₁, m₂, and a.

b) Derive the canonical conjugate momenta p_θ and p_φ. Express the Hamiltonian of this system in terms of p_θ, p_φ, θ, φ, and I.

c) The classical partition function is defined as

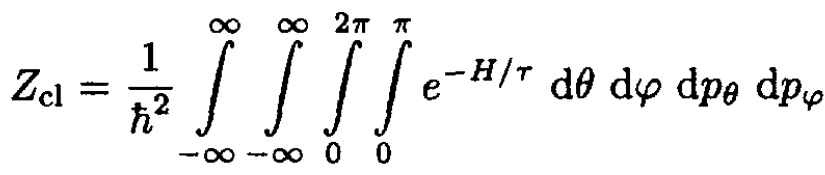
Calculate Z_cl. Calculate the heat capacity for a system of N molecules.

d) Assume now that the rotational motion of the molecule is described by quantum mechanics. Write the partition function in this case, taking into account the degeneracy of each state. Calculate the heat capacity of a system of N molecules in the limit of low and high temperatures and compare them to the classical result.

SOLUTION

a) We first transform the expression of the kinetic energy ε_k:

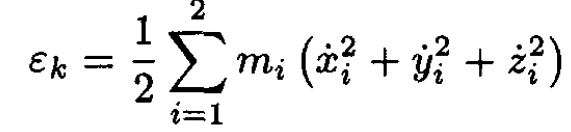(1)

where x_i, y_i, z_i are the Cartesian coordinates of the molecule in the frame with the c.m. at the origin to spherical coordinates:

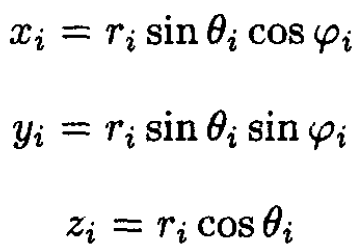(2)

For the rigid diatom,

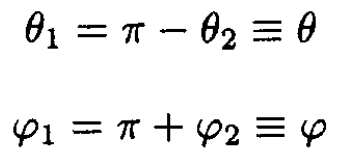
We may substitute (2) into (1), obtaining

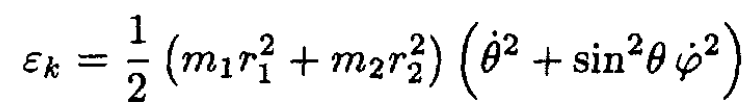(3)

Using the definition of c.m., we may write

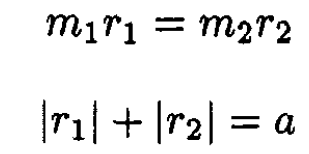(4)

yielding

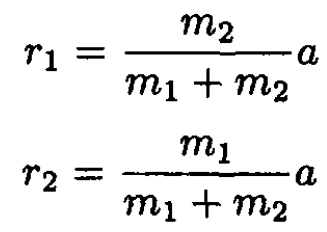(5)

Then (3) becomes

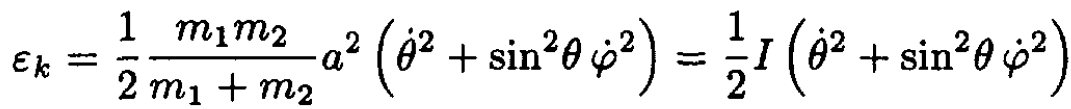(6)

with

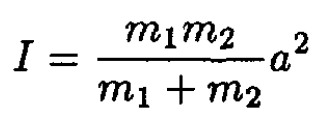
b) In order to find the conjugate momenta p_θ, p_φ, we must compute the Lagrangian

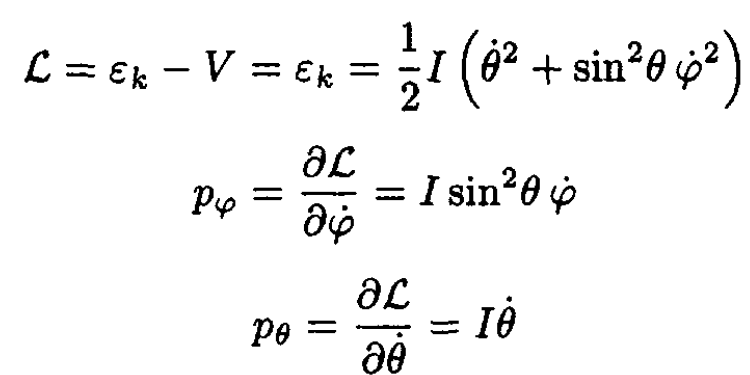(7)

Expressing 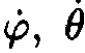 through p_φ, p_θ

we may rewrite the Hamiltonian as

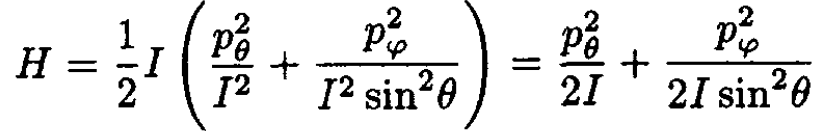(8)

c) The single-diatom partition function may be computed as follows:

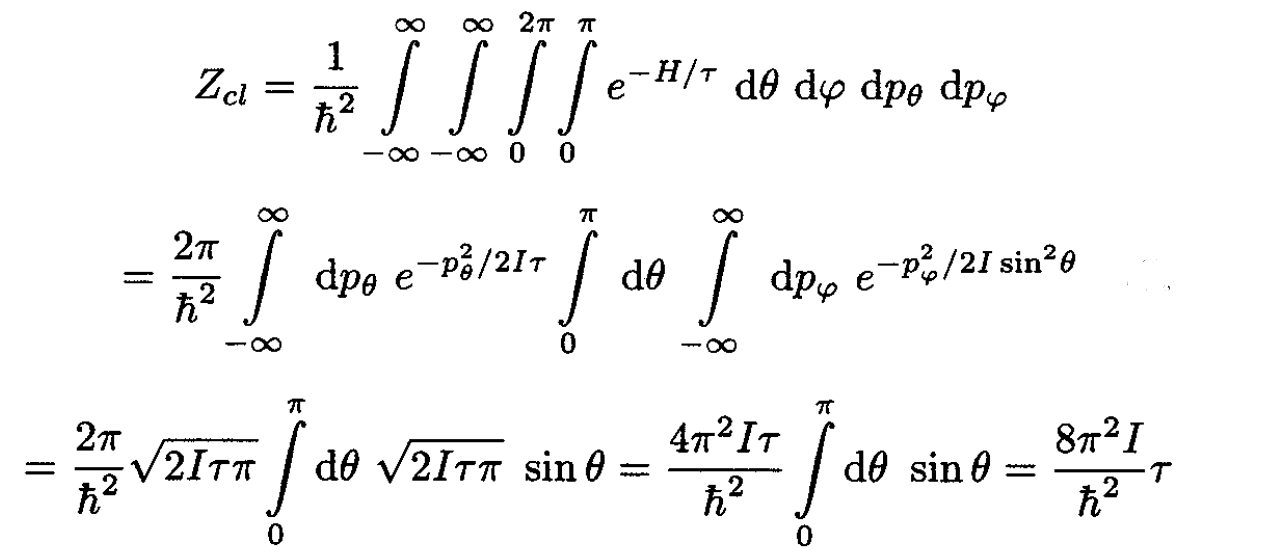(9)

Now the free energy F for N such classical molecules may be found from

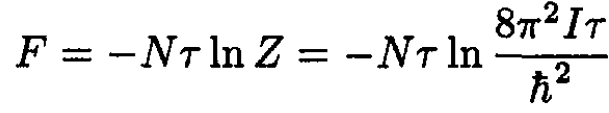(10)

The entropy S is then

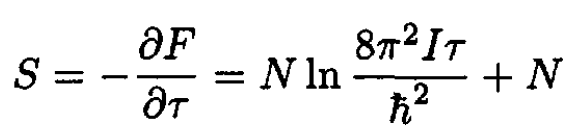(11)

and the energy E and heat capacity C are

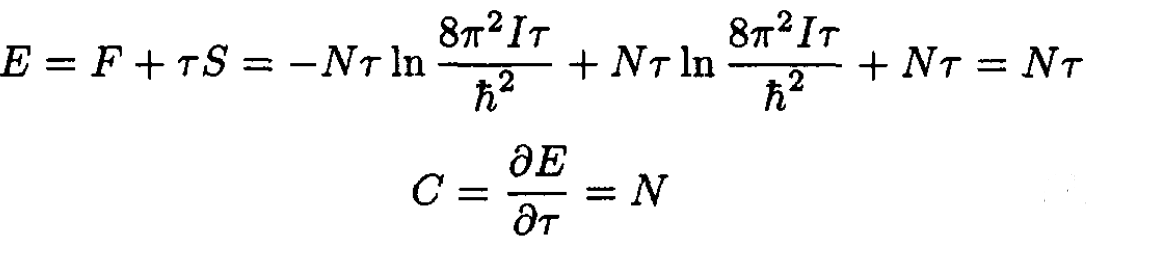(12)

d) For the quantum case the Schrodinger equation for a rigid rotator

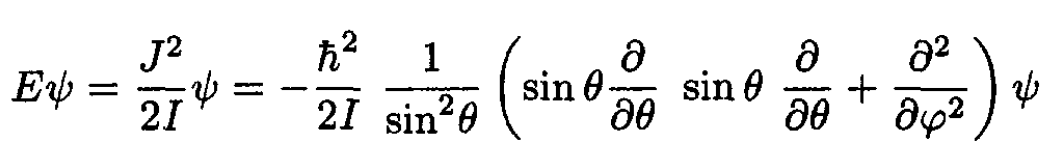(13)

admits the standard solution

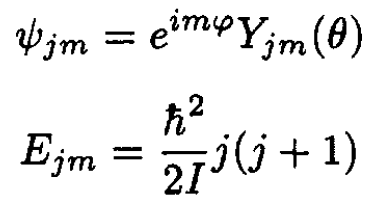(14)

where each of the energy states is (2j + 1)-degenerate. The partition function Z_q is given by

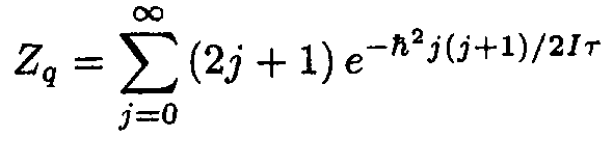(15)

For low temperatures we may neglect high-order terms and write

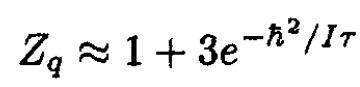
where we left only terms with j = 0 and j = 1. For N molecules we find for the free energy that

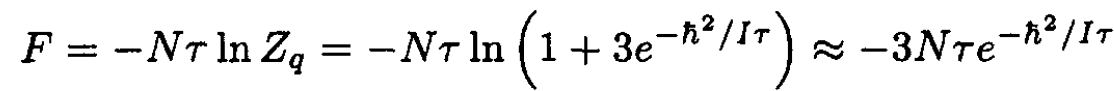(16)

The energy E and heat capacity C are then

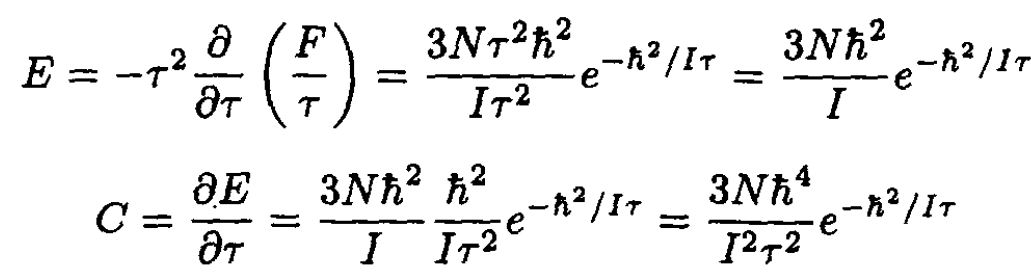(17)

So, at low temperatures the heat capacity corresponding to the rotational degrees of freedom is exponentially small. This implies that there would be no difference, in this limit, between the heat capacity for monatomic and diatomic molecules. In the opposite case, at high temperatures, h²/2I << τ, the sum may be replaced by an integral:

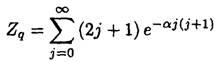(18)

where α ≡ h²/2I τ. Proceeding from (18), we have

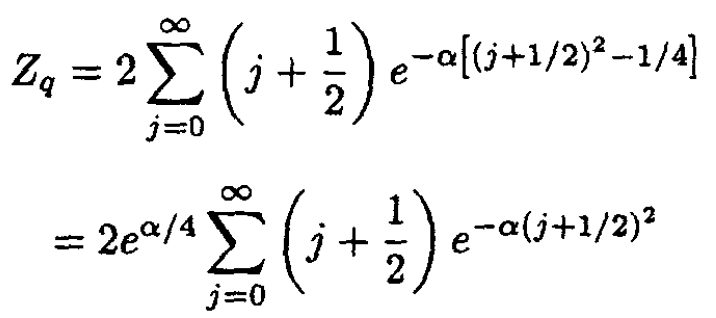(19)

Replacing the sum by an integral, we obtain

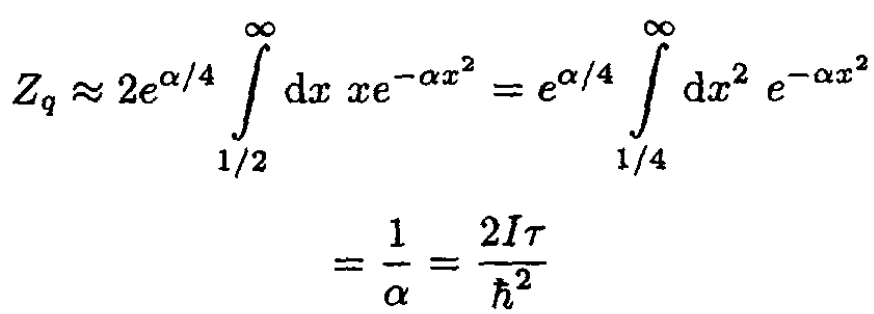(20)

Therefore, in the classical limit (high temperatures),

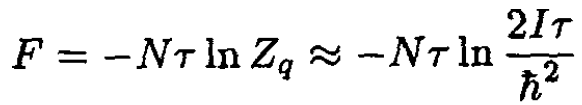(21)

The energy E and heat capacity C are given by

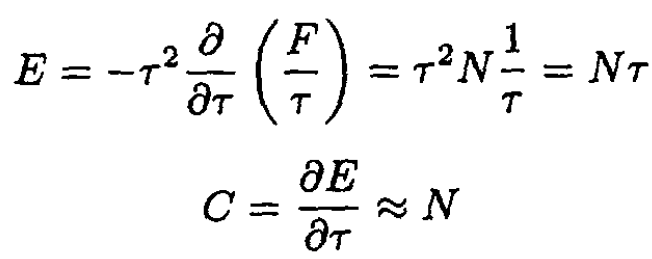(22)

We see that this is the same as found in (12). Since we expect a heat capacity per degree of freedom of 1/2, we see that there are two degrees of freedom for each molecule since

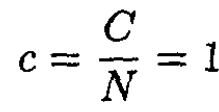
They correspond to the two rotational degrees of freedom of a classical rod. (There are no spatial degrees of freedom since the molecule is considered fixed.)