Cardinal Number

In common usage, a cardinal number is a number used in counting (a counting number), such as 1, 2, 3, ....

In formal set theory, a cardinal number (also called "the cardinality") is a type of number defined in such a way that any method of counting sets using it gives the same result. (This is not true for the ordinal numbers.) In fact, the cardinal numbers are obtained by collecting all ordinal numbers which are obtainable by counting a given set. A set has 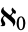 (aleph-0) members if it can be put into a one-to-one correspondence with the finite ordinal numbers. The cardinality of a set is also frequently referred to as the "power" of a set (Moore 1982, Dauben 1990, Suppes 1972).

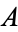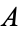In Georg Cantor's original notation, the symbol for a set  annotated with a single overbar  indicated  stripped of any structure besides order, hence it represented the order type of the set. A double overbar  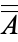then indicated stripping the order from the set and thus indicated the cardinal number of the set. However, in modern notation, the symbol  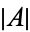is used to denote the cardinal number of set.

Cantor, the father of modern set theory, noticed that while the ordinal numbers 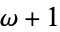, , ... were bigger than omega in the sense of order, they were not bigger in the sense of equipollence. This led him to study what would come to be called cardinal numbers. He called the ordinals 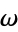, , 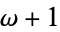... that are equipollent to the integers "the second number class" (as opposed to the finite ordinals, which he called the "first number class"). Cantor showed

1. The second number class is bigger than the first.

2. There is no class bigger than the first number class and smaller than the second.

3. The class of real numbers is bigger than the first number class.

One of the first serious mathematical definitions of cardinal was the one devised by Gottlob Frege and Bertrand Russell, who defined a cardinal number 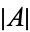 as the set of all sets equipollent to 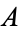. (Moore 1982, p. 153; Suppes 1972, p. 109). Unfortunately, the objects produced by this definition are not sets in the sense of Zermelo-Fraenkel set theory, but rather "proper classes" in the terminology of von Neumann.

Tarski (1924) proposed to instead define a cardinal number by stating that every set 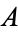 is associated with a cardinal number 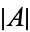, and two sets  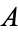and 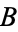 have the same cardinal number iff they are equipollent (Moore 1982, pp. 52 and 214; Rubin 1967, p. 266; Suppes 1972, p. 111). The problem is that this definition requires a special axiom to guarantee that cardinals exist.

A. P. Morse and Dana Scott defined cardinal number by letting 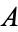 be any set, then calling  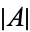the set of all sets equipollent to  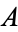and of least possible rank (Rubin 1967, p. 270).

It is possible to associate cardinality with a specific set, but the process required either the axiom of foundation or the axiom of choice. However, these are two of the more controversial Zermelo-Fraenkel axioms. With the axiom of choice, the cardinals can be enumerated through the ordinals. In fact, the two can be put into one-to-one correspondence. The axiom of choice implies that every set can be well ordered and can therefore be associated with an ordinal number.

This leads to the definition of cardinal number for a set 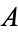 as the least ordinal number  such that 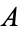 and  are equipollent. In this model, the cardinal numbers are just the initial ordinals. This definition obviously depends on the axiom of choice, because if the axiom of choice is not true, then there are sets that cannot be well ordered. Cantor believed that every set could be well ordered and used this correspondence to define the 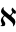s ("alephs"). For any ordinal number , .

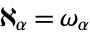An inaccessible cardinal cannot be expressed in terms of a smaller number of smaller cardinals.

REFERENCES:

Cantor, G. Über unendliche, lineare Punktmannigfaltigkeiten, Arbeiten zur Mengenlehre aus dem Jahren 1872-1884. Leipzig, Germany: Teubner, 1884.

Conway, J. H. and Guy, R. K. "Cardinal Numbers." In The Book of Numbers. New York: Springer-Verlag, pp. 277-282, 1996.

Courant, R. and Robbins, H. "Cantor's 'Cardinal Numbers.' " §2.4.3 in What Is Mathematics?: An Elementary Approach to Ideas and Methods, 2nd ed. Oxford, England: Oxford University Press, pp. 83-86, 1996.

Dauben, J. W. Georg Cantor: His Mathematics and Philosophy of the Infinite. Princeton, NJ: Princeton University Press, 1990.

Ferreirós, J. "The Notion of Cardinality and the Continuum Hypothesis." Ch. 6 in Labyrinth of Thought: A History of Set Theory and Its Role in Modern Mathematics. Basel, Switzerland: Birkhäuser, pp. 171-214, 1999.

Moore, G. H. Zermelo's Axiom of Choice: Its Origin, Development, and Influence. New York: Springer-Verlag, 1982.

Rubin, J. E. Set Theory for the Mathematician. New York: Holden-Day, 1967.

Suppes, P. Axiomatic Set Theory. New York: Dover, 1972.

Tarski, A. "Sur quelques théorèmes qui équivalent à l'axiome du choix." Fund. Math. 5, 147-154, 1924.