The improvement of the convergence properties of a series, also called convergence acceleration or accelerated convergence, such that a series reaches its limit to within some accuracy with fewer terms than required before. Convergence improvement can be effected by forming a linear combination with a series whose sum is known. Useful sums include

			(1)
			(2)
			(3)
			(4)

Kummer's transformation takes a convergent series

(5)

and another convergent series

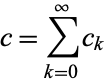(6)

with known 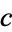 such that

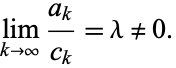(7)

Then a series with more rapid convergence to the same value is given by

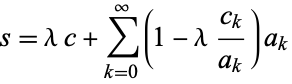(8)

(Abramowitz and Stegun 1972).

The Euler transform takes a convergent alternating series

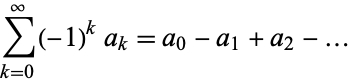(9)

into a series with more rapid convergence to the same value to

(10)

where

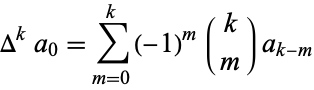(11)

(Abramowitz and Stegun 1972; Beeler et al. 1972).

A general technique that can be used to acceleration converge of series is to expand them in a Taylor series about infinity and interchange the order of summation. In cases where a symbolic form for the Taylor series can be found, this come sometimes even allow the sum over the original variable to be done symbolically. For example, consider the case of the sum

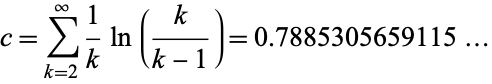(12)

(OEIS A085361) that arises in the definition of the Alladi-Grinstead constant. The summand can be expanded about infinity to get

			(13)
			(14)

Interchanging the order of summation then gives

			(15)
			(16)

where 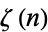 is the Riemann zeta function, which converges much more rapidly.

A transformations of the form

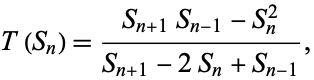(17)

where

(18)

is the th partial sum of a sequence {a_k}_(k=0)^infty" src="https://mathworld.wolfram.com/images/equations/ConvergenceImprovement/Inline28.gif" style="height:17px; width:42px" />, can often be useful for improving series convergence (Hamming 1986, p. 205). In particular, 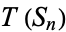 can be written

			(19)
			(20)

The application of this transformation can be efficiently carried out using Wynn's epsilon method. Letting 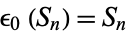, , 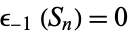and

(21)

for 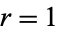, 2, ... (correcting the typo of Hamming 1986, p. 206). The values of  are there equivalent to the results of applying 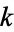 transformations to the sequence  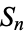(Hamming 1986, p. 206).

Given a series of the form

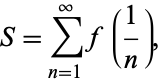(22)

where 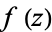 is an analytic at 0 and on the closed unit disk, and

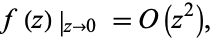(23)

then the series can be rearranged to

			(24)
			(25)
			(26)

where

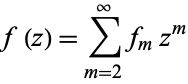(27)

is the Maclaurin series of  and 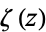 is the Riemann zeta function (Flajolet and Vardi 1996). The transformed series exhibits geometric convergence. Similarly, if  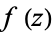is analytic in  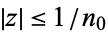for some positive integer 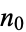, then

(28)

which converges geometrically (Flajolet and Vardi 1996). Equation (28) can also be used to further accelerate the convergence of series (◇).

REFERENCES:

Abramowitz, M. and Stegun, I. A. (Eds.). Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, p. 16, 1972.

Arfken, G. Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 288-289, 1985.

Beeler et al. Item 120 in Beeler, M.; Gosper, R. W.; and Schroeppel, R. HAKMEM. Cambridge, MA: MIT Artificial Intelligence Laboratory, Memo AIM-239, p. 55, Feb. 1972. http://www.inwap.com/pdp10/hbaker/hakmem/series.html#item120.

Flajolet, P. and Vardi, I. "Zeta Function Expansions of Classical Constants." Unpublished manuscript. 1996. http://algo.inria.fr/flajolet/Publications/landau.ps.

Hamming, R. W. Numerical Methods for Scientists and Engineers, 2nd ed. New York: Dover, pp. 206-207, 1986.

Shanks, D. "Nonlinear Transformations of Divergent and Slowly Convergent Sequences." J. Math. Phys. 34, 1-42, 1955.

Sloane, N. J. A. Sequence A085361 in "The On-Line Encyclopedia of Integer Sequences."

اخر الاخبار

اخبار العتبة العباسية المقدسة

قسم صناعة الشبابيك يشرع بإدامة شباكين قديمين لمرقد الإمامين العسكريين (عليهما السلام)

فرقة العباس (عليه السلام) تختتم خدماتها المقدمة للزائرين في سامراء

موكب العتبتين المقدستين يحيي ذكرى استشهاد الإمام الهادي (عليه السلام) في سامراء

العتبة العسكرية المقدسة تكرّم وفد العتبة العباسية المقدسة لمشاركته في تقديم الخدمات للزائرين

المزيد

الآخبار الصحية

كنز غذائي على طبقك.. ماذا تفعل السبانخ بجسمك؟

ما لا يتوقعه أحد.. "سر غذائي" في الجبن الدسم

لتحسين الهضم والنوم.. هذا أفضل وقت لتناول العشاء

10 طرق بسيطة وغير مألوفة لحرق المزيد من السعرات يوميا

المزيد

الآخبار التكنلوجية

سابقة بعالم الطيران.. طائرة تقرر الهبوط بنفسها بعد ظرف طارئ

"الإنفلونزا الخارقة" تكتسح دول العالم.. وتثير قلقا كبيرا

مرحلة ما قبل الأعراض.. دواء جديد يستهدف "شرارة" الزهايمر

إزالة ثاني أكسيد الكربون من الغلاف الجوي.. ما دور المحيطات والبحار؟

المزيد