


 تاريخ الرياضيات
تاريخ الرياضيات
 الرياضيات في الحضارات المختلفة
الرياضيات في الحضارات المختلفة 
 الرياضيات المتقطعة
الرياضيات المتقطعة
 الجبر
الجبر
 الهندسة
الهندسة 
 المعادلات التفاضلية و التكاملية
المعادلات التفاضلية و التكاملية 
 التحليل
التحليل
 علماء الرياضيات
علماء الرياضيات |
Read More
Date: 11-11-2020
Date: 5-6-2020
Date: 1-1-2020
|
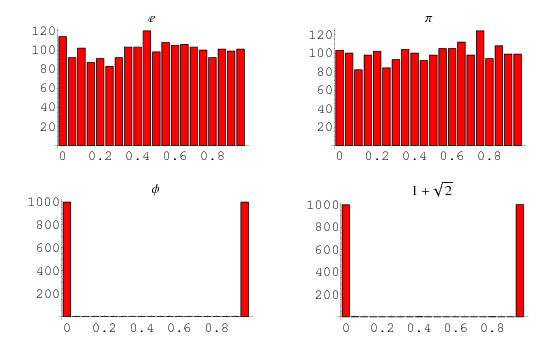
Hardy and Littlewood (1914) proved that the sequence 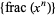 , where
, where 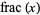 is the fractional part, is equidistributed for almost all real numbers
is the fractional part, is equidistributed for almost all real numbers  (i.e., the exceptional set has Lebesgue measure zero). Exceptional numbers include the positive integers, the silver ratio
(i.e., the exceptional set has Lebesgue measure zero). Exceptional numbers include the positive integers, the silver ratio 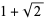 (Finch 2003), and the golden ratio
(Finch 2003), and the golden ratio  . The plots above illustrate the distribution of
. The plots above illustrate the distribution of 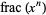 for
for 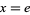 ,
, 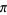 ,
, 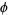 , and
, and  . Candidate members of the measure one set are easy to find, but difficult to prove. However, Levin has explicitly constructed such an example (Drmota and Tichy 1997).
. Candidate members of the measure one set are easy to find, but difficult to prove. However, Levin has explicitly constructed such an example (Drmota and Tichy 1997).
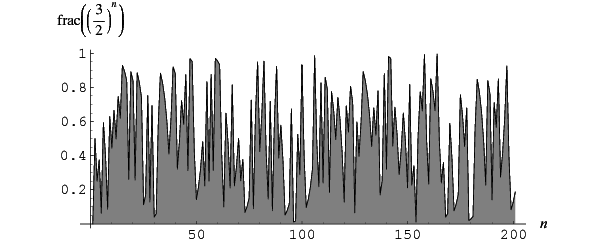
The properties of 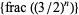 , the simplest such sequence for a rational number
, the simplest such sequence for a rational number 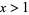 , have been extensively studied (Finch 2003). The first few terms are 0, 1/2, 1/4, 3/8, 1/16, 19/32, 25/64, 11/128, 161/256, 227/512, ... (OEIS A002380 and A000079; Pillai 1936; Lehmer 1941), plotted above (Wolfram 2002, pp. 121-122). For example,
, have been extensively studied (Finch 2003). The first few terms are 0, 1/2, 1/4, 3/8, 1/16, 19/32, 25/64, 11/128, 161/256, 227/512, ... (OEIS A002380 and A000079; Pillai 1936; Lehmer 1941), plotted above (Wolfram 2002, pp. 121-122). For example, 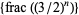 has infinitely many accumulation points in both
has infinitely many accumulation points in both  and
and ![[1/2,1]](https://mathworld.wolfram.com/images/equations/PowerFractionalParts/Inline15.gif) (Pisot 1938, Vijayaraghavan 1941). Furthermore, Flatto et al. (1995) proved that any subinterval of
(Pisot 1938, Vijayaraghavan 1941). Furthermore, Flatto et al. (1995) proved that any subinterval of ![[0,1]](https://mathworld.wolfram.com/images/equations/PowerFractionalParts/Inline16.gif) containing all but at most finitely many accumulation points of
containing all but at most finitely many accumulation points of  must have length at least 1/3. Surprisingly, the sequence
must have length at least 1/3. Surprisingly, the sequence 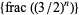 is also connected with the Collatz problem and with Waring's problem.
is also connected with the Collatz problem and with Waring's problem.
Numbers of the form 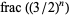 , where
, where 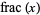 is the fractional part, appear in Waring's problem. In particular, Waring's problem can be solved completely if the inequality
is the fractional part, appear in Waring's problem. In particular, Waring's problem can be solved completely if the inequality
![frac[(3/2)^n]<=1-(3/4)^n](https://mathworld.wolfram.com/images/equations/PowerFractionalParts/NumberedEquation1.gif) |
holds. No counterexample to this inequality is known, and it is even believed that it can be extended to
![(3/4)^n<frac[(3/2)^n]<1-(3/4)^n](https://mathworld.wolfram.com/images/equations/PowerFractionalParts/NumberedEquation2.gif) |
for 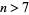 (Bennett 1993, 1994; Finch 2003). Furthermore, the constant 3/4 can be decreased to 0.5769 (Beukers 1981, Dubitskas 1990). Unfortunately, these inequalities have not been proved.
(Bennett 1993, 1994; Finch 2003). Furthermore, the constant 3/4 can be decreased to 0.5769 (Beukers 1981, Dubitskas 1990). Unfortunately, these inequalities have not been proved.
REFERENCES:
Bennett, M. A. "Fractional Parts of Powers of Rational Numbers." Math. Proc. Cambridge Philos. Soc. 114, 191-201, 1993.
Bennett, M. A. "An Ideal Waring Problem with Restricted Summands." Acta Arith. 66, 125-132, 1994.
Beukers, F. "Fractional Parts of Powers of Rational Numbers." Math. Proc. Cambridge Philos. Soc. 90, 13-20, 1981.
Drmota, M. and Tichy, R. F. Sequences, Discrepancies and Applications. New York: Springer-Verlag, 1997.
Dubitskas, A. K. "A Lower Bound for the Quantity 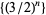 ." Russian Math. Survey 45, 163-164, 1990.
." Russian Math. Survey 45, 163-164, 1990.
Finch, S. R. "Powers of 3/2 Modulo One." §2.30.1 in Mathematical Constants. Cambridge, England: Cambridge University Press, pp. 194-199, 2003.
Flatto, L.; Lagarias, J. C.; Pollington, A. D. "On the Range of Fractional Parts  ." Acta Arith. 70, 125-147, 1995.
." Acta Arith. 70, 125-147, 1995.
Hardy, G. H. and Littlewood, J. E. "Some Problems of Diophantine Approximation." Acta Math. 37, 193-239, 1914.
Lehmer, D. H. Guide to Tables in the Theory of Numbers. Bulletin No. 105. Washington, DC: National Research Council, p. 82, 1941.
Pillai, S. S. "On Waring's Problem." J. Indian Math. Soc. 2, 16-44, 1936.
Pisot, C. "La répartition modulo 1 et les nombres algébriques." Annali di Pisa 7, 205-248, 1938.
Sloane, N. J. A. Sequences A000079/M1129 and A002380/M2235 in "The On-Line Encyclopedia of Integer Sequences."
Vijayaraghavan, T. "On the Fractional Parts of the Powers of a Number (I)." J. London Math. Soc. 15, 159-160, 1940.
Vijayaraghavan, T. "On the Fractional Parts of the Powers of a Number (II)." Proc. Cambridge Phil. Soc. 37, 349-357, 1941.
Vijayaraghavan, T. "On the Fractional Parts of the Powers of a Number (III)." J. London Math. Soc. 17, 137-138, 1942.
Wolfram, S. A New Kind of Science. Champaign, IL: Wolfram Media, pp. 121-122, 2002.



|
|
|
|
مخاطر عدم علاج ارتفاع ضغط الدم
|
|
|
|
|
|
|
اختراق جديد في علاج سرطان البروستات العدواني
|
|
|
|
|
|
|
مدرسة دار العلم.. صرح علميّ متميز في كربلاء لنشر علوم أهل البيت (عليهم السلام)
|
|
|