Partial Differential Equation
A partial differential equation (PDE) is an equation involving functions and their partial derivatives; for example, the wave equation
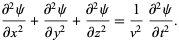 |
(1)
|
Some partial differential equations can be solved exactly in the Wolfram Language using DSolve[eqn, y, ![<span style=]() {" src="http://mathworld.wolfram.com/images/equations/PartialDifferentialEquation/Inline1.gif" style="height:14px; width:5px" />x1, x2
{" src="http://mathworld.wolfram.com/images/equations/PartialDifferentialEquation/Inline1.gif" style="height:14px; width:5px" />x1, x2![<span style=]() }" src="http://mathworld.wolfram.com/images/equations/PartialDifferentialEquation/Inline2.gif" style="height:14px; width:5px" />], and numerically using NDSolve[eqns, y,
}" src="http://mathworld.wolfram.com/images/equations/PartialDifferentialEquation/Inline2.gif" style="height:14px; width:5px" />], and numerically using NDSolve[eqns, y, ![<span style=]() {" src="http://mathworld.wolfram.com/images/equations/PartialDifferentialEquation/Inline3.gif" style="height:14px; width:5px" />x, xmin, xmax
{" src="http://mathworld.wolfram.com/images/equations/PartialDifferentialEquation/Inline3.gif" style="height:14px; width:5px" />x, xmin, xmax![<span style=]() }" src="http://mathworld.wolfram.com/images/equations/PartialDifferentialEquation/Inline4.gif" style="height:14px; width:5px" />,
}" src="http://mathworld.wolfram.com/images/equations/PartialDifferentialEquation/Inline4.gif" style="height:14px; width:5px" />, ![<span style=]() {" src="http://mathworld.wolfram.com/images/equations/PartialDifferentialEquation/Inline5.gif" style="height:14px; width:5px" />t, tmin, tmax
{" src="http://mathworld.wolfram.com/images/equations/PartialDifferentialEquation/Inline5.gif" style="height:14px; width:5px" />t, tmin, tmax![<span style=]() }" src="http://mathworld.wolfram.com/images/equations/PartialDifferentialEquation/Inline6.gif" style="height:14px; width:5px" />].
}" src="http://mathworld.wolfram.com/images/equations/PartialDifferentialEquation/Inline6.gif" style="height:14px; width:5px" />].
In general, partial differential equations are much more difficult to solve analytically than are ordinary differential equations. They may sometimes be solved using a Bäcklund transformation, characteristics, Green's function, integral transform, Lax pair, separation of variables, or--when all else fails (which it frequently does)--numerical methods such as finite differences.
Fortunately, partial differential equations of second-order are often amenable to analytical solution. Such PDEs are of the form
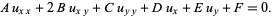 |
(2)
|
Linear second-order PDEs are then classified according to the properties of the matrix
![Z=[A B; B C]](http://mathworld.wolfram.com/images/equations/PartialDifferentialEquation/NumberedEquation3.gif) |
(3)
|
as elliptic, hyperbolic, or parabolic.
If 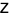 is a positive definite matrix, i.e.,
is a positive definite matrix, i.e., 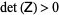 , the PDE is said to be elliptic. Laplace's equation and Poisson's equation are examples. Boundary conditions are used to give the constraint
, the PDE is said to be elliptic. Laplace's equation and Poisson's equation are examples. Boundary conditions are used to give the constraint 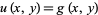 on
on  , where
, where
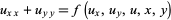 |
(4)
|
holds in 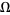 .
.
If det , the PDE is said to be hyperbolic. The wave equation is an example of a hyperbolic partial differential equation. Initial-boundary conditions are used to give
, the PDE is said to be hyperbolic. The wave equation is an example of a hyperbolic partial differential equation. Initial-boundary conditions are used to give
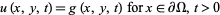 |
(5)
|
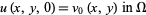 |
(6)
|
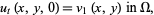 |
(7)
|
where
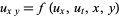 |
(8)
|
holds in  .
.
If det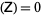 , the PDE is said to be parabolic. The heat conduction equation equation and other diffusion equations are examples. Initial-boundary conditions are used to give
, the PDE is said to be parabolic. The heat conduction equation equation and other diffusion equations are examples. Initial-boundary conditions are used to give
 |
(9)
|
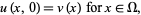 |
(10)
|
where
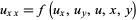 |
(11)
|
holds in 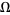 .
.
The following are examples of important partial differential equations that commonly arise in problems of mathematical physics.
Benjamin-Bona-Mahony equation
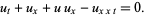 |
(12)
|
Biharmonic equation
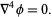 |
(13)
|
Boussinesq equation
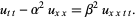 |
(14)
|
Cauchy-Riemann equations
Chaplygin's equation
 |
(17)
|
Euler-Darboux equation
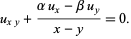 |
(18)
|
Heat conduction equation
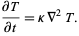 |
(19)
|
Helmholtz differential equation
 |
(20)
|
Klein-Gordon equation
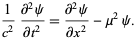 |
(21)
|
Korteweg-de Vries-Burgers equation
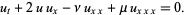 |
(22)
|
Korteweg-de Vries equation
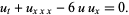 |
(23)
|
Krichever-Novikov equation
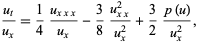 |
(24)
|
where
 |
(25)
|
Laplace's equation
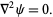 |
(26)
|
Lin-Tsien equation
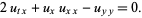 |
(27)
|
Sine-Gordon equation
 |
(28)
|
Spherical harmonic differential equation
![[1/(sintheta)partial/(partialtheta)(sinthetapartial/(partialtheta))+1/(sin^2theta)(partial^2)/(partialphi^2)+l(l+1)]u=0.](http://mathworld.wolfram.com/images/equations/PartialDifferentialEquation/NumberedEquation27.gif) |
(29)
|
Tricomi equation
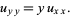 |
(30)
|
Wave equation
 |
(31)
|
REFERENCES:
Arfken, G. "Partial Differential Equations of Theoretical Physics." §8.1 in Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 437-440, 1985.
Bateman, H. Partial Differential Equations of Mathematical Physics. New York: Dover, 1944.
Conte, R. "Exact Solutions of Nonlinear Partial Differential Equations by Singularity Analysis." 13 Sep 2000. http://arxiv.org/abs/nlin.SI/0009024.
Kamke, E. Differentialgleichungen Lösungsmethoden und Lösungen, Bd. 2: Partielle Differentialgleichungen ester Ordnung für eine gesuchte Function. New York: Chelsea, 1974.
Folland, G. B. Introduction to Partial Differential Equations, 2nd ed. Princeton, NJ: Princeton University Press, 1996.
Kevorkian, J. Partial Differential Equations: Analytical Solution Techniques, 2nd ed. New York: Springer-Verlag, 2000.
Morse, P. M. and Feshbach, H. "Standard Forms for Some of the Partial Differential Equations of Theoretical Physics." Methods of Theoretical Physics, Part I. New York: McGraw-Hill, pp. 271-272, 1953.
Polyanin, A.; Zaitsev, V.; and Moussiaux, A. Handbook of First-Order Partial Differential Equations. New York: Gordon and Breach, 2001.
Press, W. H.; Flannery, B. P.; Teukolsky, S. A.; and Vetterling, W. T. "Partial Differential Equations." Ch. 19 in Numerical Recipes in FORTRAN: The Art of Scientific Computing, 2nd ed. Cambridge, England: Cambridge University Press, pp. 818-880, 1992.
Sobolev, S. L. Partial Differential Equations of Mathematical Physics. New York: Dover, 1989.
Sommerfeld, A. Partial Differential Equations in Physics. New York: Academic Press, 1964.
Taylor, M. E. Partial Differential Equations, Vol. 1: Basic Theory. New York: Springer-Verlag, 1996.
Taylor, M. E. Partial Differential Equations, Vol. 2: Qualitative Studies of Linear Equations. New York: Springer-Verlag, 1996.
Taylor, M. E. Partial Differential Equations, Vol. 3: Nonlinear Equations. New York: Springer-Verlag, 1996.
Trott, M. "The Mathematica Guidebooks Additional Material: Various Time-Dependent PDEs." http://www.mathematicaguidebooks.org/additions.shtml#N_1_06.
Webster, A. G. Partial Differential Equations of Mathematical Physics, 2nd corr. ed. New York: Dover, 1955.
Weisstein, E. W. "Books about Partial Differential Equations." http://www.ericweisstein.com/encyclopedias/books/PartialDifferentialEquations.html.
Zwillinger, D. Handbook of Differential Equations, 3rd ed. Boston, MA: Academic Press, 1997.
 الاكثر قراءة في المعادلات التفاضلية الجزئية
الاكثر قراءة في المعادلات التفاضلية الجزئية
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة


