Term symbols

  If we know (2S ‏+ 1), L and J for an energy state, we can write the full term symbol. This is done by writing the symbol of the value of L (i.e. S, P, D . . .) with the value of (2S ‏+ 1) as a left-superscript and the value of J as a rightsubscript.   Thus, the electronic ground state of carbon is 3P⁰ (‘triplet P zero’) denoting L = 1,(2S+1) = 3 (i.e. S = 1) and J = 0. Different values of J denote different Term symbols.  If we know (2S +‏ 1), L and J for an energy state, we can write the full term symbol. This is done by writing the symbol of the value of L (i.e. S, P, D . . .) with the value of  (2S ‏+ 1) as a left-superscript and the value of J as a rightsubscript. Thus, the electronic ground state of carbon is 3P⁰ (‘triplet P zero’) denoting L = 1, (2S ‏+ 1) = 3 (i.e. S = 1) and J = 0. Different values of J denote different levels within the term, i.e. ^(2S+‏1)L_J1 ,^(2S‏+1)L_J2 . . . , the levels having different energies. Inorganic chemists often omit the value of J and refer to a ^(2S‏+1)L term; we shall usually follow this practice in this book. Now we look in detail at the electronic ground states of atoms with Z = 1 to 10.

Hydrogen (Z = 1)

   A hydrogen atom has an electronic configuration of 1s¹; for the electron, l = 0 so L must be 0 and, therefore, we have an S term. The total spin quantum number S = 1/2 so) 2S +‏ 1) = 2 (a doublet term). The only possible value of J is 1/2, and so the term symbol for the hydrogen atom is ²S_1/2.

Helium (Z = 2)

For helium (1s²), both electrons have l = 0, so L = 0. Two electrons both with n = 1 and l = 0 must have m_s = ‏1/2 and^_1/2, so S = 0 and(2S +‏ 1) = 1 (a singlet term). The only value of J is 0, and so the term symbol is ¹S₀. Thus, the ns² configuration, having L = 0, S = 0 and J = 0, will contribute nothing to the term symbol in lithium and lateratoms. The same conclusion can be drawn for any np⁶ configuration and the reader is left to confirm this statement.

Lithium (Z = 3) and beryllium (Z = 4)

Atomic lithium has the electronic configuration 1s² 2s¹, and its term symbol is the same as that for hydrogen, ²S_1/2.

Similarly, the term symbol for beryllium (1s²2s²) is the same as that for helium, ¹S₀.

Boron (Z = 5)

   For boron (1s²2s²2p¹) we need only consider the p electron for reasons outlined above. For this, l = 1 so L = 1 (a P term); S = 1/2 and so (2S ‏+1( = 2 (a doublet term). J can take values) L ‏ S); (L ‏+ S ^_ 1) . . . |L ^_ S|, and so J = 3/2 or 1/2. The term symbol for boron may be ²P_3/2 or ²P_1/2.

Carbon (Z = 6)

  For carbon (1s²2s²2p²), only the p electrons need be considered, and each has l = 1. Values of ml may be ‏+1, 0 or ^_1, and the algebraic sum of ml for the individual electrons gives values of L = 2, 1 or 0 (D, P or S terms respectively).  The two electrons may be spin-paired or have parallel spins and so S = 0 or 1, giving (2S ‏+ 1) = 1 (singlet term) or 3 (triplet term). It might seem that J could be 3, 2, 1 or 0, but this is not so. If, for example, the two electrons each have n = 2, l = 1 and m_l = 1 (giving L = 2), they cannot both have m_s = ‏1/2 as this would violate Pauli’s principle.  The only allowed combinations of ml and ms (and corresponding values of M_L and MS) for two p electrons with the same value of n are shown in the table; such combinations are called microstates. Table: Microstates for two electrons in an np level: values of ml and m_s (represented as paired or unpaired electrons) and resultant values of M_L , M_S and M_J .

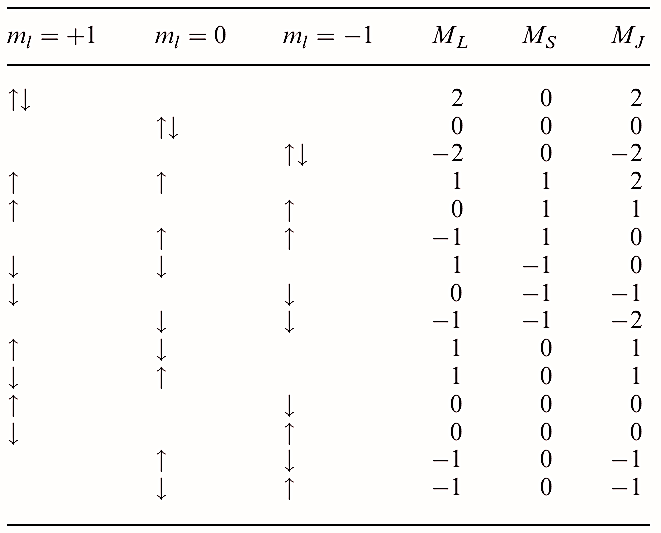
  Inspection of the table reveals the following:

• the 15 microstates can be grouped into three sets, with the proviso that no set can contain a repetition;
• there is a set of five microstates with M_L = 2, 1, 0, ^_1, ^_2 and M_S = 0 (and thus M_J = 2, 1, 0, ^_1, ^_2) corresponding to L = 1 and a D term; moreover, since S = 0 (singlet) and J = 2, the term symbol is ¹D₂;
• there is a set of nine microstates with M_L = 1, 0, ^_1 and M_S = 1, 0, ^_1 which can be assigned the term ³P (because L = 1 and S = 1);
• further examination of this last set of microstates reveals that it can be subdivided into a set of five with

J = 2 (term symbol 3P2), a set of three with J = 1 (³P₁), and a single entry with J = 0 (³P₀);

• one entry in the table remains unaccounted for and has M_L = 0, M_S = 0 and M_J = 0, corresponding to the term ¹S₀.

    We have, of course, no means of telling which entry with M_L = 0 and M_S = 0 should be assigned to which term (or similarly, how entries with M_L = 1 and M_S = 0, or M_L = ^_1 and M_S = 0 should be assigned). Indeed, it is not meaningful to do so.   Of the five terms that we have denoted for carbon (¹D₂, ³P₂, ³P₁, ³P₀ and ¹S₀), the one with the lowest energy is ³P₀ and this is the electronic ground state. The others are excited states; notice that Hund’s rules do not always apply to excited states.

Nitrogen to neon (Z = 7^_10)

   A similar treatment for the nitrogen atom shows that the 2p³ configuration gives rise to 4S, 2P and 2D terms. For the 2p⁴ configuration (oxygen), we introduce a useful simplification by considering it as 2p⁶ plus two positrons which annihilate two of the electrons. Since positrons differ from electrons only in charge, the terms arising from the np⁴ and np² configurations are the same. Similarly, np⁵ is equivalent to np1. This positron or positive hole concept is very useful and can be extended to nd configurations.  Relative energies of terms and levels.  In regard to relative energies of terms, we state all of Hund’s rules in a formal way. It is found from analysis of spectroscopic data that, provided that Russell–Saunders coupling holds:

• the term having the highest spin multiplicity (highest value of S) is the most stable (lowest energy);
• if two or more terms have the same value of S, the term having the higher value of L is the more stable;
• for all terms having the same values of S and L, the level with the lowest value of J is the most stable if the sub-shell is less than half-filled, and the level with the highest value of J is most stable if the sub-shell is more than half-filled (if the sub-shell is half-filled and S has the highest possible value, L = 0 and J = S).

   Thus for the terms corresponding to the electronic configuration np2 (¹D₂, ³P₂, ³P₁, ³P₀ and ¹S₀, see above), that of lowest energy is ³P and the level of lowest energy is ³P₀.