
تاريخ الفيزياء

علماء الفيزياء


الفيزياء الكلاسيكية

الميكانيك

الديناميكا الحرارية


الكهربائية والمغناطيسية

الكهربائية

المغناطيسية

الكهرومغناطيسية


علم البصريات

تاريخ علم البصريات

الضوء

مواضيع عامة في علم البصريات

الصوت


الفيزياء الحديثة


النظرية النسبية

النظرية النسبية الخاصة

النظرية النسبية العامة

مواضيع عامة في النظرية النسبية

ميكانيكا الكم

الفيزياء الذرية

الفيزياء الجزيئية


الفيزياء النووية

مواضيع عامة في الفيزياء النووية

النشاط الاشعاعي


فيزياء الحالة الصلبة

الموصلات

أشباه الموصلات

العوازل

مواضيع عامة في الفيزياء الصلبة

فيزياء الجوامد


الليزر

أنواع الليزر

بعض تطبيقات الليزر

مواضيع عامة في الليزر


علم الفلك

تاريخ وعلماء علم الفلك

الثقوب السوداء


المجموعة الشمسية

الشمس

كوكب عطارد

كوكب الزهرة

كوكب الأرض

كوكب المريخ

كوكب المشتري

كوكب زحل

كوكب أورانوس

كوكب نبتون

كوكب بلوتو

القمر

كواكب ومواضيع اخرى

مواضيع عامة في علم الفلك

النجوم

البلازما

الألكترونيات

خواص المادة


الطاقة البديلة

الطاقة الشمسية

مواضيع عامة في الطاقة البديلة

المد والجزر

فيزياء الجسيمات


الفيزياء والعلوم الأخرى

الفيزياء الكيميائية

الفيزياء الرياضية

الفيزياء الحيوية

الفيزياء العامة


مواضيع عامة في الفيزياء

تجارب فيزيائية

مصطلحات وتعاريف فيزيائية

وحدات القياس الفيزيائية

طرائف الفيزياء

مواضيع اخرى
Coaxial Cable and Poynting Vector
المؤلف:
Sidney B. Cahn Boris E. Nadgorny
المصدر:
A GUIDE TO PHYSICS PROBLEMS
الجزء والصفحة:
part 1 , p 64
8-8-2016
2469
Coaxial Cable and Poynting Vector
The infinitely long coaxial line in Figure 1.1 carries a steady current I upwards in the inner conductor and a return current I downwards in the outer conductor. Both conductors have a resistance per length (along the axes) λ. The space between the inner and outer conductors is occupied by
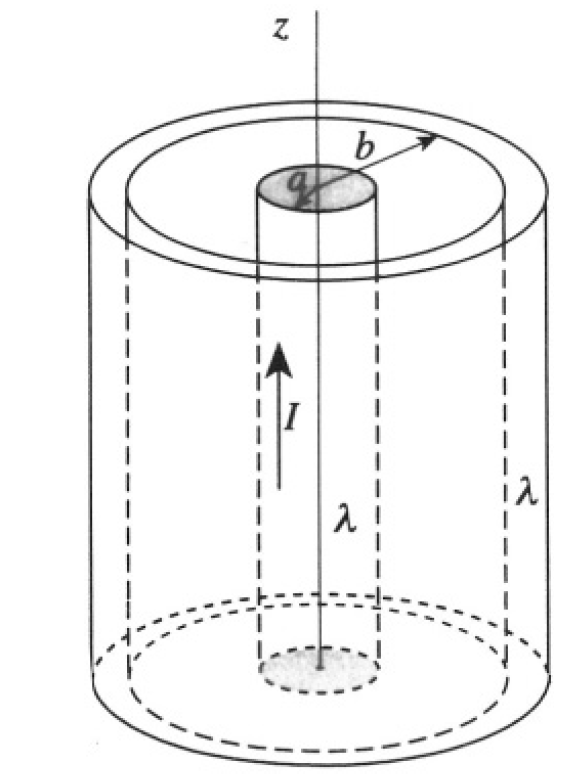
Figure 1.1
a vacuum. The radius of the inner conductor is a, and that of the outer conductor is b. In the following, use the cylindrical coordinates, ρ, φ, z. In these coordinates,

a) Find the electrostatic potential and the electric field in the region a < ρ < b. Assume that Eρ (ρ, φ, 0) = 0.
b) Find the magnetic field in the region a < ρ < b.
c) Calculate the Poynting vector in the region a < ρ < b and integrate it over the surface of the volume bounded by ρ = a, ρ = b, and –l/2 ≤ z ≤ l/2. Comment on the physical implications of your result.
SOLUTION
We have Laplace’s equation in cylindrical coordinates whose solution is
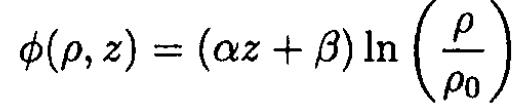 (1)
(1)
From the boundary conditions,
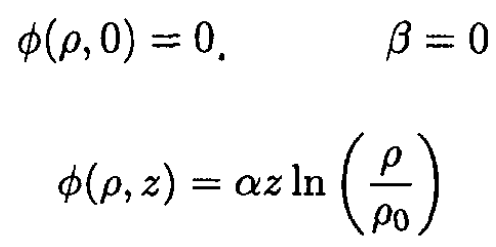 (2)
(2)

Figure 1.2
Integrating the voltage drop along the cable (see Figure 1.2), we find
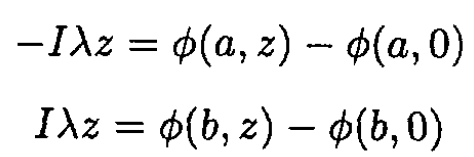 (3)
(3)
and so
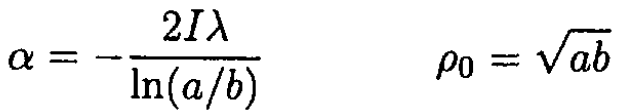
resulting in
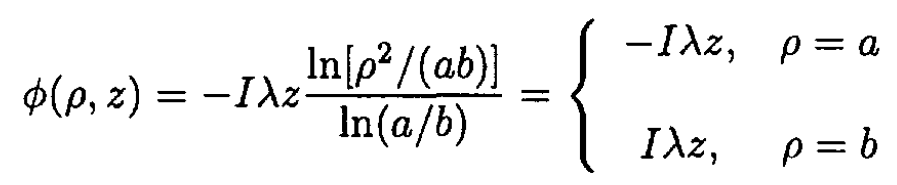 (4)
(4)
The electric field
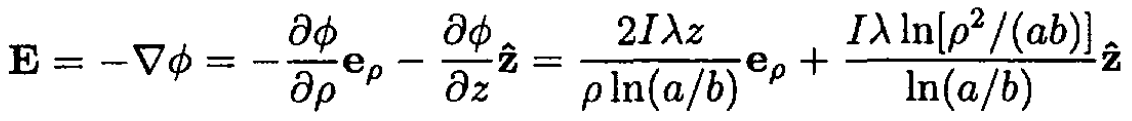 (5)
(5)
b) The magnetic field in the region a < ρ < b can be found from
 (6)
(6)
c) The Poynting vector is
 (7)
(7)
Transforming E into Cartesian coordinates, we have
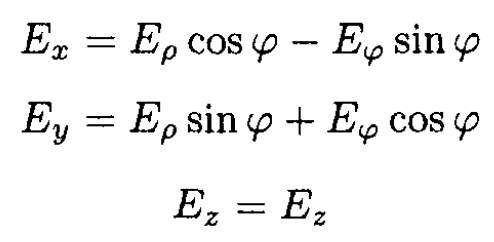 (8)
(8)
The same transformation applies to H, so we obtain
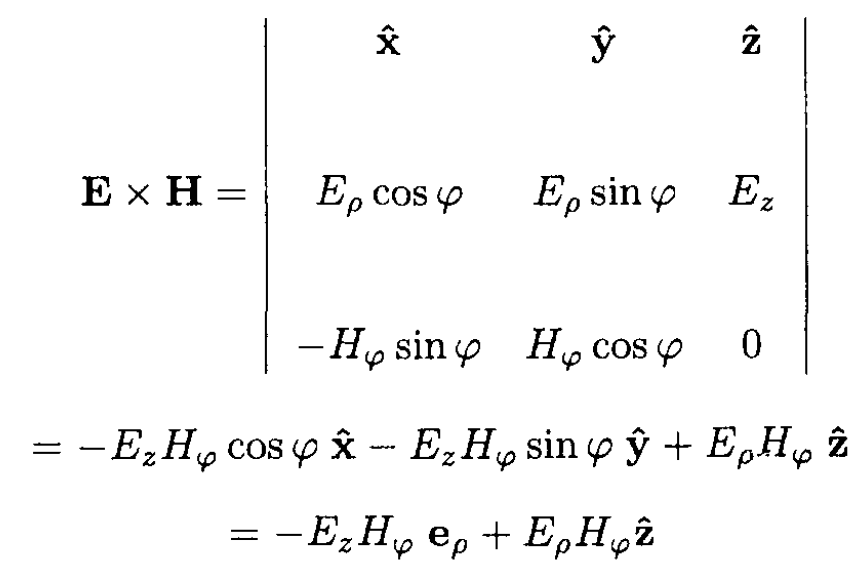 (9)
(9)
So
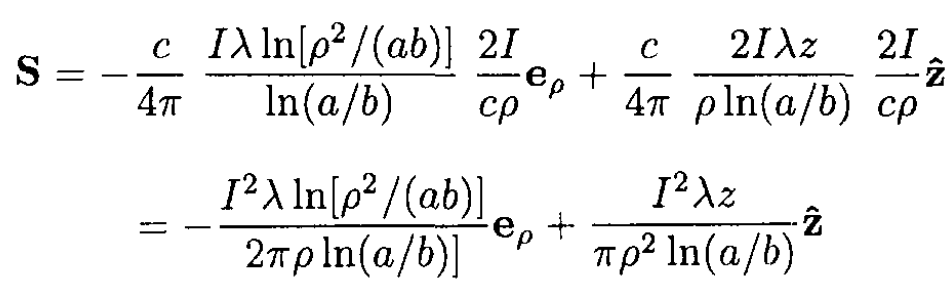 (10)
(10)
We now write the flux Fi and Fo into the inner and outer conductors, respectively, from (10):
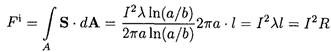 (11)
(11)
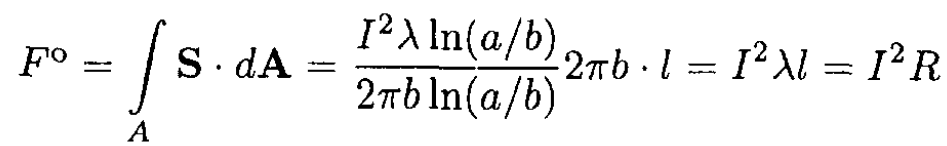 (12)
(12)
where R is the resistance of a length of each conductor. The total flux going into the conductors F = Fi + Fo = 2I2R, which corresponds to the Joule heating of the conductors. Since there is no current in the vacuum between the conductors and the conditions are stationary, Poynting’s theorem gives
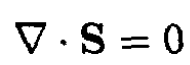 (13)
(13)
The total flux is zero. There must also be a corresponding negative flux Fe into the volume through the ends to satisfy Poynting’s theorem. Indeed
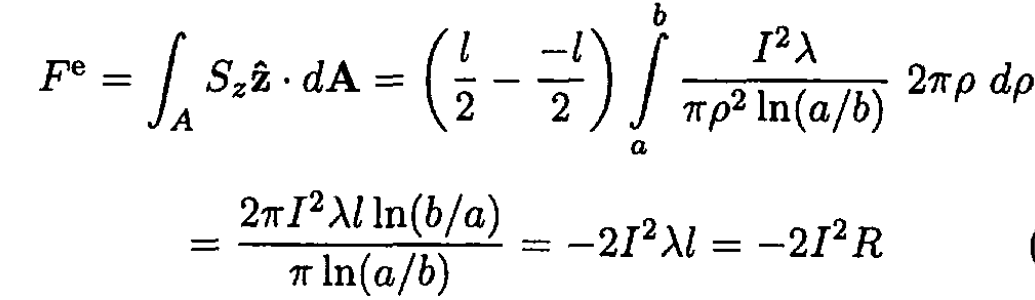 (14)
(14)
as expected.
 الاكثر قراءة في مواضيع اخرى
الاكثر قراءة في مواضيع اخرى
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة

الآخبار الصحية















 "المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة
"المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة (نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام) قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى)
قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى)


















