


 تاريخ الرياضيات
تاريخ الرياضيات
 الرياضيات في الحضارات المختلفة
الرياضيات في الحضارات المختلفة 
 الرياضيات المتقطعة
الرياضيات المتقطعة
 الجبر
الجبر
 الهندسة
الهندسة 
 المعادلات التفاضلية و التكاملية
المعادلات التفاضلية و التكاملية 
 التحليل
التحليل
 علماء الرياضيات
علماء الرياضيات |
Read More
Date: 17-6-2021
Date: 12-5-2021
Date: 8-6-2021
|
A connection on a vector bundle 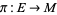 is a way to "differentiate" bundle sections, in a way that is analogous to the exterior derivative
is a way to "differentiate" bundle sections, in a way that is analogous to the exterior derivative 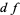 of a function
of a function 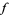 . In particular, a connection
. In particular, a connection 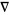 is a function from smooth sections
is a function from smooth sections 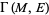 to smooth sections of
to smooth sections of 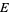 with one-forms
with one-forms 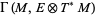 that satisfies the following conditions.
that satisfies the following conditions.
1. 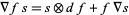 (Leibniz rule), and
(Leibniz rule), and
2. 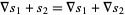 .
.
Alternatively, a connection can be considered as a linear map from bundle sections of 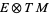 , i.e., a section of
, i.e., a section of 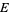 with a vector field
with a vector field 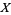 , to sections of
, to sections of 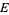 , in analogy to the directional derivative. The directional derivative of a function
, in analogy to the directional derivative. The directional derivative of a function 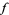 , in the direction of a vector field
, in the direction of a vector field 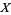 , is given by
, is given by 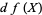 . The connection, along with a vector field
. The connection, along with a vector field 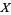 , may be applied to a section
, may be applied to a section 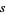 of
of 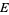 to get the section
to get the section 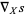 . From this perspective, connections must also satisfy
. From this perspective, connections must also satisfy
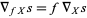 |
(1) |
for any smooth function 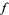 . This property follows from the first definition.
. This property follows from the first definition.
For example, the trivial bundle 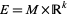 admits a flat connection since any bundle section
admits a flat connection since any bundle section  corresponds to a function
corresponds to a function 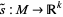 . Then setting
. Then setting 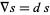 gives the connection. Any connection on the trivial bundle is of the form
gives the connection. Any connection on the trivial bundle is of the form 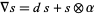 , where
, where 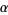 is any one-form with values in
is any one-form with values in  , i.e.,
, i.e., 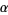 is a matrix of one-forms.
is a matrix of one-forms.
The matrix of one-forms
![alpha=[dx 2xdy 0; 0 dx-3dy 0; xydx 0 y^2dx+dy]](https://mathworld.wolfram.com/images/equations/VectorBundleConnection/NumberedEquation2.gif) |
(2) |
determines a connection 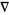 on the rank-3 bundle over
on the rank-3 bundle over 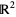 . It acts on a section
. It acts on a section  by the following.
by the following.
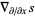 |
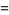 |
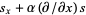 |
(3) |
 |
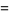 |
![s_x+[1 0 0; 0 1 0; xy 0 y^2]s](https://mathworld.wolfram.com/images/equations/VectorBundleConnection/Inline38.gif) |
(4) |
 |
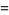 |
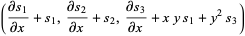 |
(5) |
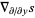 |
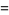 |
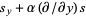 |
(6) |
 |
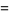 |
![s_y+[0 2x 0; 0 -3 0; 0 0 1]s](https://mathworld.wolfram.com/images/equations/VectorBundleConnection/Inline47.gif) |
(7) |
 |
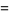 |
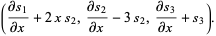 |
(8) |
In any trivialization, a connection can be described just as in the case of a trivial bundle. However, if the bundle 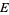 is not trivial, then the exterior derivative
is not trivial, then the exterior derivative  is not well-defined (globally) for a bundle section
is not well-defined (globally) for a bundle section  . Still, the difference between any two connections must be one-forms with values in endomorphisms of
. Still, the difference between any two connections must be one-forms with values in endomorphisms of 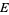 ,i.e., matrices of one forms. So the space of connections forms an affine space.
,i.e., matrices of one forms. So the space of connections forms an affine space.
The bundle curvature of the bundle is given by the formula 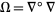 . In coordinates,
. In coordinates,  is matrix of two-forms. For instance, in the example above,
is matrix of two-forms. For instance, in the example above,
![Omega=[0 2xdx ^ dy 0; 0 -3dx ^ dy 0; 0 2x^2ydx ^ dy y^2dx ^ dy]](https://mathworld.wolfram.com/images/equations/VectorBundleConnection/NumberedEquation3.gif) |
(9) |
is the curvature.
Another way of describing a connection is as a splitting of the tangent bundle 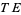 of
of  as
as 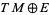 . The vertical part of
. The vertical part of 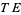 corresponds to tangent vectors along the fibers, and is the kernel of
corresponds to tangent vectors along the fibers, and is the kernel of 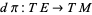 . The horizontal part is not well-defined a priori. A connection defines a subspace of
. The horizontal part is not well-defined a priori. A connection defines a subspace of 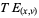 which is isomorphic to
which is isomorphic to 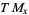 . It defines
. It defines 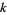 flat sections
flat sections 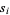 such that
such that 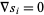 , which are a vector basis for the fiber bundles of
, which are a vector basis for the fiber bundles of  , at least nearby
, at least nearby  . These flat sections determine the horizontal part of
. These flat sections determine the horizontal part of  near
near  . Also, a connection on a vector bundle can be defined by a principal bundle connection on the associated principal bundle.
. Also, a connection on a vector bundle can be defined by a principal bundle connection on the associated principal bundle.
In some settings there is a canonical connection. For example, a Riemannian manifold has the Levi-Civita connection, given by the Christoffel symbols of the first and second kinds, which is the unique torsion-free connection compatible with the metric. A holomorphic vector bundle with a Hermitian metric has a unique connection which is compatible with both metric and the complex structure.



|
|
|
|
"عادة ليلية" قد تكون المفتاح للوقاية من الخرف
|
|
|
|
|
|
|
ممتص الصدمات: طريقة عمله وأهميته وأبرز علامات تلفه
|
|
|
|
|
|
|
المجمع العلمي للقرآن الكريم يقيم جلسة حوارية لطلبة جامعة الكوفة
|
|
|