


 تاريخ الرياضيات
تاريخ الرياضيات
 الرياضيات في الحضارات المختلفة
الرياضيات في الحضارات المختلفة 
 الرياضيات المتقطعة
الرياضيات المتقطعة
 الجبر
الجبر
 الهندسة
الهندسة 
 المعادلات التفاضلية و التكاملية
المعادلات التفاضلية و التكاملية 
 التحليل
التحليل
 علماء الرياضيات
علماء الرياضيات |
Read More
Date: 23-10-2016
Date: 30-10-2016
Date: 30-10-2016
|
Died: 3 May 1885 in Dorpat, Russia (now Tartu, Estonia)
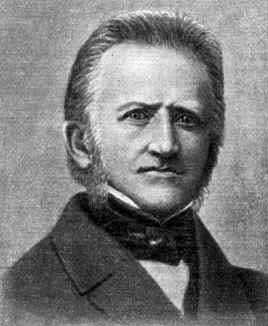
Ferdinand Minding's family moved from Kalisz to Hirschberg in Prussian Silesia when he was one year old. Hirschberg is now Jelenia Góra in Poland. He attended Hirschberg Gymnasium graduating in 1824. From there he went to the universities of Halle and Berlin where he studied philology, philosophy and physics. He graduated from Berlin in 1827, then taught mathematics in schools for several years.
Minding had not studied mathematics at university, so how did he become a mathematics teacher? He was self taught in mathematics having studied the subject on his own while pursuing other topics at university. While he was a school teacher, he studied for his doctorate which was awarded by Halle for a thesis on approximating the values of double integrals.
In 1831 Minding became a mathematics lecturer at the University of Berlin then, in 1843 he was to become professor at Dorpat, a post he held for 40 years. At Dorpat Minding taught algebra, analysis, geometry, probability, mechanics and physics. From 1851 until 1855 he was Dean of the Faculty at Dorpat.
In 1864 Minding became a Russian citizen and, in the same year, was elected to the St Petersburg Academy.
His work, which continued Gauss's study of 1828 on the differential geometry of surfaces, greatly influenced Peterson. In 1830 Minding published on the problem of the shortest closed curve on a given surface enclosing a given area. He introduced the geodesic curvature although he did not use the term which was due to Bonnet who discovered it independently in 1848. In fact Gauss had proved these results, before either Minding of Bonnet, in 1825 but he had not published them.
Minding also studied the bending of surfaces proving what is today called Minding's theorem in 1839. The following year he published in Crelle's Journal a paper giving results about trigonometric formulae on surfaces of constant curvature. Lobachevsky had published, also in Crelle's Journal, related results three years earlier and these results by Lobachevsky and Minding formed the basis of Beltrami's interpretation of hyperbolic geometry in 1868.
Minding also worked on differential equations, algebraic functions, continued fractions and analytic mechanics. In differential equations he used integrating factor methods. This work won Minding the Demidov prize of the St Petersburg Academy in 1861. It was further developed by A N Korkin. Darboux and Émile Picard pushed these results still further in 1878.
Books:
Articles:



|
|
|
|
علامات بسيطة في جسدك قد تنذر بمرض "قاتل"
|
|
|
|
|
|
|
أول صور ثلاثية الأبعاد للغدة الزعترية البشرية
|
|
|
|
|
|
|
مكتبة أمّ البنين النسويّة تصدر العدد 212 من مجلّة رياض الزهراء (عليها السلام)
|
|
|