
Cunningham Number
 المؤلف:
Mudge, M.
المؤلف:
Mudge, M.
 المصدر:
"Not Numerology but Numeralogy!" Personal Computer World
المصدر:
"Not Numerology but Numeralogy!" Personal Computer World
 الجزء والصفحة:
...
الجزء والصفحة:
...
 1-1-2021
1-1-2021
 1196
1196
Cunningham Number
A Cunningham number is a binomial number of the form 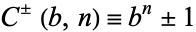 with
with 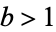 and
and 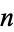 positive integers. Bases
positive integers. Bases 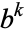 which are themselves powers need not be considered since they correspond to
which are themselves powers need not be considered since they correspond to 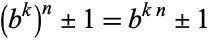 . Prime numbers of the form
. Prime numbers of the form 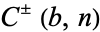 are very rare.
are very rare.
A necessary (but not sufficient) condition for 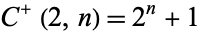 to be prime is that
to be prime is that 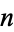 be of the form
be of the form 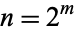 . Numbers of the form
. Numbers of the form 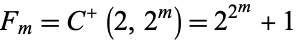 are called Fermat numbers, and the only known primes occur for
are called Fermat numbers, and the only known primes occur for 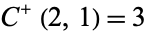 ,
, 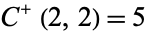 ,
, 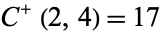 ,
, 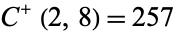 , and
, and 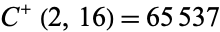 (i.e.,
(i.e., 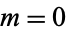 , 1, 2, 3, 4). The only other primes
, 1, 2, 3, 4). The only other primes 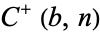 for nontrivial
for nontrivial  and
and  are
are  ,
,  , and
, and  .
.
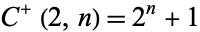 is always divisible by 3 when
is always divisible by 3 when 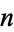 is odd.
is odd.
Primes of the form  are also very rare. The Mersenne numbers
are also very rare. The Mersenne numbers 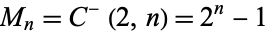 are known to be prime only for 44 values, the first few of which are
are known to be prime only for 44 values, the first few of which are 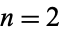 , 3, 5, 7, 13, 17, 19, ... (OEIS A000043). Such numbers are known as Mersenne primes. There are no other primes
, 3, 5, 7, 13, 17, 19, ... (OEIS A000043). Such numbers are known as Mersenne primes. There are no other primes 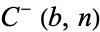 for nontrivial
for nontrivial 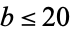 and
and  .
.
In 1925, Cunningham and Woodall (1925) gathered together all that was known about the primality and factorization of the numbers  and published a small book of tables. These tables collected from scattered sources the known prime factors for the bases 2 and 10 and also presented the authors' results of 30 years' work with these and other bases.
and published a small book of tables. These tables collected from scattered sources the known prime factors for the bases 2 and 10 and also presented the authors' results of 30 years' work with these and other bases.
Since 1925, many people have worked on filling in these tables. D. H. Lehmer, a well-known mathematician who died in 1991, was for many years a leader of these efforts. Lehmer was a mathematician who was at the forefront of computing as modern electronic computers became a reality. He was also known as the inventor of some ingenious pre-electronic computing devices specifically designed for factoring numbers.
Updated factorizations were published in Brillhart et al. (1988). The tables have been extended by Brent and te Riele (1992) to  , ..., 100 with
, ..., 100 with 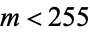 for
for 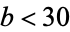 and
and 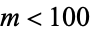 for
for 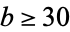 . All numbers with exponent 58 and smaller, and all composites with
. All numbers with exponent 58 and smaller, and all composites with  digits have now been factored.
digits have now been factored.
REFERENCES:
Brent, R. P. and te Riele, H. J. J. "Factorizations of 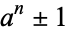 ,
, 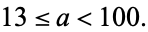 " Report NM-R9212, Centrum voor Wiskunde en Informatica. Amsterdam, June 1992. https://web.comlab.ox.ac.uk/oucl/work/richard.brent/pub/pub200.html.
" Report NM-R9212, Centrum voor Wiskunde en Informatica. Amsterdam, June 1992. https://web.comlab.ox.ac.uk/oucl/work/richard.brent/pub/pub200.html.
Brillhart, J.; Lehmer, D. H.; Selfridge, J.; Tuckerman, B.; and Wagstaff, S. S. Jr. Factorizations of b-n+/-1, b=2, 3, 5, 6, 7, 10, 11, 12 Up to High Powers, 3rd ed. Providence, RI: Amer. Math. Soc., 1988. https://www.ams.org/online_bks/conm22/.
Cunningham, A. J. C. and Woodall, H. J. Factorisation of y-n∓1, y=2, 3, 5, 6, 7, 10, 11, 12 Up to High Powers (n). London: Hodgson, 1925.
Mudge, M. "Not Numerology but Numeralogy!" Personal Computer World, 279-280, 1997.
Ribenboim, P. "Numbers 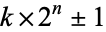 ." §5.7 in The New Book of Prime Number Records. New York: Springer-Verlag, pp. 355-360, 1996.
." §5.7 in The New Book of Prime Number Records. New York: Springer-Verlag, pp. 355-360, 1996.
Sloane, N. J. A. Sequence A000043/M0672 in "The On-Line Encyclopedia of Integer Sequences."
Wagstaff, S. S. Jr. "The Cunningham Project." https://www.cerias.purdue.edu/homes/ssw/cun/.
Wagstaff, S. S. Jr. "The Third Edition of the Cunningham Books." https://www.cerias.purdue.edu/homes/ssw/cun/third/.a
 الاكثر قراءة في نظرية الاعداد
الاكثر قراءة في نظرية الاعداد
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة


