A figurate number of the form

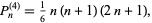(1)

corresponding to a configuration of points which form a square pyramid, is called a square pyramidal number (or sometimes, simply a pyramidal number). The first few are 1, 5, 14, 30, 55, 91, 140, 204, ... (OEIS A000330). The generating function for square pyramidal numbers is

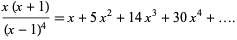(2)

The square pyramidal numbers are sums of consecutive pairs of tetrahedral numbers and satisfy

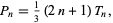(3)

where 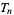 is the th triangular number.

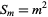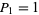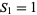The only numbers which are simultaneously square  and square pyramidal  (the cannonball problem) are  and , corresponding to  and 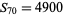 (Ball and Coxeter 1987, p. 59; Ogilvy 1988; Dickson 2005, p. 25), as conjectured by Lucas (1875), partially proved by Moret-Blanc (1876) and Lucas (1877), and proved by Watson (1918). The problem requires solving the Diophantine equation

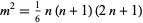(4)

(Guy 1994, p. 147). Watson (1918) gave an almost elementary proof, disposing of most cases by elementary means, but resorting to the use of elliptic functions for one pesky case. Entirely elementary proofs have been given by Ma (1985) and Anglin (1990).

Numbers which are simultaneously triangular 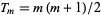 and square pyramidal  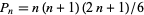satisfy the Diophantine equation

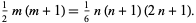(5)

Completing the square gives

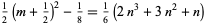(6)

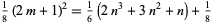(7)

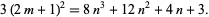(8)

The only solutions are , (0, 0), (1, 1), (5, 10), (6, 13), and (85, 645) (Guy 1994, p. 147), corresponding to the nontrivial triangular square pyramidal numbers 1, 55, 91, 208335.

Numbers which are simultaneously tetrahedral 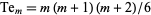 and square pyramidal  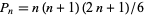satisfy the Diophantine equation

(9)

Beukers (1988) has studied the problem of finding solutions via integral points on an elliptic curve and found that the only solution is the trivial .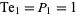

REFERENCES:

Anglin, W. S. "The Square Pyramid Puzzle." Amer. Math. Monthly 97, 120-124, 1990.

Anglin, W. S. The Queen of Mathematics: An Introduction to Number Theory. Dordrecht, Netherlands: Kluwer, 1995.

Baker, A. and Davenport, H. "The Equations 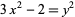 and 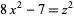." Quart J. Math. Ser. 2 20, 129-137, 1969.

Ball, W. W. R. and Coxeter, H. S. M. Mathematical Recreations and Essays, 13th ed. New York: Dover, p. 59, 1987.

Beukers, F. "On Oranges and Integral Points on Certain Plane Cubic Curves." Nieuw Arch. Wisk. 6, 203-210, 1988.

Conway, J. H. and Guy, R. K. The Book of Numbers. New York: Springer-Verlag, pp. 47-50, 1996.

Dickson, L. E. History of the Theory of Numbers, Vol. 2: Diophantine Analysis. New York: Dover, 2005.

Guy, R. K. "Figurate Numbers." §D3 in Unsolved Problems in Number Theory, 2nd ed. New York: Springer-Verlag, pp. 147-150, 1994.

Kanagasabapathy, P. and Ponnudurai, T. "The Simultaneous Diophantine Equations 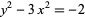 and ." Quart. J. Math. Ser. 2 26, 275-278, 1975.

Ljunggren, W. "New Solution of a Problem Posed by E. Lucas." Nordisk Mat. Tidskrift 34, 65-72, 1952.

Lucas, É. Question 1180. Nouv. Ann. Math. Ser. 2 14, 336, 1875.

Lucas, É. Solution de Question 1180. Nouv. Ann. Math. Ser. 2 15, 429-432, 1877.

Ma, D. G. "An Elementary Proof of the Solution to the Diophantine Equation 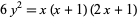." Sichuan Daxue Xuebao 4, 107-116, 1985.

Moret-Blanc, M. Question 1180. Nouv. Ann. Math. Ser. 2 15, 46-48, 1876.

Ogilvy, C. S. and Anderson, J. T. Excursions in Number Theory. New York: Dover, pp. 77 and 152, 1988.

Sloane, N. J. A. Sequence A000330/M3844 in "The On-Line Encyclopedia of Integer Sequences."

Watson, G. N. "The Problem of the Square Pyramid." Messenger. Math. 48, 1-22, 1918.

Wolf, T. "The 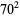 Puzzle." https://home.tiscalinet.ch/t_wolf/tw/misc/squares.html.

اخر الاخبار

اخبار العتبة العباسية المقدسة

قسم صناعة الشبابيك يشرع بإدامة شباكين قديمين لمرقد الإمامين العسكريين (عليهما السلام)

فرقة العباس (عليه السلام) تختتم خدماتها المقدمة للزائرين في سامراء

موكب العتبتين المقدستين يحيي ذكرى استشهاد الإمام الهادي (عليه السلام) في سامراء

العتبة العسكرية المقدسة تكرّم وفد العتبة العباسية المقدسة لمشاركته في تقديم الخدمات للزائرين

المزيد

الآخبار الصحية

كنز غذائي على طبقك.. ماذا تفعل السبانخ بجسمك؟

ما لا يتوقعه أحد.. "سر غذائي" في الجبن الدسم

لتحسين الهضم والنوم.. هذا أفضل وقت لتناول العشاء

10 طرق بسيطة وغير مألوفة لحرق المزيد من السعرات يوميا

المزيد

الآخبار التكنلوجية

سابقة بعالم الطيران.. طائرة تقرر الهبوط بنفسها بعد ظرف طارئ

"الإنفلونزا الخارقة" تكتسح دول العالم.. وتثير قلقا كبيرا

مرحلة ما قبل الأعراض.. دواء جديد يستهدف "شرارة" الزهايمر

إزالة ثاني أكسيد الكربون من الغلاف الجوي.. ما دور المحيطات والبحار؟

المزيد