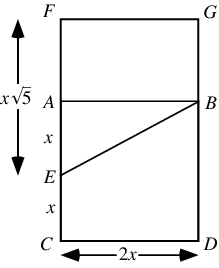
Given a rectangle having sides in the ratio 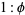, the golden ratio  is defined such that partitioning the original rectangle into a square and new rectangle results in a new rectangle having sides with a ratio . Such a rectangle is called a golden rectangle. Euclid used the following construction to construct them. Draw the square , call 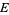 the midpoint of 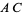, so that 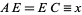. Now draw the segment 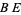, which has length

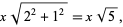(1)

and construct 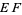 with this length. Now complete the rectangle 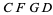, which is golden since

(2)

Successive points dividing a golden rectangle into squares lie on a logarithmic spiral (Wells 1991, p. 39; Livio 2002, p. 119) which is sometimes known as the golden spiral.

The spiral is not actually tangent at these points, however, but passes through them and intersects the adjacent side, as illustrated above.

If the top left corner of the original square is positioned at (0, 0), the center of the spiral occurs at the position

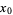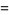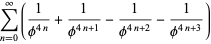			(3)
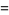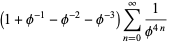			(4)
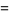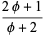			(5)
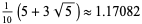			(6)
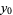			(7)
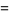			(8)
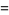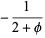			(9)
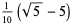			(10)
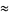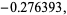			(11)

and the parameters of the spiral 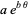 are given by

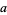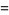			(12)
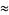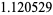			(13)
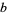			(14)
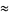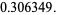			(15)

REFERENCES:

Bicknell, M.; and Hoggatt, V. E. Jr. "Golden Triangles, Rectangles, and Cuboids." Fib. Quart. 7, 73-91, 1969.

Cook, T. A. The Curves of Life, Being an Account of Spiral Formations and Their Application to Growth in Nature, To Science and to Art. New York: Dover, 1979.

Cundy, H. and Rollett, A. Mathematical Models, 3rd ed. Stradbroke, England: Tarquin Pub., p. 70, 1989.

Kabai, S. Mathematical Graphics I: Lessons in Computer Graphics Using Mathematica. Püspökladány, Hungary: Uniconstant, p. 79, 2002.

Livio, M. The Golden Ratio: The Story of Phi, the World's Most Astonishing Number. New York: Broadway Books, p. 85, 2002.

Pappas, T. "The Golden Rectangle." The Joy of Mathematics. San Carlos, CA: Wide World Publ./Tetra, pp. 102-106, 1989.

Steinhaus, H. Mathematical Snapshots, 3rd ed. New York: Dover, pp. 45-47, 1999.

Wells, D. The Penguin Dictionary of Curious and Interesting Geometry. London: Penguin, p. 88, 1991.

Williams, R. The Geometrical Foundation of Natural Structure: A Source Book of Design. New York: Dover, p. 53, 1979.

اخر الاخبار

اخبار العتبة العباسية المقدسة

جمعية كشافة الكفيل: المجالس الحسينية تعلّم الفتية قيم نهضة الإمام الحسين (عليه السلام)

عَبرَ المجالس الحسينية.. المجمَع العلمي يقدّم محاضرات دينية وتلاوات قرآنية في بغداد والنجف وميسان

برعاية العتبة العباسية المقدسة.. موكب قناديل السماء يُحيي مراسم اليوم الثالث عشر من المحرّم

بعد انتهاء مراسم ذكرى دفن الأجساد الطاهرة قسم رعاية الصحن يباشر بحملة تنظيف واسعة لصحن مرقد أبي الفضل العباس (عليه السلام)

المزيد

الآخبار الصحية

اختراق علمي.. دواء يؤخر ظهور السكري من النوع الأول لسنوات

هذا ما يحدث لجسمك إن أهملت نظافة فراشك

لماذا تعتبر النساء أكثر عرضة للإصابة بألزهايمر من الرجال؟

لماذا يحذر الخبراء من الإفراط في تناول الفراولة؟

المزيد

الآخبار التكنلوجية

السيارات الكهربائية أنظف بنسبة 73% من سيارات الوقود

سيارة لوسِد الكهربائية تدخل "غينيس" بأطول مدى في العالم

نظرية جديدة تنسف كل ما نعرفه عن تشكل أول قارة في الأرض

منذ 37 ألف عام.. دراسة تكشف "أقدم أمراض" أصابت البشر

المزيد