

تاريخ الرياضيات

الاعداد و نظريتها

تاريخ التحليل

تار يخ الجبر

الهندسة و التبلوجي


الرياضيات في الحضارات المختلفة

العربية

اليونانية

البابلية

الصينية

المايا

المصرية

الهندية


الرياضيات المتقطعة

المنطق

اسس الرياضيات

فلسفة الرياضيات

مواضيع عامة في المنطق


الجبر

الجبر الخطي

الجبر المجرد

الجبر البولياني

مواضيع عامة في الجبر

الضبابية

نظرية المجموعات

نظرية الزمر

نظرية الحلقات والحقول

نظرية الاعداد

نظرية الفئات

حساب المتجهات

المتتاليات-المتسلسلات

المصفوفات و نظريتها

المثلثات


الهندسة

الهندسة المستوية

الهندسة غير المستوية

مواضيع عامة في الهندسة

التفاضل و التكامل


المعادلات التفاضلية و التكاملية

معادلات تفاضلية

معادلات تكاملية

مواضيع عامة في المعادلات


التحليل

التحليل العددي

التحليل العقدي

التحليل الدالي

مواضيع عامة في التحليل

التحليل الحقيقي

التبلوجيا

نظرية الالعاب

الاحتمالات و الاحصاء

نظرية التحكم

بحوث العمليات

نظرية الكم

الشفرات

الرياضيات التطبيقية

نظريات ومبرهنات


علماء الرياضيات

500AD

500-1499

1000to1499

1500to1599

1600to1649

1650to1699

1700to1749

1750to1779

1780to1799

1800to1819

1820to1829

1830to1839

1840to1849

1850to1859

1860to1864

1865to1869

1870to1874

1875to1879

1880to1884

1885to1889

1890to1894

1895to1899

1900to1904

1905to1909

1910to1914

1915to1919

1920to1924

1925to1929

1930to1939

1940to the present

علماء الرياضيات

الرياضيات في العلوم الاخرى

بحوث و اطاريح جامعية

هل تعلم

طرائق التدريس

الرياضيات العامة

نظرية البيان
Repeating Decimal
المؤلف:
Ball, W. W. R. and Coxeter, H. S. M
المصدر:
Mathematical Recreations and Essays, 13th ed. New York: Dover
الجزء والصفحة:
...
1-12-2019
1281
Repeating Decimal
A repeating decimal, also called a recurring decimal, is a number whose decimal representation eventually becomes periodic (i.e., the same sequence of digits repeats indefinitely). The repeating portion of a decimal expansion is conventionally denoted with a vinculum so, for example,
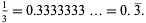 |
The minimum number of digits that repeats in such a number is known as the decimal period.
Repeating decimal notation was implemented in versions of the Wolfram Language prior to 6 as PeriodicForm[RealDigits[r]] after loading the add-on package NumberTheory`ContinuedFractions`.
All rational numbers have either finite decimal expansions (i.e., are regular numbers; e.g.,  ) or repeating decimals (e.g.,
) or repeating decimals (e.g., 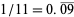 ). However, irrational numbers, such as
). However, irrational numbers, such as 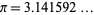 neither terminate nor become periodic.
neither terminate nor become periodic.
Numbers such as 0.5 are sometimes regarded as repeating decimals since 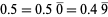 .
.
The denominators of the first few unit fractions having repeating decimals are 3, 6, 7, 9, 11, 12, 13, 14, 15, 17, 18, 19, 21, 22, 23, 24, 26, 27, 28, 29, ... (OEIS A085837).
The repeating portion of a rational number can be found in the Wolfram Language using the command RealDigits[r][[1,-1]]. The number of digits in the repeating portion of the decimal expansion of a rational number can also be found directly from the multiplicative order of its denominator. The periods of the decimal expansions of 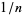 for
for 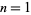 , 2, ... are 0, 0, 1, 0, 0, 1, 6, 0, 1, 0, 2, 1, 6, 6, 1, 0, 16, 1, 18, ... (OEIS A051626), where 0 indicates that the number is regular.
, 2, ... are 0, 0, 1, 0, 0, 1, 6, 0, 1, 0, 2, 1, 6, 6, 1, 0, 16, 1, 18, ... (OEIS A051626), where 0 indicates that the number is regular.
If 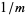 is a repeating decimal and
is a repeating decimal and  is a terminating decimal, them
is a terminating decimal, them 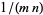 has a nonperiodic part whose length is that of
has a nonperiodic part whose length is that of 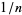 and a repeating part whose length is that of
and a repeating part whose length is that of 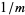 (Wells 1986, p. 60).
(Wells 1986, p. 60).
REFERENCES:
Ball, W. W. R. and Coxeter, H. S. M. Mathematical Recreations and Essays, 13th ed. New York: Dover, pp. 53-54, 1987.
Conway, J. H. and Guy, R. K. The Book of Numbers. New York: Springer-Verlag, pp. 167-168, 1996.
Courant, R. and Robbins, H. "Rational Numbers and Periodic Decimals." §2.2.4 in What Is Mathematics?: An Elementary Approach to Ideas and Methods, 2nd ed. Oxford, England: Oxford University Press, pp. 66-68, 1996.
Sloane, N. J. A. Sequences A051626 and A085837 in "The On-Line Encyclopedia of Integer Sequences."
Wells, D. The Penguin Dictionary of Curious and Interesting Numbers. Middlesex, England: Penguin Books, p. 60, 1986.
 الاكثر قراءة في نظرية الاعداد
الاكثر قراءة في نظرية الاعداد
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة

الآخبار الصحية















 قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة
قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة "المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة
"المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة (نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)


















