


 تاريخ الرياضيات
تاريخ الرياضيات
 الرياضيات في الحضارات المختلفة
الرياضيات في الحضارات المختلفة 
 الرياضيات المتقطعة
الرياضيات المتقطعة
 الجبر
الجبر
 الهندسة
الهندسة 
 المعادلات التفاضلية و التكاملية
المعادلات التفاضلية و التكاملية 
 التحليل
التحليل
 علماء الرياضيات
علماء الرياضيات |
Read More
Date: 14-8-2019
Date: 24-9-2018
Date: 6-8-2019
|
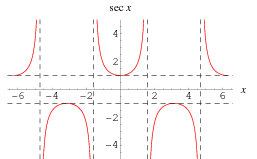
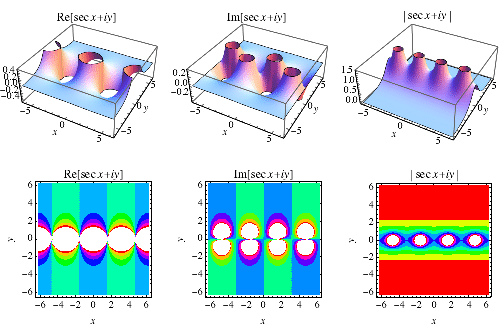 |
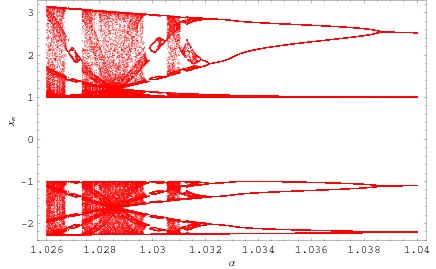
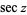 is the trigonometric function defined by
is the trigonometric function defined by
 |
 |
 |
(1) |
 |
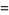 |
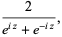 |
(2) |
where 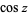 is the cosine. The secant is implemented in the Wolfram Language as Sec[z].
is the cosine. The secant is implemented in the Wolfram Language as Sec[z].
Note that the secant does not appear to be in consistent widespread use in Europe, although it does appear explicitly in various German and Russian handbooks (e.g., Gradshteyn and Ryzhik 2000, p. 43). Interestingly, while 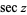 is treated on a par with the other trigonometric functions in some tabulations (Gellert et al. 1989, p. 222), it is not in others (Gradshteyn and Ryzhik 2000, who do not list it in their table of "basic functional relations" on p. 28, but do give identities involving it on p. 43).
is treated on a par with the other trigonometric functions in some tabulations (Gellert et al. 1989, p. 222), it is not in others (Gradshteyn and Ryzhik 2000, who do not list it in their table of "basic functional relations" on p. 28, but do give identities involving it on p. 43).
Tropfke states, "The history of the secant function begins almost contemporaneously with that of the tangent, but ended after discovery of logarithmic calculation in the first half of the 17th century" (Tropfke 1923, pp. 28) and, "The secant naturally disappeared again from trigonometry when, after the introduction of logarithms, the appearance of trigonometric functions in the denominator no longer posed any difficulty" (Tropfke 1923, pp. 30). Harris and Stocker (1998, p. 300) call secant and cosecant "rarely used functions," but then devote an entire section to them. Because these functions do seem to be in widespread use in the United States (e.g., Abramowitz and Stegun 1972, p. 72), reports of their demise seem to be a bit premature.
The derivative is
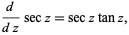 |
(3) |
and the indefinite integral is
![intseczdz=ln[cos(1/2z)+sin(1/2z)]-ln[cos(1/2z)-sin(1/2z)]+C,](http://mathworld.wolfram.com/images/equations/Secant/NumberedEquation2.gif) |
(4) |
where 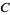 is a constant of integration. For
is a constant of integration. For 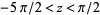 , this can be written
, this can be written
 |
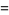 |
![ln[tan(1/4pi+1/2z)]+C](http://mathworld.wolfram.com/images/equations/Secant/Inline14.gif) |
(5) |
 |
 |
 |
(6) |
The Maclaurin series of the secant is
 |
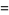 |
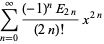 |
(7) |
 |
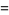 |
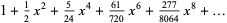 |
(8) |
(OEIS A046976 and A046977), where 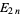 is an Euler number. The first few reduced numerators that are prime are 5, 61, 277, 23489580527043108252017828576198947741, ... (OEIS A092838), corresponding to
is an Euler number. The first few reduced numerators that are prime are 5, 61, 277, 23489580527043108252017828576198947741, ... (OEIS A092838), corresponding to 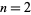 , 3, 4, 19, 24, ... (OEIS A092837).
, 3, 4, 19, 24, ... (OEIS A092837).
A bifurcation plot of  is illustrated above (Trott 2004, p. 169). Of all the trigonometric functions,
is illustrated above (Trott 2004, p. 169). Of all the trigonometric functions, 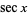 is apparently the only one displaying interesting bifurcation structure for iterates of this form.
is apparently the only one displaying interesting bifurcation structure for iterates of this form.
The positive integer values of  giving incrementally largest values of
giving incrementally largest values of  are given by 1, 2, 5, 8, 11, 344, 699, 1054, 1409, 1764, 2119, ... (OEIS A004112), corresponding to the values 1.85082, 2.403, 3.52532, 6.87285, 225.953, 227.503, ....
are given by 1, 2, 5, 8, 11, 344, 699, 1054, 1409, 1764, 2119, ... (OEIS A004112), corresponding to the values 1.85082, 2.403, 3.52532, 6.87285, 225.953, 227.503, ....
REFERENCES:
Abramowitz, M. and Stegun, I. A. (Eds.). "Circular Functions." §4.3 in Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, pp. 71-79, 1972.
Beyer, W. H. CRC Standard Mathematical Tables, 28th ed. Boca Raton, FL: CRC Press, p. 224, 1987.
Gellert, W.; Gottwald, S.; Hellwich, M.; Kästner, H.; and Künstner, H. (Eds.). VNR Concise Encyclopedia of Mathematics, 2nd ed. New York: Van Nostrand Reinhold, 1989.
Gradshteyn, I. S. and Ryzhik, I. M. Tables of Integrals, Series, and Products, 6th ed. San Diego, CA: Academic Press, 2000.
Harris, J. W. and Stocker, H. "Secant and Cosecant." §5.34 in Handbook of Mathematics and Computational Science. New York: Springer-Verlag, pp. 300-307, 1998.
Jeffrey, A. "Trigonometric Identities." §2.4 in Handbook of Mathematical Formulas and Integrals, 2nd ed. Orlando, FL: Academic Press, pp. 111-117, 2000.
Sloane, N. J. A. Sequences A004112, A046976, A046977, A092837, and A092838 in "The On-Line Encyclopedia of Integer Sequences."
Spanier, J. and Oldham, K. B. "The Secant 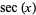 and Cosecant
and Cosecant 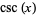 Functions." Ch. 33 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 311-318, 1987.
Functions." Ch. 33 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 311-318, 1987.
Tropfke, J. Teil IB, §3. "Die Begriffe von Sekans und Kosekans eines Winkels." In Geschichte der Elementar-Mathematik in systematischer Darstellung mit besonderer Berücksichtigung der Fachwörter, fünfter Band, zweite aufl. Berlin and Leipzig, Germany: de Gruyter, pp. 28-30, 1923.
Trott, M. The Mathematica GuideBook for Programming. New York: Springer-Verlag, 2004. http://www.mathematicaguidebooks.org/.
Zwillinger, D. (Ed.). "Trigonometric or Circular Functions." §6.1 in CRC Standard Mathematical Tables and Formulae. Boca Raton, FL: CRC Press, pp. 452-460, 1995.



|
|
|
|
التوتر والسرطان.. علماء يحذرون من "صلة خطيرة"
|
|
|
|
|
|
|
مرآة السيارة: مدى دقة عكسها للصورة الصحيحة
|
|
|
|
|
|
|
نحو شراكة وطنية متكاملة.. الأمين العام للعتبة الحسينية يبحث مع وكيل وزارة الخارجية آفاق التعاون المؤسسي
|
|
|