

تاريخ الرياضيات

الاعداد و نظريتها

تاريخ التحليل

تار يخ الجبر

الهندسة و التبلوجي


الرياضيات في الحضارات المختلفة

العربية

اليونانية

البابلية

الصينية

المايا

المصرية

الهندية


الرياضيات المتقطعة

المنطق

اسس الرياضيات

فلسفة الرياضيات

مواضيع عامة في المنطق


الجبر

الجبر الخطي

الجبر المجرد

الجبر البولياني

مواضيع عامة في الجبر

الضبابية

نظرية المجموعات

نظرية الزمر

نظرية الحلقات والحقول

نظرية الاعداد

نظرية الفئات

حساب المتجهات

المتتاليات-المتسلسلات

المصفوفات و نظريتها

المثلثات


الهندسة

الهندسة المستوية

الهندسة غير المستوية

مواضيع عامة في الهندسة

التفاضل و التكامل


المعادلات التفاضلية و التكاملية

معادلات تفاضلية

معادلات تكاملية

مواضيع عامة في المعادلات


التحليل

التحليل العددي

التحليل العقدي

التحليل الدالي

مواضيع عامة في التحليل

التحليل الحقيقي

التبلوجيا

نظرية الالعاب

الاحتمالات و الاحصاء

نظرية التحكم

بحوث العمليات

نظرية الكم

الشفرات

الرياضيات التطبيقية

نظريات ومبرهنات


علماء الرياضيات

500AD

500-1499

1000to1499

1500to1599

1600to1649

1650to1699

1700to1749

1750to1779

1780to1799

1800to1819

1820to1829

1830to1839

1840to1849

1850to1859

1860to1864

1865to1869

1870to1874

1875to1879

1880to1884

1885to1889

1890to1894

1895to1899

1900to1904

1905to1909

1910to1914

1915to1919

1920to1924

1925to1929

1930to1939

1940to the present

علماء الرياضيات

الرياضيات في العلوم الاخرى

بحوث و اطاريح جامعية

هل تعلم

طرائق التدريس

الرياضيات العامة

نظرية البيان
Liouville,s Constant
المؤلف:
Apostol, T. M.
المصدر:
Modular Functions and Dirichlet Series in Number Theory, 2nd ed. New York: Springer-Verlag
الجزء والصفحة:
...
1-2-2021
2809
Liouville's Constant
Liouville's constant, sometimes also called Liouville's number, is the real number defined by
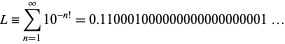 |
(OEIS A012245). Liouville's constant is a decimal fraction with a 1 in each decimal place corresponding to a factorial 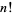 , and zeros everywhere else. Liouville (1844) constructed an infinite class of transcendental numbers using continued fractions, but the above number was the first decimal constant to be proven transcendental (Liouville 1850). However, Cantor subsequently proved that "almost all" real numbers are in fact transcendental.
, and zeros everywhere else. Liouville (1844) constructed an infinite class of transcendental numbers using continued fractions, but the above number was the first decimal constant to be proven transcendental (Liouville 1850). However, Cantor subsequently proved that "almost all" real numbers are in fact transcendental.
A recurrence plot of the binary digits is illustrated above.
Liouville's constant nearly satisfies
 |
which has solution 0.1100009999... (OEIS A093409), but plugging 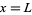 into this equation gives
into this equation gives  instead of 0.
instead of 0.
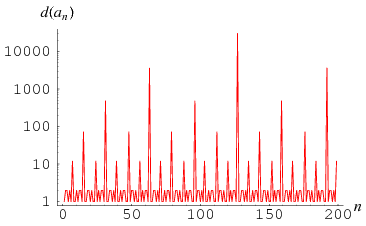
Liouville's constant has continued fraction [0, 9, 11, 99, 1, 10, 9, 999999999999, 1, 8, 10, 1, 99, 11, 9, 999999999999999999999999999999999999999999999999999999999999999999999999, ...] (OEIS A058304; Stark 1994, pp. 172-177), which shows sporadic large terms. The numbers of digits 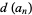 in the
in the  th term is plotted above on a semilog plot, which shows a nested structure (E. Zeleny, pers. comm., Aug. 17, 2005). Interestingly, the
th term is plotted above on a semilog plot, which shows a nested structure (E. Zeleny, pers. comm., Aug. 17, 2005). Interestingly, the 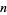 th incrementally largest term (considering only those entirely of 9s in order to exclude the term
th incrementally largest term (considering only those entirely of 9s in order to exclude the term 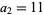 ) occurs precisely at position
) occurs precisely at position  , and this term consists of
, and this term consists of 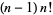 9s.
9s.
REFERENCES:
Apostol, T. M. Modular Functions and Dirichlet Series in Number Theory, 2nd ed. New York: Springer-Verlag, p. 147, 1997.
Conway, J. H. and Guy, R. K. "Liouville's Number." In The Book of Numbers. New York: Springer-Verlag, pp. 239-241, 1996.
Courant, R. and Robbins, H. "Liouville's Theorem and the Construction of Transcendental Numbers." §2.6.2 in What Is Mathematics?: An Elementary Approach to Ideas and Methods, 2nd ed. Oxford, England: Oxford University Press, pp. 104-107, 1996.
Liouville, J. "Mémoires et communications des Membres et des correspondants de l'Académie." C. R. Acad. Sci. Paris 18, 883-885, 1844.
Liouville, J. "Nouvelle démonstration d'un théor'eme sur les irrationalles algébriques, inséré dans le Compte rendu de la dernière séance." C. R. Acad. Sci. Paris 18, 910-911, 1844.
Liouville, J. "Sur des classes très-étendues de quantités dont la valeur n'est ni algébrique, ni même réductible à des irrationelles algébriques." J. Math. pures appl. 16, 133-142, 1851.
Sloane, N. J. A. Sequences A012245, A058304, and A093409 in "The On-Line Encyclopedia of Integer Sequences."
Stark, H. M. An Introduction to Number Theory. Cambridge, MA: MIT Press, 1994.
Wells, D. The Penguin Dictionary of Curious and Interesting Numbers. Middlesex, England: Penguin Books, p. 26, 1986.
 الاكثر قراءة في نظرية الاعداد
الاكثر قراءة في نظرية الاعداد
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة

الآخبار الصحية















 (نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام) قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى)
قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى) قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى)
قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى)

















