Definition Let σ and τ be simplices in R^k . We say that τ is a face of σ if the set of vertices of τ is a subset of the set of vertices of σ. A face of σ is said to be a proper face if it is not equal to σ itself. An r-dimensional face of σ is referred to as an r-face of σ. A 1-dimensional face of σ is referred to as an edge of σ.

Note that any simplex is a face of itself. Also the vertices and edges of any simplex are by definition faces of the simplex.

Definition A finite collection K of simplices in R^k is said to be a simplicial complex if the following two conditions are satisfied:—

• if σ is a simplex belonging to K then every face of σ also belongs to K,

• if σ₁ and σ₂ are simplices belonging to K then either σ₁ ∩ σ₂ = ∅ or else σ₁ ∩ σ₂ is a common face of both σ₁ and σ₂.

The dimension of a simplicial complex K is the greatest non-negative integer n with the property that K contains an n-simplex. The union of all the simplices of K is a compact subset |K| of R^k referred to as the polyhedron of K. (The polyhedron is compact since it is both closed and bounded in R^k .)

Example Let K_σ consist of some n-simplex σ together with all of its faces.

Then K_σ is a simplicial complex of dimension n, and |K_σ| = σ.

Lemma 1.2 Let K be a simplicial complex, and let X be a topological space.

A function f: |K| → X is continuous on the polyhedron |K| of K if and only if the restriction of f to each simplex of K is continuous on that simplex.

Proof If a topological space can be expressed as a finite union of closed subsets, then a function is continuous on the whole space if and only if its restriction to each of the closed subsets is continuous on that closed set. The required result is a direct application of this general principle.

We shall denote by Vert K the set of vertices of a simplicial complex K  (i.e., the set consisting of all vertices of all simplices belonging to K). A collection of vertices of K is said to span a simplex of K if these vertices are the vertices of some simplex belonging to K.

Definition: Let K be a simplicial complex in R^k . A subcomplex of K is a collection L of simplices belonging to K with the following property:

• if σ is a simplex belonging to L then every face of σ also belongs to L.

 Note that every subcomplex of a simplicial complex K is itself a simplicial complex.

Definition Let v₀, v₁, . . . , v_q be the vertices of a q-simplex σ in some Euclidean space R^k . We define the interior of the simplex σ to be the set of all points of σ that are of the form 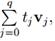 where t_j > 0 for j = 0, 1, . . . , q and  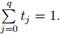One can readily verify that the interior of the simplex σ consists of all points of σ that do not belong to any proper face of σ. (Note that, if σ ∈ R^k, then the interior of a simplex defined in this fashion will not coincide with the topological interior of σ unless dim σ = k.)

Note that any point of a simplex σ belongs to the interior of a unique face of σ. Indeed let v₀, v₁, . . . , v_q be the vertices of σ, and let x ∈ σ. Then

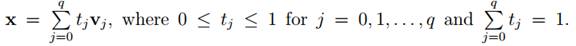
The unique face of σ containing x in its interior is then the face spanned by those vertices v_j for which t_j > 0.

Lemma 1.3 Let K be a finite collection of simplices in some Euclidean space R^k , and let |K| be the union of all the simplices in K. Then K is a simplicial complex (with polyhedron |K|) if and only if the following two conditions are satisfied:

• K contains the faces of its simplices,

• every point of |K| belongs to the interior of a unique simplex of K.

Proof Suppose that K is a simplicial complex. Then K contains the faces of its simplices. We must show that every point of |K| belongs to the interior of a unique simplex of K. Let x ∈ |K|. Then x belongs to the interior of a face σ of some simplex of K (since every point of a simplex belongs to the interior of some face). But then σ ∈ K, since K contains the faces of all its simplices. Thus x belongs to the interior of at least one simplex of K.

Suppose that x were to belong to the interior of two distinct simplices σ and τ of K. Then x would belong to some common face σ ∩ τ of σ and τ  (since K is a simplicial complex). But this common face would be a proper face of one or other of the simplices σ and τ (since σ ≠τ ), contradicting the fact that x belongs to the interior of both σ and τ . We conclude that the simplex σ of K containing x in its interior is uniquely determined, as required.

Conversely, we must show that any collection of simplices satisfying the given conditions is a simplicial complex. Since K contains the faces of all its simplices, it only remains to verify that if σ and τ are any two simplices of K with non-empty intersection then σ ∩ τ is a common face of σ and τ .

Let x ∈ σ ∩ τ . Then x belongs to the interior of a unique simplex ω of K. However any point of σ or τ belongs to the interior of a unique face of that simplex, and all faces of σ and τ belong to K. It follows that ω is a common face of σ and τ , and thus the vertices of ω are vertices of both σ and τ . We deduce that the simplices σ and τ have vertices in common, and that every point of σ ∩ τ belongs to the common face ρ of σ and τ spanned by these common vertices. But this implies that σ ∩ τ = ρ, and thus σ ∩ τ is a common face of both σ and τ , as required.

Definition : A triangulation (K, h) of a topological space X consists of a simplicial complex K in some Euclidean space, together with a homeomorphism h: |K| → X mapping the polyhedron |K| of K onto X.

The polyhedron of a simplicial complex is a compact Hausdorff space.  Thus if a topological space admits a triangulation then it must itself be a compact Hausdorff space.

Lemma 1.4 Let X be a Hausdorff topological space, let K be a simplicial complex, and let h: |K| → X be a bijection mapping |K| onto X. Suppose that the restriction of h to each simplex of K is continuous on that simplex. Then the map h: |K| → X is a homeomorphism, and thus (K, h) is a triangulation of X.

Proof Each simplex of K is a closed subset of |K|, and the number of simplices of K is finite. It follows from Lemma 1.2 that h: |K| → X is continuous.

اخر الاخبار

اخبار العتبة العباسية المقدسة

أهالي كربلاء يحيون اليوم السابع لذكرى شهادة الإمام الحسين (عليه السلام)

مشروع أمير القراء الوطني يشهد مشاركة أكثر من 100 طالبٍ في مرحلته الثالثة

قسم الشؤون الفكرية يصدر دليلًا يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة

قسم الشؤون الفكرية يعلن عن توفير عددٍ من المكتبات الإلكترونية المتخصّصة للمؤسّسات الدينية والمكتبات العامّة

المزيد

الآخبار الصحية

نصائح عملية للحد من التعرق المفرط في الصيف

أطباء يحذرون: مكمل غذائي شائع قد يدمر الجهاز العصبي

تحذير طبي من تأثير جانبي "غير متوقع" لحقن التخسيس

حمامات الثلج قد تكون خطيرة وأحيانا مميتة.. ما السبب؟

المزيد

الآخبار التكنلوجية

أكبر جدارية شمسية في العالم تدخل موسوعة غينيس.. مشروع ضخم

تسارع دوران الأرض يثير القلق.. وتحذيرات من "كوارث محتملة" ؟

"سوذبيز" تطرح أكبر قطعة من المريخ على الأرض في مزاد علني

أوبن أيه آي تعتزم إطلاق متصفح إنترنت يعمل بالذكاء الاصطناعي

المزيد

مواضيع ذات صلة

Triple Torus

Thurston Elliptization Conjecture

Thurston,s Geometrization Conjecture

Real Projective Plane

Pseudometric Topology

Pseudocrosscap

Parallelizable

Möbius Strip

Möbius Shorts

Path

Product Topology

Handle