Amphichiral Knot

An amphichiral knot is a knot that is capable of being continuously deformed into its own mirror image. More formally, a knot 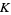 is amphichiral (also called achiral or amphicheiral) if there exists an orientation-reversing homeomorphism of  mapping  to itself (Hoste et al. 1998). (If the words "orientation-reversing" are omitted, all knots are equivalent to their mirror images.)

Knots on ten and fewer crossing can be tested in the Wolfram Language to see if they are amphichiral using the command KnotData[knot, "Amphichiral"].

There are 20 amphichiral knots having ten or fewer crossings, namely 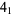 (the figure eight knot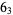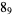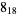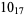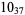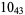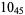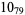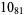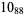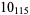), , , , , , , , , , , , , , , , , , , and 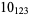 (Jones 1985), the first few of which are illustrated above.

The following table gives the total number of prime amphichiral knots, number of 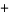 amphichiral noninvertible prime knots,  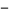amphichiral noninvertible prime knots, and fully amphichiral invertible knots prime knots (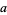) with  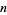crossings, starting with .

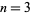type	OEIS	counts
amph.	A052401	0, 1, 0, 1, 0, 5, 0, 13, 0, 58, 0, 274, 1, ...
	A051767	0, 0, 0, 0, 0, 0, 0, 0, 0, 1, 0, 6, 0, 65, ...
	A051768	0, 0, 0, 0, 0, 1, 0, 6, 0, 40, 0, 227, 1, ...
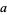	A052400	0, 1, 0, 1, 0, 4, 0, 7, 0, 17, 0, 41, 0, 113, ...

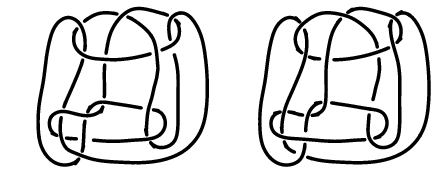
Prime amphichiral alternating knots can only exist for even 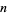, but the 15-crossing nonalternating amphichiral knot illustrated above was discovered by Hoste et al. (1998). It is the only known prime nonalternating amphichiral knot with an odd number of crossings.

The HOMFLY polynomial is good at identifying amphichiral knots, but sometimes fails to identify knots which are not. No knot invariant which always definitively determines if a knot is amphichiral is known.

Let 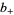 be the sum of positive exponents, and  the sum of negative exponents in the braid group . If

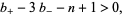then the knot corresponding to the closed braid 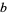 is not amphichiral (Jones 1985).

REFERENCES:

Burde, G. and Zieschang, H. Knots, 2nd rev. ed. Berlin: de Gruyter, pp. 311-319, 2002.

Haseman, M. G. "On Knots, with a Census of the Amphicheirals with Twelve Crossings." Trans. Roy. Soc. Edinburgh 52, 235-255, 1917.

Haseman, M. G. "Amphicheiral Knots." Trans. Roy. Soc. Edinburgh 52, 597-602, 1918.

Hoste, J.; Thistlethwaite, M.; and Weeks, J. "The First  Knots." Math. Intell. 20, 33-48, Fall 1998.

Jones, V. "A Polynomial Invariant for Knots via von Neumann Algebras." Bull. Amer. Math. Soc. 12, 103-111, 1985.

Jones, V. "Hecke Algebra Representations of Braid Groups and Link Polynomials." Ann. Math. 126, 335-388, 1987.

Sloane, N. J. A. Sequences A051767, A051768, A052400, and A052401 in "The On-Line Encyclopedia of Integer Sequences."