Commutative Diagram
المؤلف:
Cartan H. and Eilenberg, S
المصدر:
Homological Algebra. Princeton, NJ: Princeton University Press, 1956.
الجزء والصفحة:
...
8-5-2021
2173
Commutative Diagram
A commutative diagram is a collection of maps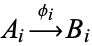 
in which all map compositions starting from the same set 
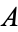and ending with the same set 
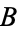give the same result. In symbols this means that, whenever one can form two sequences
 |
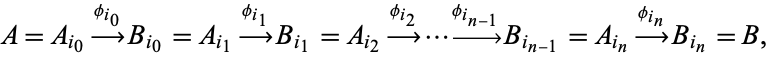(1)
|
and
 |
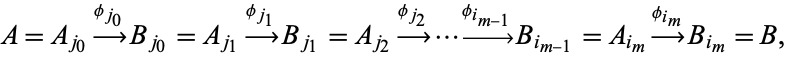(2)
|
the following equality holds:
 |
(3)
|
Commutative diagrams are usually composed by commutative triangles and commutative squares.
Commutative triangles and squares can also be combined to form plane figures or space arrangements.
A commutative diagram can also contain multiple arrows that indicate different maps between the same two sets.
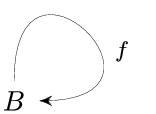
A looped arrow indicates a map from a set to itself.
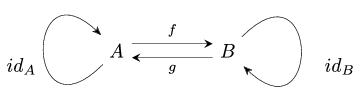
The above commutative diagram expresses the fact that 
is the inverse map to 
, since it is a pictorial translation of the map equalities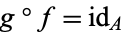 
and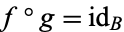 
.
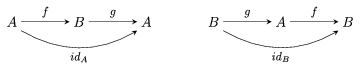
This can also be represented using two separate diagrams.
Many other mathematical concepts and properties, especially in algebraic topology, homological algebra, and category theory, can be formulated in terms of commutative diagrams.
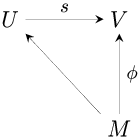
For example, a module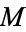 
is projective iff any surjective module homomorphism 
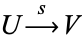and any module homomorphism 
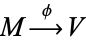can be completed to a commutative diagram.
Similarly, one can characterize the dual notion of injective module: a module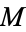 
is injective iff any injective module homomorphism and 
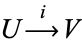and any module homomorphism 
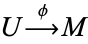can be completed to a commutative triangle.
According to Baer's criterion, it is sufficient to require this condition for the inclusion maps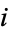 
of the ideals of 
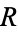in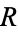 
.
Another example of a notion based on diagrams is the chain homomorphism, which can be visualized as a sequence of commutative squares.
The advantage of drawing commutative diagrams is the possibility to seize any given map configuration at a glance. The picture also facilitates the task of composing maps, which is like following directed paths from set to set. Many homological theorems are proven by studying commutative diagrams: this method is usually referred to as "diagram chasing."
REFERENCES:
Bourbaki, N. "Diagrammes commutatifs." §1.1 in Algèbre. Chap. 10, Algèbre Homologique. Paris, France: Masson, 1-3, 1980.
Cartan H. and Eilenberg, S. Homological Algebra. Princeton, NJ: Princeton University Press, 1956.
Davis, J. F. and Kirk, P. Lecture Notes in Algebraic Topology. Providence, RI: Amer. Math. Soc., 2001.
Eilenberg, S. and Steenrod, N. Foundations of Algebraic Topology. Princeton, NJ: Princeton University Press, 1952.
Herrlich, H. and Strecker, G. E. Category Theory: An Introduction. Boston, MA: Allyn and Bacon, 1973.
Hilton, P. J. and Stammbach, U. A Course in Homological Algebra, 2nd ed. New York: Springer-Verlag, 1997.
Lang, S. Algebra, rev. 3rd ed. New York: Springer-Verlag, 2002.
Mac Lane, S. Categories for the Working Mathematician. New York: Springer-Verlag, 1971.
Mac Lane, S. Homology. Berlin: Springer-Verlag, 1967.
Mitchell, B. Theory of Categories. New York: Academic Press, 1965.
Northcott, D. G. An Introduction to Homological Algebra. Cambridge, England: Cambridge University Press, 1966.
Rotman, J. J. An Introduction to Algebraic Topology. New York: Springer-Verlag, 1988.
Scott Osborne, M. Basic Homological Algebra. New York: Springer-Verlag, 2000.
الاكثر قراءة في التبلوجيا
اخر الاخبار
اخبار العتبة العباسية المقدسة
