Definition

Domain: The domain of a function is the set of all possible input values (often the "x" variable), which produce a valid output from a particular function. It is the set of all real numbers for which a function is mathematically defined.

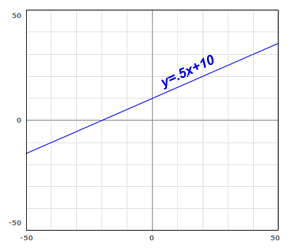
Most often a simple function's domain is all real numbers. Consider a simple linear equation like the graph shown below drawn from the function y=.5x+10. What values are valid inputs? It's not a trick question -- every real number! Its range is all real numbers because there is nothing you can put in for x that won't work. That's why the graph extends forever in the x directions (left and right).

What kind of functions don't have a domain of all real numbers? Well, if the domain is the set of all real numbers for which the function is defined, then logically we're looking for a function that has certain input values that do not produce a valid output, i.e., the function is undefined for that input. Here is an example:

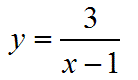
This function is defined for almost any real x. But, what is the value of y when x=1? Well, it's 3 divided by 0, which is undefined. Therefore 1 is not in the domain of this function. All other real numbers are valid inputs, so the domain is all real numbers except for x=1.

What other kinds of functions have domains that aren't all real numbers? Certain "inverse" functions, like the inverse trig functions, have limited domains as well. Since the sine function can only haveoutputs from -1 to 1, its inverse can only accept inputs from -1 to 1. The domain of inverse sine is -1 to 1. However, the most common reason for limited domains is probably the divide by zero issue. When finding the domain of a function, first look for any values that cause you to divide by zero. Remember also that we cannot take the square root of a negative number, so keep an eye out for situations where the radicand (the "stuff" inside the square root sign) could result in a negative value. In that case, it would not be a valid input so the domain would not include such values.

Definition

Range: The range is the set of all possible output values (usually the variable y, or sometimes expressed as f(x)), which result from using a particular function.

The range of a simple linear function is almost always going to be all real numbers. A graph of a line, such as the one shown below on the left, will extend forever in either y direction. There's one notable exception: y=constant. When you have a function where y equals a constant (like y=3), your graph is a horizontal line. In that case, the range is just that one value. Otherwise, the range is all real numbers.

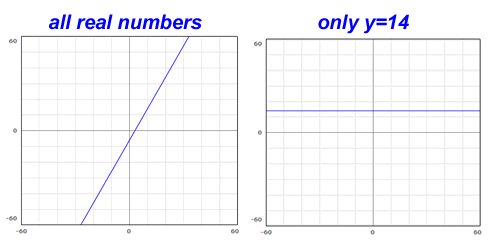
Many other functions have limited ranges. While only a few types have limited domains, you will frequenty see functions with unusual ranges. Here are a few examples:

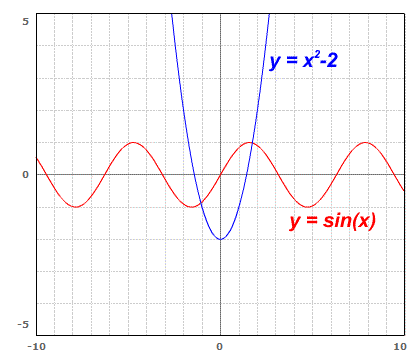
As you can see, these two functions have ranges that are limited. No matter what values you enter into a sine function you will never get a result greater than 1 or less than -1. No matter what values you enter into y=x2−2 you will never get a result less than -2.

Summary: The domain of a function is all the possible input values for which the function is defined, and the range is all possible output values.