

تاريخ الرياضيات

الاعداد و نظريتها

تاريخ التحليل

تار يخ الجبر

الهندسة و التبلوجي


الرياضيات في الحضارات المختلفة

العربية

اليونانية

البابلية

الصينية

المايا

المصرية

الهندية


الرياضيات المتقطعة

المنطق

اسس الرياضيات

فلسفة الرياضيات

مواضيع عامة في المنطق


الجبر

الجبر الخطي

الجبر المجرد

الجبر البولياني

مواضيع عامة في الجبر

الضبابية

نظرية المجموعات

نظرية الزمر

نظرية الحلقات والحقول

نظرية الاعداد

نظرية الفئات

حساب المتجهات

المتتاليات-المتسلسلات

المصفوفات و نظريتها

المثلثات


الهندسة

الهندسة المستوية

الهندسة غير المستوية

مواضيع عامة في الهندسة

التفاضل و التكامل


المعادلات التفاضلية و التكاملية

معادلات تفاضلية

معادلات تكاملية

مواضيع عامة في المعادلات


التحليل

التحليل العددي

التحليل العقدي

التحليل الدالي

مواضيع عامة في التحليل

التحليل الحقيقي

التبلوجيا

نظرية الالعاب

الاحتمالات و الاحصاء

نظرية التحكم

بحوث العمليات

نظرية الكم

الشفرات

الرياضيات التطبيقية

نظريات ومبرهنات


علماء الرياضيات

500AD

500-1499

1000to1499

1500to1599

1600to1649

1650to1699

1700to1749

1750to1779

1780to1799

1800to1819

1820to1829

1830to1839

1840to1849

1850to1859

1860to1864

1865to1869

1870to1874

1875to1879

1880to1884

1885to1889

1890to1894

1895to1899

1900to1904

1905to1909

1910to1914

1915to1919

1920to1924

1925to1929

1930to1939

1940to the present

علماء الرياضيات

الرياضيات في العلوم الاخرى

بحوث و اطاريح جامعية

هل تعلم

طرائق التدريس

الرياضيات العامة

نظرية البيان
Functions-Inverse Functions and Permutations
المؤلف:
Ivo Düntsch and Günther Gediga
المصدر:
Sets, Relations, Functions
الجزء والصفحة:
42-44
14-2-2017
2210
Recall Definition 1.3in( One–one, Onto, and Bijective Functions) of the converse R˘ of a relation R on a set A;
we have obtained the converse R˘ of R by turning around all the arrows, resp. the order of the components. This was always possible, since a relation was just a set of ordered pairs with no other conditions attached.
For a function, reversing the arrows need not result in another function: Consider the examples in Figure 1.1in ( One–one, Onto, and Bijective Functions) . In the surjective function, turning around the arrows does not result in a function, because there are two arrows leaving e.
Furthermore, you will notice that applying f to an element of dom f and then applying g to the result gets us right back to where we started, in other words, g(f(a)) = a. This property is decisive, and leads to the following
Definition 1.1. Let f : A → B and g : B → A be functions; g is called the inverse of f, denoted by f−1 , if g(f(x)) = x for all x ∈ A.
In other words, g is an inverse of f if and only if g ◦ f = idA. The examples above suggest that a function which is not bijective cannot have an inverse. The following Theorem shows that this observation is correct.
Theorem 1.1. f : A → B has an inverse if and only if f is injective.
Proof. “⇒”: Suppose that g : B → A is an inverse of f; we have to show that f is one–one. Let x,y ∈ A and f(x) = f(y); then, g(f(x)) = g(f(y)). Since g is an inverse of f, we have g(f(x)) = x and g(f(y)) = y, which implies x = y.
“⇐”: Suppose that f : A → B is one–one; for each y ∈ B we distinguish two cases:
1. y ∈ ran f: Then there is exactly one x ∈ A such that f(x) = y, since f is one–one. Now set g(y) = x.
2. x ∉ran f: Choose an arbitrary element yx of A, and set g(y) = yx. So, dom g = B, codom g = A, and, since, f is one–one, each element of B is assigned exactly one element of A; hence, g : B → A is a function.
Finally, let x ∈ A; by definition of g we have g(f(x)) = x, which shows that g is an inverse of f.
Note that in case f is one–one but not onto it has more than one inverse function, since g is defined arbitrarily for every x ∈ B which is not in the range of f. Those elements of the codomain which are not in the range of f are in a way immaterial to the assignment f; from previous examples we know that a one–one function can be made bijective by restricting its codomain to the range. If f is bijective then there is only one inverse function:
Theorem 1.2. If f : A → B is bijective, then f has unique inverse g : B → A; furthermore, f is an inverse to g.
Proof. Since f is bijective, it is one–one, and thus it has an inverse g : B → A.
Suppose that h : B → A is also an inverse to f, i.e. we have h(f(x)) = x for every x ∈ A. Since in particular f is onto, for every y ∈ B there is an x ∈ A such that f(x) = y; now, since g is an inverse to f, we have g(y) = g(f(x)) = x, and since h is also an inverse to f, we have h(y) = h(f(x)) = x. It follows that g(y) = h(y) for all y ∈ B, which shows that g = h.
For the second part we have to show that f(g(y)) = y for all y B. Thus, let y ∈ B.
Since f is onto, there exists an x ∈ A such that f(x) = y. Thus,
f(g(y)) = f(g(f(x))) = f(x),
since g is an inverse of f, and hence, g(f(x)) = x.
Let us briefly look at bijective functions f : A → A, where A is a finite set.
Definition 1.2. Let A = {1, 2, 3,. . ., n}, and f : A → A be a bijective function;
then f is called a permutation on n.
The reason for calling these function permutations is that they arrange (i.e. permute) the elements of Ain a different order. Amore general definition of a permutation would allow as domain arbitrary finite sets. For example, if you have n books arranged on a shelf and you put them in a different order, you have in fact performed a permutation of n objects.
If n is small there is a convenient way of picturing f; for example if A = {1, 2, 3, 4, 5}, and f(1) = 1, f(2) = 3, f(3) = 5, f(4) = 2, f(5) = 4, then we can write

Observe that every element of A appears exactly once in each of the two lines, since f is bijective. In general, if A = {1, 2,. . ., n}, and f(1) = a1,f(2) = a2,. . ., f(n) = an, we can list the function by

It is easy to find the inverse of a permutation by just looking from bottom to top in the given list. Let us go back to the example above: The inverse g of f looks like this:

This method of representing a permutation is also useful for obtaining the composition; look at the following two permutations of A:
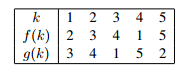
By going through the tables, we find that for h = g ◦ f,

 الاكثر قراءة في نظرية المجموعات
الاكثر قراءة في نظرية المجموعات
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة

الآخبار الصحية















 قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة
قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة "المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة
"المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة (نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)


















