1. A and B are sets, and f ⊆ A × B,

2. For every x ∈ A there is some y ∈ B such that 〈x ,y〉 ∈ f

3. If 〈x ,y〉 ∈ f and 〈x ,z〉 ∈ f, then y = z; in other words, the assignment is unique in the sense that an x ∈ A is assigned at most one element of B.

A is called the domain of f, and B its codomain.

It is customary to write the function 〈f,A, B〉 as f : A → B. Also, if 〈x ,y〉 ∈ f,  then we will usually write y = f(x), and call y the image of x under f.

The set {y ∈ B : There is an x ∈ A such that y = f(x)} is called the range of f.

Observe that the range of f is always a subset of the codomain. Observe carefully the distinction between f(x) and f: Whereas f(x) is an element of the codomain,  f is the rule of assignment, conveniently expressed as a subset of A × B.

Suppose that f : A → B and g : C → D are functions. It follows from the definition of a function that they are equal if and only if

1. A = C,

2. B = D,

3. f = g.

If for a function f : A → B it is clear what A and B are, we sometimes call the function simply f, but we must keep in mind that a function is only properly defined if we also give a domain and a codomain!

Usually, after having agreed on a domain and a codomain, f is given by a rule,

e.g. f(x) = x² , f(t) = sin t,f(n) = n + 1. As in the previous section

with relations, we sometimes use a diagram to describe a function; for example,  the diagram of Figure 1.1 describes the function f : A → B, where A = {1, −1, 0, −2} is the domain of f,B = {1, 0, 2, 4, } is its codomain, and f = {〈1, 1〉,〈−1, 1〉,〈0, 0〉,〈−2, 4〉}.

Figure 1.1: Function arrow diagram

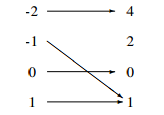
Observe that for all x ∈ A, f(x) = x² . This can also be indicated by writing x → x² .

 The definition of a function implies that each element of A is the origin of exactly one arrow; it does not imply that at each element of B is the target of an arrow, or that only one arrow from A points to a single element of B. Functions with these properties have special names, and we shall look at them in a later section.

Definition 1.2. Let f : A → B be a function.

1. If A = B and f(x) = x for all x ∈ A, the f is called the identity function on A, and it is denoted by idA.

2. If A ⊆ B and f(x) = x for all x ∈ A, then f is called the inclusion function from A to B, or, if no confusion can arise, simply the inclusion. Observe that, if A = B and f is the inclusion, then f is in fact the identity on A.

3. If f(x) = x for some x ∈ A, then x is called a fixed point of f.

4. If f(x) = b for all x ∈ A, then f is called a constant function.

5. If g : C → D is a function such that A ⊆ C, B ⊆ D, and f ⊆ g, then g is

called an extension of f over C, and f is called the restriction of g to A.

While a function may have many different extension, it can only have one restriction to a subset of its domain. The following diagrams shall illustrate these situations:

Consider the assignment h(x) = (sin x)² , and suppose that dom h = codom h = R. To find h(x) for a given x, we do two things:

1. First find sin x and set y = sin x;

2. Then find y².

If we look close enough, we find that we have actually used two functions to find h(x):

1. f : R → R, f(x) = sin x,

2. g : R → R, g(y) = y²,

This shows that h(x) = g(f(x)): We have first applied f to x, found that f(x) is an element of dom g, and then applied g to f(x). This brings us to

Definition 1.3. Let f : A → B and g : C → D be functions such that ran f ⊆ dom g. Then the function g ◦ f : A → C defined by (g ◦ f)(x) = g(f(x)) is called the (functional) composition of f and g.

the reason for using a different interpretation of composition for functions is historical, and we shall not go into details.

Lemma 1.1. If f and g are functions such that ran f ⊆ dom g, then dom(g ◦ f) = dom f, and codom(g ◦ f) = codom g.

Proof. This follows immediately from the definition of composite functions.

One the most useful properties of functional composition is the following:

Lemma .1.2. Let f : A → B, g : B → C, h : C → D be functions. Then,  h ◦ (g ◦ f) = (h ◦ g) ◦ f, i.e. the composition of functions is associative.

Proof. Let p = h ◦ (g ◦ f), and q = (h ◦ g) ◦ f; to show that two functions are equal we use the remarks following to find their domains and co-domains.

Looking first at p, we see that p is the composite of the functions g ◦ f and h, so,  we will have to look at g ◦ f first. Now, dom(g ◦ f) = dom f = A by Lemma

1.1, thus, dom p = dom h ◦ (g ◦ f) = A. Next,

 codom p = codom h ◦ (g ◦ f) = codom h = D,  also by Lemma 1.1.

Looking at q, we see by a similar reasoning that dom q = A and that codom q = codom(h ◦ g). Since codom(h ◦ g) = codom h, we have codom h = D; thus, we find that dom p = dom q, and codom p = codom q.

All that is left to show is that p = q, i.e. that p(x) = q(x) for all x ∈ A. Let x ∈ A;

then,

p(x) = (h ◦ (g ◦ f))(x)

   = h((g ◦ f)(x))

 = h(g(f(x)))

     = (h ◦ g)(f(x))

         = ((h ◦ g) ◦ f)(x)

= q(x).

اخر الاخبار

اخبار العتبة العباسية المقدسة

بمشاركة الأمين العام للعتبة العبّاسية المقدّسة.. موكب عزاء السادة الخَدَم يحيي ليلة عاشوراء

صحفيّون يشيدون بالتسهيلات الإعلامية التي تقدّمها العتبة العباسية المقدّسة خلال زيارة عاشوراء

أهالي كربلاء ينظّمون مسيرة الشموع الحسينية إحياءً لليلة العاشر من شهر المحرّم

عبر مجلس عزاء.. العتبة العباسية المقدّسة تحيي ليلة اليوم العاشر من المحرّم

المزيد

الآخبار الصحية

لهذا السبب.. إغلاق 25 مركزًا لعلاج الإدمان في مصر ؟

خلافا للاعتقاد الشائع.. علماء يكشفون أسرار بذور البطيخ ؟!

هذا ما يحدث لجسمك عند تناولك الطماطم بانتظام !

تعرف على أطعمة تسرّع "تعافي الجسم" من حروق الشمس

المزيد

الآخبار التكنلوجية

الثلوج تغطي الصحراء الأكثر جفافا في العالم!

بتلسكوب "ألما".. التقاط صورا غير مسبوقة لبدايات الكون !

هل هاتفك مراقب؟.. 5 علامات خطيرة تكشف التجسس عليك

دبي تطلق أول رحلة تجريبية للتاكسي الجوي

المزيد

مواضيع ذات صلة

Subset

Set Class

Set

Second Category

Proper Subset

Power Set

Matroid

Inductive Set

Independent Vertex Set

Independent Set

Graphoid

G_delta Set