

تاريخ الرياضيات

الاعداد و نظريتها

تاريخ التحليل

تار يخ الجبر

الهندسة و التبلوجي


الرياضيات في الحضارات المختلفة

العربية

اليونانية

البابلية

الصينية

المايا

المصرية

الهندية


الرياضيات المتقطعة

المنطق

اسس الرياضيات

فلسفة الرياضيات

مواضيع عامة في المنطق


الجبر

الجبر الخطي

الجبر المجرد

الجبر البولياني

مواضيع عامة في الجبر

الضبابية

نظرية المجموعات

نظرية الزمر

نظرية الحلقات والحقول

نظرية الاعداد

نظرية الفئات

حساب المتجهات

المتتاليات-المتسلسلات

المصفوفات و نظريتها

المثلثات


الهندسة

الهندسة المستوية

الهندسة غير المستوية

مواضيع عامة في الهندسة

التفاضل و التكامل


المعادلات التفاضلية و التكاملية

معادلات تفاضلية

معادلات تكاملية

مواضيع عامة في المعادلات


التحليل

التحليل العددي

التحليل العقدي

التحليل الدالي

مواضيع عامة في التحليل

التحليل الحقيقي

التبلوجيا

نظرية الالعاب

الاحتمالات و الاحصاء

نظرية التحكم

بحوث العمليات

نظرية الكم

الشفرات

الرياضيات التطبيقية

نظريات ومبرهنات


علماء الرياضيات

500AD

500-1499

1000to1499

1500to1599

1600to1649

1650to1699

1700to1749

1750to1779

1780to1799

1800to1819

1820to1829

1830to1839

1840to1849

1850to1859

1860to1864

1865to1869

1870to1874

1875to1879

1880to1884

1885to1889

1890to1894

1895to1899

1900to1904

1905to1909

1910to1914

1915to1919

1920to1924

1925to1929

1930to1939

1940to the present

علماء الرياضيات

الرياضيات في العلوم الاخرى

بحوث و اطاريح جامعية

هل تعلم

طرائق التدريس

الرياضيات العامة

نظرية البيان
Continuity
المؤلف:
Murray H. Protter
المصدر:
Basic Elements of Real Analysis
الجزء والصفحة:
21-27
24-11-2016
1009
Most of the functions we study in elementary calculus are described by formulas. These functions almost always possess derivatives. In fact, a portion of any first course in calculus is devoted to the development of routine methods for computing derivatives. However, not all functions possess derivatives everywhere. For example, the functions (1 + x2)/x, cot x, and sin(1/x) do not possess derivatives at x =0 no matter how they are defined at x =0.
As we progress in the study of analysis, it is important to enlarge substantially the class of functions we examine. Functions that possess derivatives everywhere form a rather restricted class; extending this class to functions that are differentiable except at a few isolated points does not enlarge it greatly. We wish to investigate significantly larger classes of functions, and to do so we introduce the notion of a continuous function.
Definitions
Suppose that f is a function from a domain D in R1 to R1. The function f is continuous at a if (i) the point a is in an open interval I contained in D, and (ii) for each positive number ε there is a positive number δ such that
|f(x) − f(a)| <ε whenever |x − a| <δ.
If f is continuous at each point of a set S, we say that f is continuous on S. A function f is called continuous if it is continuous at every point of its domain. The geometric significance of continuity at a point a is indicated in Figure 1.1. We recall that the inequality |f(x) − f(a)| <ε is equivalent to the double inequality
−ε<f(x) − f(a) < ε,
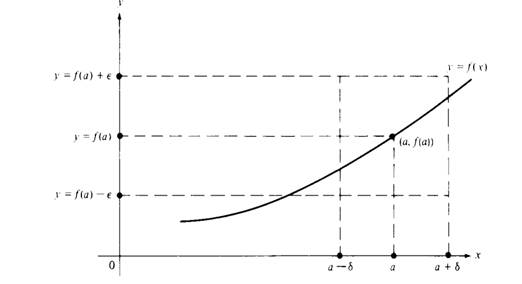
Figure 1.1 The graph of f is in the rectangle for a − δ<x<a + δ.
Or
f(a) − ε<f(x)<f(a) + ε.
Similarly, the inequality |x − a| <δ is equivalent to the two inequalities
a − δ<x<a + δ.
We construct the four lines x a − δ, x a + δ, y f(a) − ε, and y =f(a) + ε, as shown in Figure 1.1. The rectangle determined by these four lineshasitscenter at the point with coordinates (a, f(a)). The geometric interpretation of continuity at a point may be given in terms of this rectangle. A function f is continuous at a if for each ε> 0 there is a number δ> 0 such that the graph of f remains within the rectangle for all x in the interval (a − δ, a + δ).
It is usually very difficult to verify continuity directly from the definition. Such verification requires that for every positive number ε we exhibit a number δ and show that the graph of f lies in the appropriate rectangle.
However, if the function f is given by a sufficiently simple expression, it is sometimes possible to obtain an explicit value for the quantity δ corresponding to a given number ε. We describe the method by means of two examples.
Example 1
Given the function
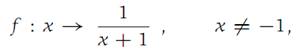
and a = 1, ε = 0.1, find a number δ such that |f(x) − f(1)| < 0.1 for |x − 1| <δ.
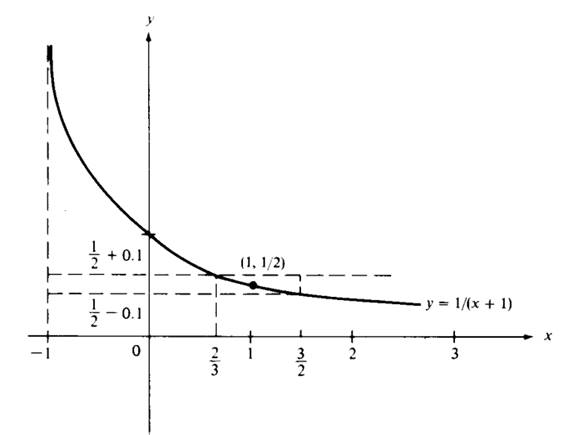
Figure 1.2
Solution. We sketch the graph of f and observe that f is decreasing for x> −1 (see Figure 1.2). The equations f(x)−f(1) = 0.1, f(x)−f(1) =−0.1 can be solved for x. We find
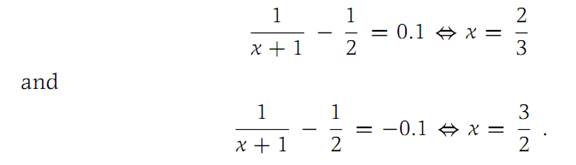

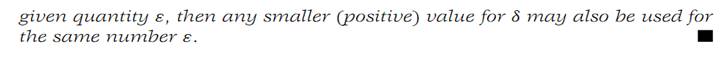
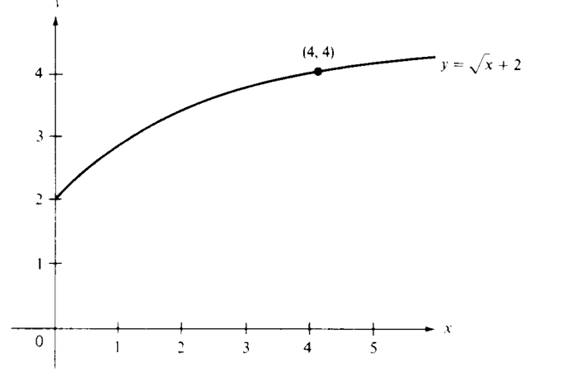
Figure 1.3
Example 2
Consider the function
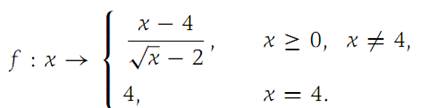
If ε = 0.01, find a δ such that |f(x) − f(4)| < 0.01 for all x such that |x − 4| <δ.
Solution.
If x ≠ 4, then factoring x − 4, we get
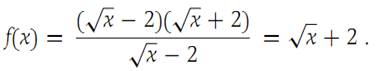
The graph of f is shown in Figure 1.3, and we observe that f is an increasing function. We solve the equations f(x) − f(4) =0.01 and f(x) − f(4) =−0.01 and obtain
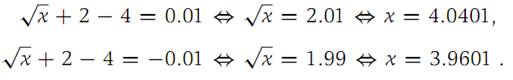
Since f is increasing, it follows that |f(x) − f(4)| < 0.01 for 3.9601 <x< 4.0401. Selecting δ = 0.0399,
we find that |f(x) − f(4)| <ε for |x − 4| <δ.
Definition
Suppose that a and L are real numbers and f is a function from a domain D in R1 to R1. The number a may or may not be in the domain of f . The function f tends to L as a limit as x tends to a if (i) there is an open interval I containing a that, except possibly for the point a, is contained in D, and (ii) for each positive number ε there is a positive number δ such that
|f(x) − L| <ε whenever 0 < |x − a| <δ.
If f tends to L as x tends to a, we write
f(x) → L as x → a
and denote the number L by
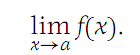
(i) We see that a function f is continuous at a if and only if a is in the domain of f and f(x) → f(a) as x → a.
(ii) The condition 0 < |x − a| <δ (excluding the possibility x= a)is used rather than the condition |x−a| <δ as in the definition of continuity, since f may not be defined at a itself.
Problems
In Problems1 through 8 the functions are continuous at the value a given. In each case find a value δ corresponding to the given value of ε such that the definition of continuity is satisfied. Draw a graph.
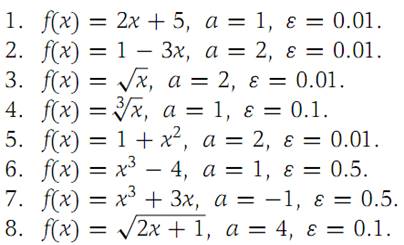
Basic Elements of Real Analysis, Murray H. Protter, Springer, 1998 Page(21-27)
 الاكثر قراءة في التحليل الحقيقي
الاكثر قراءة في التحليل الحقيقي
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة

الآخبار الصحية















 قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة
قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة "المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة
"المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة (نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)


















