Definition of Fuzzy Set

1.1 Expression for Fuzzy Set

Membership function μ _A in crisp set maps whole members in universal set X to set {0,1}.

                                              μ _A : X→{0, 1}.

Definition (Membership function of fuzzy set) In fuzzy sets, each elements is mapped to [0,1] by membership function.

                                      μ _A : X →[0, 1]

where [0,1] means real numbers between 0 and 1 (including 0,1).  

Consequently, fuzzy set is ‘vague boundary set’ comparing with crisp set.

Example 1.1 (see Fig 1.1 and 1.2) show the difference between the crisp and fuzzy sets represented by membership functions, respectively.

Example 1.2 Consider fuzzy set ‘two or so’. In this instance, universal set  X are the positive real numbers. 

                                              X = {1, 2, 3, 4, 5, 6, ….}

Membership function for A =’two or so’ in this universal set X is given  as follows:

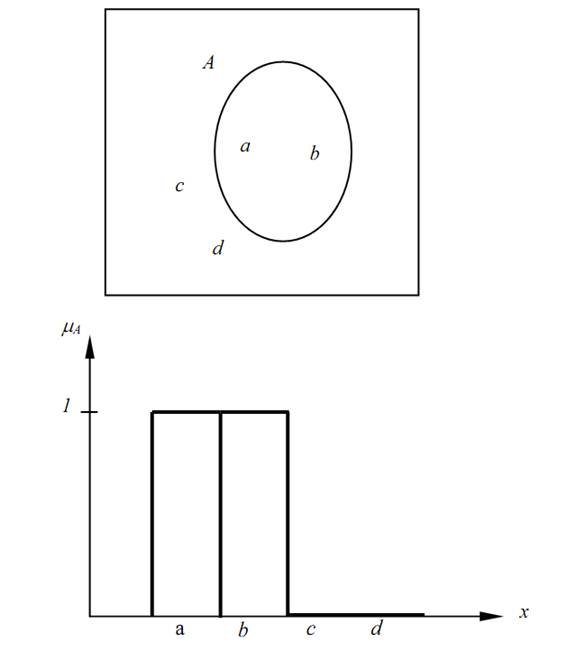
                                           Fig. 1.1. Graphical representation of crisp set

                                   Fig. 1.2. Graphical representation of fuzzy set

Usually, if elements are discrete as the above, it is possible to have  membership degree or grade as

be sure to notice that the symbol ‘+’ implies not addition but union. More generally, we use

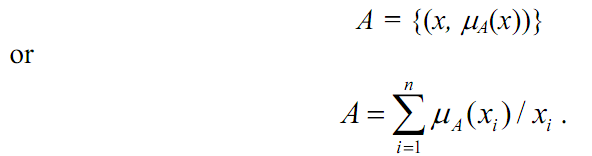
Suppose elements are continuous, then the set can be represented as follows:

For the discrimination of fuzzy set with crisp set, the symbol  A^~ is frequently used. However in this book, just notation A is used for it.

1.2 Examples of Fuzzy Set

Example 1.3 We consider statement "Jenny is young". At this time, the term "young" is vague. To represent the meaning of "vague" exactly, it

would be necessary to define its membership function as in Fig 1.2. When we refer "young", there might be age which lies in the range [0,80] and we

can account these "young age" in these scope as a continuous set. 

The horizontal axis shows age and the vertical one means the numerical value of membership function. The line shows possibility (value of

membership function) of being contained in the fuzzy set "young". 

For example, if we follow the definition of "young" as in the figure, ten year-old boy may well be young. So the possibility for the "age ten” to join

the fuzzy set of "young is 1. Also that of "age twenty seven" is 0.9. But we might not say young to a person who is over sixty and the possibility of

this case is 0. 

Now we can manipulate our last sentence to "Jenny is very young". In order to be included in the set of "very young", the age should be lowered

and let us think the line is moved leftward as in the figure. If we define fuzzy set as such, only the person who is under forty years old can be

included in the set of "very young". Now the possibility of twenty-seven year old man to be included in this set is 0.5.

That is, if we denote A= "young" and B="very young",

Example 1.4 Let’s define a fuzzy set  A ={real number near 0}. The boundary for set “real number near 0” is pretty ambiguous. The possibility

of real number x to be a member of prescribed set can be defined by the following membership function.  

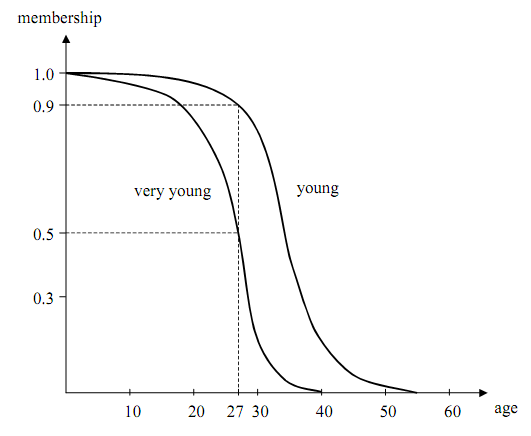
             Fig. 1.3. Fuzzy sets representing “young” and “very young”

                   Fig. 1.4. Membership function of fuzzy set “real number near 0”

Fig 1.4 shows this membership function. We can also write the fuzzy set with the function.

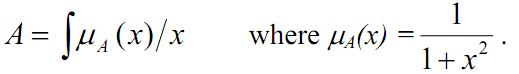
The membership degree of 1 is

the possibility of 2 is 0.2 and that of 3 is 0.1. 

Example 1.5 Another fuzzy set A ={real number very near 0} can be defined and its membership function is

the possibility of 1 is 0.25, that of 2 is 0.04 and of 3 is 0.01 (Fig 1.5).

By modifying the above function, it is able to denote membership  function of fuzzy set A = {real number near a} as,

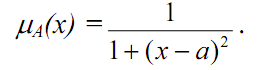
                             Fig. 1.5. Membership function for “real number very near to 0”

1.3 Expansion of Fuzzy Set

Definition (Type-n Fuzzy Set) The value of membership degree might include uncertainty. If the value of membership function is given by a

fuzzy set, it is a type-2 fuzzy set. This concept can be extended up to Type-n fuzzy set.  

Example 1.6 Consider set A= “adult”. The membership function of this set maps whole age to “youth”, “manhood” and “senior”(Fig 1.6). For

instance, for any person x, y, and z,

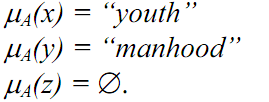
The values of membership for “youth” and “manhood” are also fuzzy sets , and thus the set “adult” is a type-2 fuzzy set. The sets “youth” and “manhood” are type-1 fuzzy sets. In the same

manner, if the values of membership function of “youth” and “manhood” are type-2, the set “adult” is type-3.  

Definition (Level-k fuzzy set) The term “level-2 set” indicates fuzzy sets whose elements are fuzzy sets (Fig 1.7). The term “level-1 set” is applicable to fuzzy sets whose elements are no fuzzy sets ordinary

elements. In the same way, we can derive up to level-k fuzzy set.

                                   Fig. 1.6. Fuzzy Set of Type-2

                                  Fig. 1.7. Level-2 Fuzzy Set

Example 1.7 In the figure, there are 3 fuzzy set elements.

1.4 Relation between Universal Set and Fuzzy Set

If there are a universal set and a crisp set, we consider the set as a subset of the universal set. In the same way, we regard a fuzzy set A as a subset of

universal set X.

Example 1.8 Let X = {a, b, c} be a universal set.

A₁ = {(a, 0.5), (b, 1.0), (c, 0.5)} and A₂ = {(a, 1.0), (b, 1.0), (c, 0.5)}

would be subsets of X.

                                  A₁ ⊆ X ,   A₂ ⊆X.

The collection of these subsets of X (including fuzzy set) is called power set P(X).

Kwang H. Lee, First Course on Fuzzy Theory and Applications, 2005, Springer, pag(7-14)