Central Limit Theorem
المؤلف:
Abramowitz, M. and Stegun, I. A.
المصدر:
Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, 1972.
الجزء والصفحة:
...
23-4-2021
3830
Central Limit Theorem
Let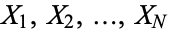 
be a set of 
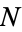independent random variates and each 
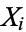have an arbitrary probability distribution 
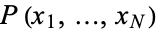with mean 
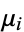and a finite variance 
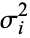. Then the normal form variate
 |
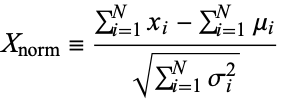(1)
|
has a limiting cumulative distribution function which approaches a normal distribution.
Under additional conditions on the distribution of the addend, the probability density itself is also normal (Feller 1971) with mean 
and variance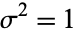 
. If conversion to normal form is not performed, then the variate
 |
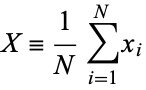(2)
|
is normally distributed with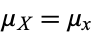 
and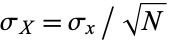 
.
Kallenberg (1997) gives a six-line proof of the central limit theorem. For an elementary, but slightly more cumbersome proof of the central limit theorem, consider the inverse Fourier transform of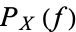 
.
Now write
 |
(7)
|
so we have
Now expand
 |
(17)
|
so
since
Taking the Fourier transform,
This is of the form
 |
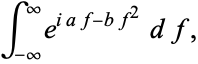(25)
|
where 
and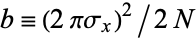 
. But this is a Fourier transform of a Gaussian function, so
 |
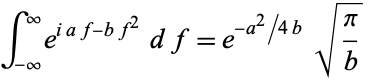(26)
|
(e.g., Abramowitz and Stegun 1972, p. 302, equation 7.4.6). Therefore,
But 
and 
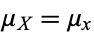, so
 |
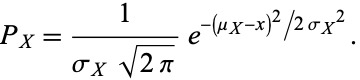(30)
|
The "fuzzy" central limit theorem says that data which are influenced by many small and unrelated random effects are approximately normally distributed.
REFERENCES:
Abramowitz, M. and Stegun, I. A. (Eds.). Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, 1972.
Feller, W. "The Fundamental Limit Theorems in Probability." Bull. Amer. Math. Soc. 51, 800-832, 1945.
Feller, W. An Introduction to Probability Theory and Its Applications, Vol. 1, 3rd ed. New York: Wiley, p. 229, 1968.
Feller, W. An Introduction to Probability Theory and Its Applications, Vol. 2, 3rd ed. New York: Wiley, 1971.
Kallenberg, O. Foundations of Modern Probability. New York: Springer-Verlag, 1997.
Lindeberg, J. W. "Eine neue Herleitung des Exponentialgesetzes in der Wahrscheinlichkeitsrechnung." Math. Z. 15, 211-225, 1922.
Spiegel, M. R. Theory and Problems of Probability and Statistics. New York: McGraw-Hill, pp. 112-113, 1992.
Trotter, H. F. "An Elementary Proof of the Central Limit Theorem." Arch. Math. 10, 226-234, 1959.
Zabell, S. L. "Alan Turing and the Central Limit Theorem." Amer. Math. Monthly 102, 483-494, 1995.
الاكثر قراءة في الاحتمالات و الاحصاء
اخر الاخبار
اخبار العتبة العباسية المقدسة
