The asymptotic form of the -step Bernoulli distribution with parameters 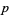 and 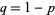 is given by

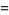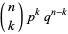			(1)
			(2)

(Papoulis 1984, p. 105).

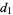Uspensky (1937) defines the de Moivre-Laplace theorem as the fact that the sum of those terms of the binomial series of  for which the number of successes  falls between  and 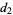 is approximately

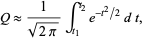(3)

where

			(4)
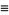			(5)
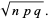			(6)

More specifically, Uspensky (1937, p. 129) showed that

(7)

where the error term satisfies

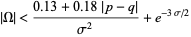(8)

for 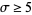 (Uspensky 1937, p. 129; Kenney and Keeping 1951, pp. 36-37). Note that Kenney and Keeping (1951, p. 37) give the slightly smaller denominator .

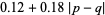A corollary states that the probability that  successes in 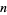 trials will differ from the expected value  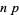by more than  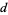is , where

(9)

with

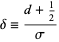(10)

(Kenney and Keeping 1951, p. 39). Uspensky (1937, p. 130) showed that 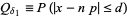 is given by

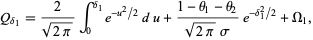(11)

where

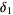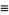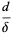			(12)
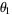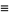			(13)
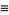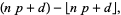			(14)

and the error term satisfies

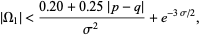(15)

for 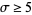 (Uspensky 1937, p. 130; Kenney and Keeping 1951, pp. 40-41).

REFERENCES:

de la Vallée-Poussin, C. "Demonstration nouvelle du théorème de Bernoulli." Ann. Soc. Sci. Bruxelles 31, 219-236, 1907.

de Moivre, A. Miscellanea analytica. Lib. 5, 1730.

de Moivre, A. The Doctrine of Chances, or, a Method of Calculating the Probabilities of Events in Play, 3rd ed. New York: Chelsea, 2000. Reprint of 1756 3rd ed. Original ed. published 1716.

Kenney, J. F. and Keeping, E. S. "The DeMoivre-Laplace Theorem" and "Simple Sampling of Attributes." §2.10 and 2.11 in Mathematics of Statistics, Pt. 2, 2nd ed. Princeton, NJ: Van Nostrand, pp. 36-41, 1951.

Laplace, P. Théorie analytiques de probabilités, 3ème éd., revue et augmentée par l'auteur. Paris: Courcier, 1820. Reprinted in Œuvres complètes de Laplace, tome 7. Paris: Gauthier-Villars, pp. 280-285, 1886.

Mirimanoff, D. "Le jeu de pile ou face et les formules de Laplace et de J. Eggenberger." Commentarii Mathematici Helvetici 2, 133-168, 1930.

Papoulis, A. Probability, Random Variables, and Stochastic Processes, 2nd ed. New York: McGraw-Hill, 1984.

Uspensky, J. V. "Approximate Evaluation of Probabilities in Bernoullian Case." Ch. 7 in Introduction to Mathematical Probability. New York: McGraw-Hill, pp. 119-138, 1937.

اخر الاخبار

اخبار العتبة العباسية المقدسة

جولة تفقدية لمتابعة خطة تنظيم مداخل المعزّين المشاركين في عزاء ركضة طويريج

لتعزيز التعاون الأكاديمي والبحثي.. جامعة الكفيل توقع اتفاقيتي توأمة مع جامعتين عراقيتين

المجمَع العلميّ يواصل إقامة مجلسه العزائي بذكرى شهادة الإمام الحسين (عليه السلام) في النجف الأشرف

قسم حفظ النظام يواصل تنفيذ خطته لتنظيم سير المواكب والزائرين في شهر المحرم

المزيد

الآخبار الصحية

لهذا السبب.. إغلاق 25 مركزًا لعلاج الإدمان في مصر ؟

خلافا للاعتقاد الشائع.. علماء يكشفون أسرار بذور البطيخ ؟!

هذا ما يحدث لجسمك عند تناولك الطماطم بانتظام !

تعرف على أطعمة تسرّع "تعافي الجسم" من حروق الشمس

المزيد

الآخبار التكنلوجية

الثلوج تغطي الصحراء الأكثر جفافا في العالم!

بتلسكوب "ألما".. التقاط صورا غير مسبوقة لبدايات الكون !

هل هاتفك مراقب؟.. 5 علامات خطيرة تكشف التجسس عليك

دبي تطلق أول رحلة تجريبية للتاكسي الجوي

المزيد