Correlation Coefficient--Bivariate Normal Distribution
المؤلف:
Bevington, P. R.
المصدر:
Data Reduction and Error Analysis for the Physical Sciences. New York: McGraw-Hill, 1969.
الجزء والصفحة:
...
27-3-2021
4112
Correlation Coefficient--Bivariate Normal Distribution
For a bivariate normal distribution, the distribution of correlation coefficients is given by
where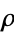 
is the population correlation coefficient, 
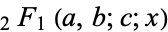is a hypergeometric function, and 
is the gamma function (Kenney and Keeping 1951, pp. 217-221). The moments are
where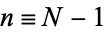 
. If the variates are uncorrelated, then 
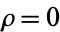and
so
But from the Legendre duplication formula,
 |
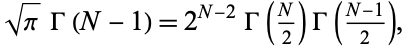(12)
|
so
The uncorrelated case can be derived more simply by letting 
be the true slope, so that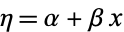 
. Then
 |
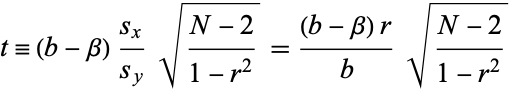(17)
|
is distributed as Student's t with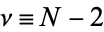 
degrees of freedom. Let the population regression coefficient 
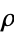be 0, then 
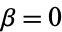, so
 |
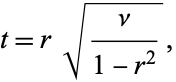(18)
|
and the distribution is
 |
(19)
|
Plugging in for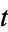 
and using
gives
so
 |
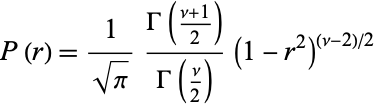(27)
|
as before. See Bevington (1969, pp. 122-123) or Pugh and Winslow (1966, §12-8). If we are interested instead in the probability that a correlation coefficient would be obtained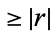 
, where 
is the observed coefficient, then
Let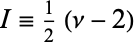 
. For even 
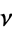, the exponent 
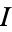is an integer so, by the binomial theorem,
 |
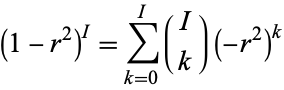(31)
|
and
For odd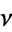 
, the integral is
Let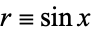 
so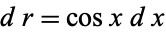 
, then
But 
is odd, so 
is even. Therefore
 |
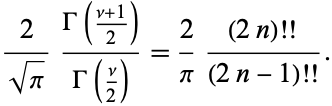(38)
|
Combining with the result from the cosine integral gives
![P_c(r)=1-2/pi((2n)!!(2n-1)!!)/((2n-1)!!(2n)!!)[sinxsum_(k=0)^(n-1)((2k)!!)/((2k+1)!!)cos^(2k+1)x+x]_0^(sin^(-1)|r|).](https://mathworld.wolfram.com/images/equations/CorrelationCoefficientBivariateNormalDistribution/NumberedEquation8.gif) |
(39)
|
Use
 |
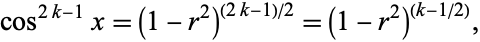(40)
|
and define 
, then
 |
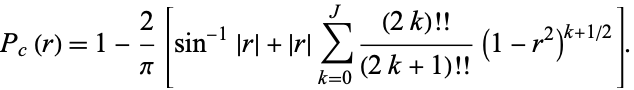(41)
|
(In Bevington 1969, this is given incorrectly.) Combining the correct solutions
 |
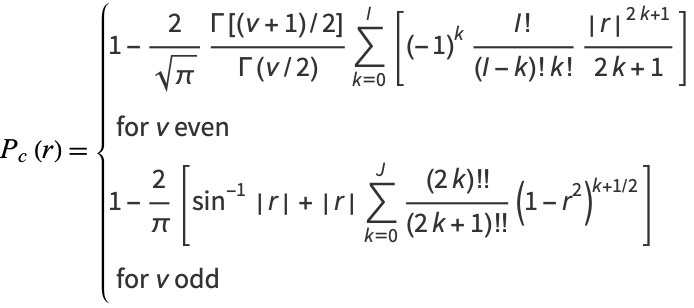(42)
|
If 
, a skew distribution is obtained, but the variable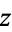 
defined by
 |
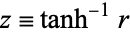(43)
|
is approximately normal with
(Kenney and Keeping 1962, p. 266).
Let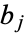 
be the slope of a best-fit line, then the multiple correlation coefficient is
 |
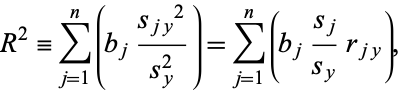(46)
|
where 
is the sample variance.
On the surface of a sphere,
 |
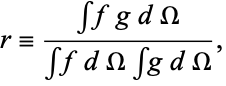(47)
|
where 
is a differential solid angle. This definition guarantees that 
. If 
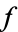and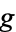 
are expanded in real spherical harmonics,
Then
 |
(50)
|
The confidence levels are then given by
where
 |
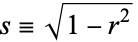(56)
|
(Eckhardt 1984).
REFERENCES:
Bevington, P. R. Data Reduction and Error Analysis for the Physical Sciences. New York: McGraw-Hill, 1969.
Eckhardt, D. H. "Correlations Between Global Features of Terrestrial Fields." Math. Geology 16, 155-171, 1984.
Kenney, J. F. and Keeping, E. S. Mathematics of Statistics, Pt. 2, 2nd ed. Princeton, NJ: Van Nostrand, 1951.
Kenney, J. F. and Keeping, E. S. Mathematics of Statistics, Pt. 1, 3rd ed. Princeton, NJ: Van Nostrand, 1962.
Pugh, E. M. and Winslow, G. H. The Analysis of Physical Measurements. Reading, MA: Addison-Wesley, 1966.
الاكثر قراءة في الاحتمالات و الاحصاء
اخر الاخبار
اخبار العتبة العباسية المقدسة
