Let the number of random walks on a 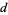-D hypercubic lattice starting at the origin which never land on the same lattice point twice in  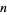steps be denoted 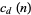. The first few values are

			(1)
			(2)
			(3)

In general,

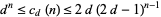(4)

(Pönitz and Tittman 2000), with tighter bounds given by Madras and Slade (1993). Conway and Guttmann (1996) have enumerated walks of up to length 51.

On any lattice, breaking a self-avoiding walk in two yields two self-avoiding walks, but concatenating two self-avoiding walks does not necessarily maintain the self-avoiding property. Let 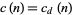 denote the number of self-avoiding walks with  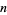steps in a lattice of  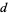dimensions. Then the above observation tells us that , and Fekete's lemma shows that

(5)

called the connective constant of the lattice, exists and is finite. The best ranges for these constants are

			(6)
			(7)
			(8)
			(9)
			(10)

(Beyer and Wells 1972, Noonan 1998, Finch 2003). The upper bound of 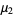 improves on the 2.6939 found by Noonan (1998) and was computed by Pönitz and Tittman (2000).

For the triangular lattice in the plane, 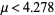 (Alm 1993), and for the hexagonal planar lattice, it is conjectured that

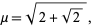(11)

(Madras and Slade 1993).

The following limits are also believed to exist and to be finite:

{lim_(n->infty)(c(n))/(mu^nn^(gamma-1)) for d!=4; lim_(n->infty)(c(n))/(mu^nn^(gamma-1)(lnn)^(1/4)) for d=4, " src="https://mathworld.wolfram.com/images/equations/Self-AvoidingWalkConnectiveConstant/NumberedEquation4.gif" style="height:88px; width:198px" />

(12)

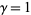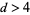where the critical exponent  for  (Madras and Slade 1993) and it has been conjectured that

{(43)/(32) for d=2; 1.162... for d=3; 1 for d=4. " src="https://mathworld.wolfram.com/images/equations/Self-AvoidingWalkConnectiveConstant/NumberedEquation5.gif" style="height:70px; width:156px" />

(13)

Define the mean square displacement over all 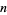-step self-avoiding walks  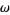as

			(14)
			(15)

The following limits are believed to exist and be finite:

{lim_(n->infty)(s(n))/(n^(2nu)) for d!=4; lim_(n->infty)(s(n))/(n^(2nu)(lnn)^(1/4)) for d=4, " src="https://mathworld.wolfram.com/images/equations/Self-AvoidingWalkConnectiveConstant/NumberedEquation6.gif" style="height:86px; width:177px" />

(16)

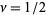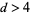where the critical exponent  for  (Madras and Slade 1993), and it has been conjectured that

{3/4 for d=2; 0.59... for d=3; 1/2 for d=4. " src="https://mathworld.wolfram.com/images/equations/Self-AvoidingWalkConnectiveConstant/NumberedEquation7.gif" style="height:78px; width:149px" />

(17)

REFERENCES:

Alm, S. E. "Upper Bounds for the Connective Constant of Self-Avoiding Walks." Combin. Probab. Comput. 2, 115-136, 1993.

Beyer, W. A. and Wells, M. B. "Lower Bound for the Connective Constant of a Self-Avoiding Walk on a Square Lattice." J. Combin. Th. A 13, 176-182, 1972.

Conway, A. R. and Guttmann, A. J. "Square Lattice Self-Avoiding Walks and Corrections to Scaling." Phys. Rev. Lett. 77, 5284-5287, 1996.

Finch, S. R. "Self-Avoiding Walk Constants." §5.10 in Mathematical Constants. Cambridge, England: Cambridge University Press, pp. 331-339, 2003.

Madras, N. and Slade, G. The Self-Avoiding Walk. Boston, MA: Birkhäuser, 1993.

Noonan, J. "New Upper Bounds for the Connective Constants of Self-Avoiding Walks." J. Stat. Phys. 91, 871-888, 1998.

Pönitz, A. and Tittman, P. "Improved Upper Bounds for Self-Avoiding Walks in 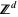." Electronic J. Combinatorics 7, No. 1, R21, 1-19, 2000. https://www.combinatorics.org/Volume_7/Abstracts/v7i1r21.html.

اخر الاخبار

اخبار العتبة العباسية المقدسة

قسم الشؤون الطبية يقدم الخدمات الصحية والعلاجية لزائري مدينة سامراء

العتبة العباسية المقدسة تقدم خدماتها لزائري مرقد الإمامين العسكريين (عليهما السلام) في سامراء

المجمَع العلمي ووفد وزارة التربية يبحثان سبل التعاون المشترك

قسم الشؤون الفكرية يصدر العدد 192 من مجلة الرياحين للأطفال

المزيد

الآخبار الصحية

كنز غذائي على طبقك.. ماذا تفعل السبانخ بجسمك؟

ما لا يتوقعه أحد.. "سر غذائي" في الجبن الدسم

لتحسين الهضم والنوم.. هذا أفضل وقت لتناول العشاء

10 طرق بسيطة وغير مألوفة لحرق المزيد من السعرات يوميا

المزيد

الآخبار التكنلوجية

سابقة بعالم الطيران.. طائرة تقرر الهبوط بنفسها بعد ظرف طارئ

"الإنفلونزا الخارقة" تكتسح دول العالم.. وتثير قلقا كبيرا

مرحلة ما قبل الأعراض.. دواء جديد يستهدف "شرارة" الزهايمر

إزالة ثاني أكسيد الكربون من الغلاف الجوي.. ما دور المحيطات والبحار؟

المزيد