The Buffon-Laplace needle problem asks to find the probability 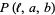 that a needle of length  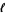will land on at least one line, given a floor with a grid of equally spaced parallel lines distances  and 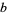 apart, with 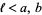. The position of the needle can be specified with points  and its orientation with coordinate 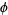. By symmetry, we can consider a single rectangle of the grid, so  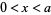and 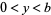. In addition, since opposite orientations are equivalent, we can take .

The probability is given by

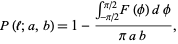(1)

where

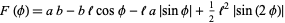(2)

(Uspensky 1937, p. 256; Solomon 1978, p. 4), giving

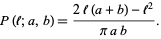(3)

This problem was first solved by Buffon (1777, pp. 100-104), but his derivation contained an error. A correct solution was given by Laplace (1812, pp. 359-362; Laplace 1820, pp. 365-369).

If  so that 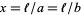 and 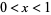, then the probabilities of a needle crossing 0, 1, and 2 lines are

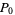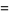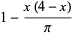			(4)
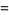			(5)
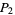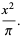			(6)

Defining 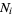 as the number of times in  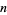tosses that a short needle crosses exactly  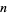lines, the variable  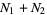has a binomial distribution with parameters  and , where  (Perlman and Wichura 1975). A point estimator for 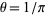 is given by

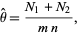(7)

which is a uniformly minimum variance unbiased estimator with variance

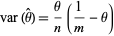(8)

(Perlman and Wishura 1975). An estimat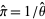or  for 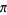 is then given by

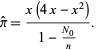(9)

This has asymptotic variance

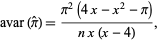(10)

which, for , becomes

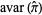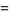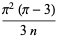			(11)
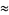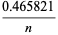			(12)

(OEIS A114602).

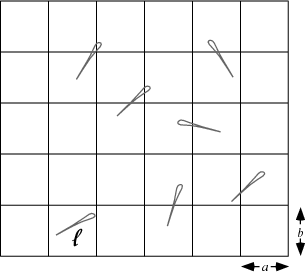
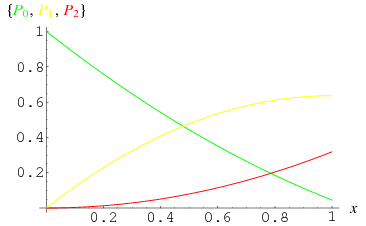
A set of sample trials is illustrated above for needles of length 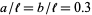, where needles intersecting 0 lines are shown in green, those intersecting a single line are shown in yellow, and those intersecting two lines are shown in red.

If the plane is instead tiled with congruent triangles with sides , , 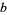, 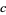and a needle with length  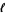less than the shortest altitude is thrown, the probability that the needle is contained entirely within one of the triangles is given by

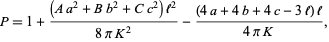(13)

where 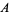, , 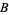and  are the angles opposite 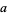, , 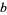and 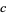, respectively, and  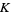is the area of the triangle. For a triangular grid consisting of equilateral triangles, this simplifies to

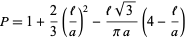(14)

(Markoff 1912, pp. 169-173; Uspensky 1937, p. 258).

REFERENCES:

Buffon, G. "Essai d'arithmétique morale." Histoire naturelle, générale er particulière, Supplément 4, 46-123, 1777.

Laplace, P. S. Théorie analytique des probabilités. Paris: Veuve Courcier, 1812.

Laplace, P. S. Théorie analytique des probabilités, 3rd rev. ed. Paris: Veuve Courcier, 1820.

Markoff, A. A. Wahrscheinlichkeitsrechnung. Leipzig, Germany: Teubner, 1912.

Perlman, M. and Wichura, M. "Sharpening Buffon's Needle." Amer. Stat. 20, 157-163, 1975.

Schuster, E. F. "Buffon's Needle Experiment." Amer. Math. Monthly 81, 26-29, 1974.

Sloane, N. J. A. Sequence A114602 in "The On-Line Encyclopedia of Integer Sequences."

Solomon, H. Geometric Probability. Philadelphia, PA: SIAM, pp. 3-6, 1978.

Uspensky, J. V. "Laplace's Problem." §12.17 in Introduction to Mathematical Probability. New York: McGraw-Hill, pp. 255-257, 1937.

اخر الاخبار

اخبار العتبة العباسية المقدسة

المجمَع العلمي يقيم مسابقة الكوثر الخاصة بترتيل القرآن الكريم في كربلاء

قسم الشؤون الفكرية والثقافية يُصدر العدد الثالث والعشرين من مجلّة (أوراق معرفية)

قسم التربية والتعليم يُطلق الموسم التدريبيّ الصيفيّ لملاكات مجموعة العميد التربوية

المجمَع العلمي يُطلق المرحلة الثالثة من مشروع أمير القرّاء الوطني

المزيد

الآخبار الصحية

اختراق علمي.. دواء يؤخر ظهور السكري من النوع الأول لسنوات

هذا ما يحدث لجسمك إن أهملت نظافة فراشك

لماذا تعتبر النساء أكثر عرضة للإصابة بألزهايمر من الرجال؟

لماذا يحذر الخبراء من الإفراط في تناول الفراولة؟

المزيد

الآخبار التكنلوجية

السيارات الكهربائية أنظف بنسبة 73% من سيارات الوقود

سيارة لوسِد الكهربائية تدخل "غينيس" بأطول مدى في العالم

نظرية جديدة تنسف كل ما نعرفه عن تشكل أول قارة في الأرض

منذ 37 ألف عام.. دراسة تكشف "أقدم أمراض" أصابت البشر

المزيد