Riemann Zeta Function zeta(2)

The value for

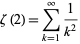(1)

can be found using a number of different techniques (Apostol 1983, Choe 1987, Giesy 1972, Holme 1970, Kimble 1987, Knopp and Schur 1918, Kortram 1996, Matsuoka 1961, Papadimitriou 1973, Simmons 1992, Stark 1969, 1970, Yaglom and Yaglom 1987).

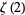 is therefore the definite sum version of the indefinite sum

			(2)
			(3)

where 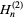 is a generalized harmonic number (whose numerator is known as a Wolstenholme number) and  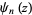is a polygamma function.

The problem of finding this value analytically is sometimes known as the Basel problem (Derbyshire 2004, pp. 63 and 370) or Basler problem (Castellanos 1988). It was first proposed by Pietro Mengoli in 1644 (Derbyshire 2004, p. 370). The solution

(4)

was first found by Euler in 1735 (Derbyshire 2004, p. 64) or 1736 (Srivastava 2000).

Yaglom and Yaglom (1987), Holme (1970), and Papadimitriou (1973) all derive the result, 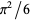 from de Moivre's identity or related identities.

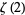 is given by the series

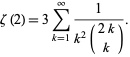(5)

(Knopp 1990, pp. 266-267), probably known to Euler and rediscovered by Apéry.

Bailey (2000) and Borwein and Bailey (2003, pp. 128-129) give a collection of BBP-type formulas that include a number for ,

			(6)
			(7)

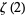 is given by the double series

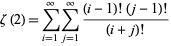(8)

(B. Cloitre, pers. comm., Dec. 9, 2004).

One derivation for 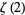 considers the Fourier series of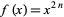 

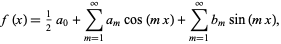(9)

which has coefficients given by

			(10)
			(11)
			(12)

where 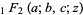 is a generalized hypergeometric function and (12) is true since the integrand is odd. Therefore, the Fourier series is given explicitly by

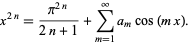(13)

If 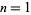, then

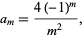(14)

so the Fourier series is

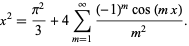(15)

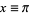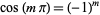Letting  gives , so

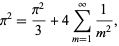(16)

and we have

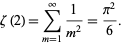(17)

Higher values of 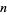 can be obtained by finding  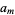and proceeding as above.

The value 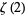 can also be found simply using the root linear coefficient theorem. Consider the equation  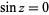and expand sin in a Maclaurin series

(18)

			(19)
			(20)

where 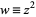. But the zeros of  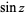occur at 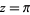, , 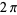, 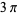..., or 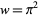, , 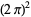.... Therefore, the sum of the roots equals the coefficient of the leading term

(21)

which can be rearranged to yield

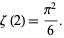(22)

Yet another derivation (Simmons 1992) evaluates 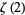 using Beukers's (1979) integral

			(23)
			(24)
			(25)
			(26)
			(27)
			(28)
			(29)

To evaluate the integral, rotate the coordinate system by 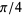 so

			(30)
			(31)

and

			(32)
			(33)

Then

			(34)
			(35)

Now compute the integrals  and .

			(36)
			(37)
			(38)

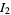Make the substitution

			(39)
			(40)
			(41)

so

(42)

and

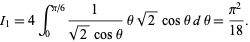(43)

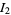 can also be computed analytically,

			(44)
			(45)
			(46)

But

			(47)
			(48)
			(49)
			(50)
			(51)

so

			(52)
			(53)
			(54)

Combining  and 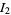 gives

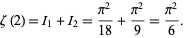(55)

REFERENCES:

Apostol, T. M. "A Proof That Euler Missed: Evaluating  the Easy Way." Math. Intel. 5, 59-60, 1983.

Bailey, D. H. "A Compendium of BBP-Type Formulas for Mathematical Constants." 28 Nov 2000. http://crd.lbl.gov/~dhbailey/dhbpapers/bbp-formulas.pdf.

Beukers, F. "A Note on the Irrationality of 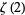 and 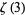." Bull. London Math. Soc. 11, 268-272, 1979.

Borwein, J. and Bailey, D. Mathematics by Experiment: Plausible Reasoning in the 21st Century. Wellesley, MA: A K Peters, pp. 89-90, 2003.

Castellanos, D. "The Ubiquitous Pi. Part I." Math. Mag. 61, 67-98, 1988.

Choe, B. R. "An Elementary Proof of 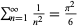." Amer. Math. Monthly 94, 662-663, 1987.

Derbyshire, J. Prime Obsession: Bernhard Riemann and the Greatest Unsolved Problem in Mathematics. New York: Penguin, 2004.

Giesy, D. P. "Still Another Proof That 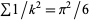." Math. Mag. 45, 148-149, 1972.

Havil, J. Gamma: Exploring Euler's Constant. Princeton, NJ: Princeton University Press, pp. 37-40, 2003.

Holme, F. "Ein enkel beregning av 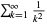." Nordisk Mat. Tidskr. 18, 91-92 and 120, 1970.

Kimble, G. "Euler's Other Proof." Math. Mag. 60, 282, 1987.

Knopp, K. Theory and Application of Infinite Series. New York: Dover, 1990.

Knopp, K. and Schur, I. "Über die Herleitug der Gleichung ." Archiv der Mathematik u. Physik 27, 174-176, 1918.

Kortram, R. A. "Simple Proofs for 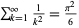 and ." Math. Mag. 69, 122-125, 1996.

Matsuoka, Y. "An Elementary Proof of the Formula 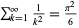." Amer. Math. Monthly 68, 486-487, 1961.

Papadimitriou, I. "A Simple Proof of the Formula 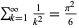." Amer. Math. Monthly 80, 424-425, 1973.

Simmons, G. F. "Euler's Formula 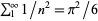 by Double Integration." Ch. B. 24 in Calculus Gems: Brief Lives and Memorable Mathematics. New York: McGraw-Hill, 1992.

Spiess, O. "Die Summe der reziproken Quadratzahlen." In Festschrift zum 60 Geburtstag von Dr. Andreas Speiser (Ed. L. V. Ahlfors et al. ). Zürich: Füssli, pp. 66-86, 1945.

Srivastava, H. M. "Some Simple Algorithms for the Evaluations and Representations of the Riemann Zeta Function at Positive Integer Arguments." J. Math. Anal. Appl. 246, 331-351, 2000.

Stark, E. L. "Another Proof of the Formula 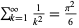." Amer. Math. Monthly 76, 552-553, 1969.

Stark, E. L. "." Praxis Math. 12, 1-3, 1970.

Wells, D. The Penguin Dictionary of Curious and Interesting Numbers. Middlesex, England: Penguin Books, p. 40, 1986.

Yaglom, A. M. and Yaglom, I. M. Problem 145 in Challenging Mathematical Problems with Elementary Solutions, Vol. 2. New York: Dover, 1987.