Gosper,s Algorithm
المؤلف:
Gessel, I. and Stanton, D.
المصدر:
"Strange Evaluations of Hypergeometric Series." SIAM J. Math. Anal. 13
الجزء والصفحة:
...
20-6-2019
3613
Gosper's Algorithm
An algorithm for finding closed form hypergeometric identities. The algorithm treats sums whose successive terms have ratios which are rational functions. Not only does it decide conclusively whether there exists a hypergeometric sequence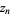 
such that
 |
(1)
|
but actually produces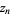 
if it exists. If not, it produces 
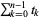. An outline of the algorithm follows (Petkovšek et al. 1996):
1. For the ratio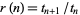 
which is a rational function of 
.
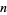2. Write
 |
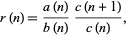(2)
|
where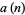 
, 
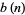, and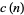 
are polynomials satisfying
 |
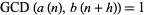(3)
|
for all nonnegative integers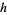 
.
3. Find a nonzero polynomial solution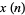 
of
 |
(4)
|
if one exists.
4. Return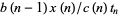 
and stop.
Petkovšek et al. (1996) describe the algorithm as "one of the landmarks in the history of computerization of the problem of closed form summation." Gosper's algorithm is vital in the operation of Zeilberger's algorithm and the machinery of Wilf-Zeilberger pairs.
REFERENCES:
Gessel, I. and Stanton, D. "Strange Evaluations of Hypergeometric Series." SIAM J. Math. Anal. 13, 295-308, 1982.
Gosper, R. W. "Decision Procedure for Indefinite Hypergeometric Summation." Proc. Nat. Acad. Sci. USA 75, 40-42, 1978.
Graham, R. L.; Knuth, D. E.; and Patashnik, O. Concrete Mathematics: A Foundation for Computer Science, 2nd ed. Reading, MA: Addison-Wesley, 1994.
Koepf, W. "Algorithms for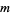 
-fold Hypergeometric Summation." J. Symb. Comput. 20, 399-417, 1995.
Koepf, W. "Gosper's Algorithm." Ch. 5 in Hypergeometric Summation: An Algorithmic Approach to Summation and Special Function Identities. Braunschweig, Germany: Vieweg, pp. 61-79, 1998.
Lafron, J. C. "Summation in Finite Terms." In Computer Algebra Symbolic and Algebraic Computation, 2nd ed. (Ed. B. Buchberger, G. E. Collins, and R. Loos). New York: Springer-Verlag, 1983.
Paule, P. and Schorn, M. "A Mathematica Version of Zeilberger's Algorithm for Proving Binomial Coefficient Identities." J. Symb. Comput. 20, 673-698, 1995.
Petkovšek, M.; Wilf, H. S.; and Zeilberger, D. "Gosper's Algorithm." Ch. 5 in A=B. Wellesley, MA: A K Peters, pp. 73-99, 1996. http://www.cis.upenn.edu/~wilf/AeqB.html.
Pirastu, R. and Strehl, V. "Rational Summation and Gosper-Petkovšek Representation." J. Symb. Comput. 20, 617-635, 1995.
Zeilberger, D. "The Method of Creative Telescoping." J. Symb. Comput. 11, 195-204, 1991.
الاكثر قراءة في التفاضل و التكامل
اخر الاخبار
اخبار العتبة العباسية المقدسة
