To divide means to split up a large number into smaller groups of numbers. For example, a group of 50 people could be divided into smaller groups of people, such as 5 groups of 10. In order to do basic division, it is very important that you know your multiplication tables (or times tables).

For example, you might see a problem that looks like this:

This problem would be read, "Eighteen divided by 2 equals _____." In order to solve this problem, you would need to think of your times tables. Then, you would turn the problem around in your head, and say "What number times 2 equals 18?" or "How many times can we put 2 into 18?"

After thinking about your two's times tables, you would remember that 9 x 2 = 18. Thus, your answer to the division problem is 9. Notice that these can be said in two different ways:

Eighteen divided by two equals nine.
Two times nine equals eighteen.

Let's try another one. Our new problem is:

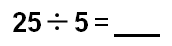
This is read "twenty-five divided by five equals ____." Now, we have to flip it around and say, "What times 5 gives us 25?" We think back to our times tables and remember that 5 x 5 = 25, therefore 25 divided by 5 equals 5. Our final answer is five.

Notice that these can also be said opposite of each other:

• Twenty-five divided by five equals five.
• Five times five equals twenty-five.

Division problems like this can often be done in your head, so there’s no real work to show for solving them. This is called using mental math—because it’s done in your mind. For examples of how to do harder division problems, see our page on long division.

Long Division

When starting division, you may be given simple division problems that you can do in your head, using mental math. Those problems would look like this:

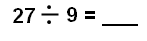
You would think to yourself, what number times 9 gives me 27? And your answer would be 3. However, eventually you will encounter bigger division problems that you cannot do using mental math. In these cases, you will have to use long division.

For example, you might have a problem that looks like this:

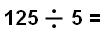
You would re-write the problem so that it looks like this:

In this case, 5 is the divisor (the number we’re dividing by) and it goes on the outside of the division bar, as shown. 125 is the dividend (the number we’re dividing) and it goes on the inside of the division bar. The quotient (answer) will eventually sit on top of the division bar, when we’re done. Right now, the top of the division bar should be blank because we have not started yet.

Now, we can start our long division. There are four steps of long division; they are: divide, multiply, subtract, and bring down. Each step will be explained and shown in a different color in the step-by-step image.

Our first step of long division is to divide. In this step, we have to ask ourselves how many times the divisor goes into the first number of the dividend; or, in this case, we ask ourselves how many times we can put 5 into 1. You will notice that we cannot put 5 into 1, because 5 is bigger than one; thus, our first division results in 0. We write this number on top of the division bar, above the number we used (in this case, 1). Your problem so far looks like this:

Our next step of long division is to multiply. In this step, we multiply the divisor (5) by the answer we got to our division (in this case, 0). We multiply the two numbers together like this: 5 x 0 = 0. We write this number below the dividend, lining it up with the number we divided.

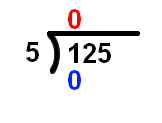
Our next step of long division is to subtract. In this step, we subtract our product (answer) from multiplication from the original number in the dividend. In this case, our problem would be 1 – 0 = 1. We would write the answer in the column we’ve now made (see diagram below).

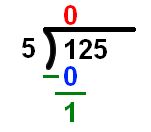
Now we move on to our last step, which is to bring down. In order to bring down, we have to look at the next number in the dividend that we haven’t worked with yet; in this case, it’s 2. In order to bring down, we draw an arrow from the number in the dividend down to where we just ended our subtraction, and we write this number (2) next to the answer from our subtraction (1) to form a new number (12). This is shown in the diagram below.

Once you bring down the next number, you start this entire process over with division! In the image below, you’ll see the next set of steps performed, starting with this division question: how many times can we put 5 into 12? Follow along with the diagrams:

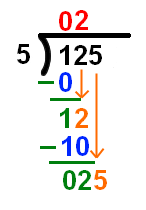
That was a complete step (division, multiplication, subtraction, and bringing down) that we just went through! We keep repeating the process until there are no more numbers to bring down. In this problem, we have one more complete step to go through before we get our answer. Here’s how to go through the last step:

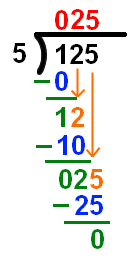
Notice that when you went to bring down, there were no other numbers after the 5, so you had nothing to bring down. This means you are done! Your answer is the number that you have written on top of the division bar. For this problem, our answer is 25, and it is written in red on top of our division bar.

Some people like to have a way to remember the steps to long division, so they’ve come up with a saying to help you remember the order. The order is: Divide, Multiply, Subtract, Bring down. The saying is: Does McDonald’s Sell Burgers? The first letters of this saying match up with the first letters of the order of long division: D-M-S-B. If this helps you, feel free to use it to remember; if it confuses you, then don’t use it—just memorize the steps for long division.

Long Division Examples

Let’s go through one more example like this before we move on. Our new example is:

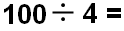
Let’s re-write the problem using the long division bar, and then follow the steps to long division (divide-multiply-subtract-bring down). Re-read the steps to the first problem if you’re still having trouble. Here’s the problem worked out:

Once again, our answer (quotient) is written above the division bar. Ours is written in red. Both of these problems had quotients of 25, but this will not always be the case! You could have any number as your quotient for a division problem.

When we have an answer for our division problem, it is easy to go back and check it. In order to check a division problem, you multiply the quotient (answer) by the divisor, and your product (answer to the multiplication problem) should be the same as the dividend.

Here’s the work for checking the last division problem:

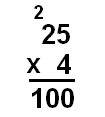
We can see that our product, 100, is the same as the dividend, so we know we did our division correctly.

Now, here's one for you to try to make sure you get the hang of it!

Dividing by 10, 100, and 1,000

Dividing by 10, 100, and 1,000 can be done using long division, like we’ve shown, or you can take a quick short cut to make life a little easier. There are several easy steps you can follow to use this short cut. First, start with a problem that looks like this:

Next, count the number of zeroes in the divisor. In this case, the divisor is 10, so we can count 1 zero. Be aware that the decimal place of 38 is after the 8, so it can be re-written like this: 38. Then, you’re going to move the decimal place of the dividend (38.) to the left the same number as the number of zeroes you counted earlier (so in this problem, 1).

Thus, your new answers is 3.8.

Let's try it one more time.

Now, count the number of zeroes in the divisor. There are 2 zeroes in 100. This means that you will move the decimal place after 489. two spaces to the left, like this:

Thus, your answer is 4.89.

Division with Decimals

Another type of division you’ll encounter is division with decimals in both the divisor and the dividend. It might look something like this:

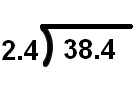
In this situation, you move the decimal place the number of spaces in the divisor until the decimal is at the end of the number; you move the decimal the same number of spaces in the dividend: this does NOT necessarily mean the decimal will land at the end of the dividend. Here’s an example:

Your new problem looks like this (note the change in decimal places in both the divisor and dividend):

Now you continue to work the problem out, remembering to bring your decimal up into your quotient at the appropriate time (it will be in red in the diagram).

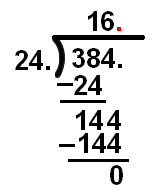
Thus, your final answer is simply 16.

Let’s try one more example of moving the decimal over in order to solve the problem.

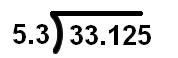
becomes

After the decimals are moved, it looks like this:

After you move the decimals, continue the problem, like this:

Thus, your final answer is 6.25.

Long Division with Remainders

We’ve already practiced long division, but so far our answers have all come out even (in other words, our last subtraction problem ended in an answer of 0). However, sometimes our division problems will not come out evenly, and we will have another number (not 0) when we do the last subtraction problem. This leftover number is called a remainder, and it is written as part of the quotient. Follow along with this example:

The red circled number at the bottom our remainder. You do not have to circle the remainder; we just circled ours so that you know which number it is. After you have your remainder, you write it on top of the division bar, with an r in front of it, like this: 25 r 3.

When your division ends with a remainder, you must make sure that your remainder is less than your divisor. If your remainder is more than your divisor, you need to go back and check your division, because it is incorrect. We can still use our multiplication method to check our division; you will multiply the quotient (25) by the divisor (5), and then add our remainder to the answer to the multiplication problem, like this:

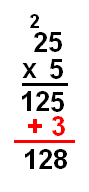
Let’s try that one more time. Here’s a new example:

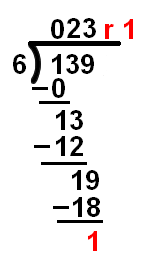
Our answer to this problem is 23 r 1; note that we always write the remainder after the quotient, on top of the division bar. Also notice that our remainder (1) is smaller than our divisor (6).

Now let’s check our work, like this:

There are also several different ways to write remainders. The standard way is shown above, with an r in front of the number. However, you can also write remainders as fractions and as decimals.

Long Division with Remainders as Fractions

Now that you understand the basics of long division, you may be asked to write your remainder as a fraction. Don’t worry! It’s not hard at all. You’re going to do long division the same way—divide, multiply, subtract, bring down, and then you’re going to get a remainder. Instead of writing r and then the number, you are going to take your remainder and make it the numerator of a fraction. The denominator comes from the divisor—you use the same number you’re dividing by in your denominator.

Let’s look at the following example:

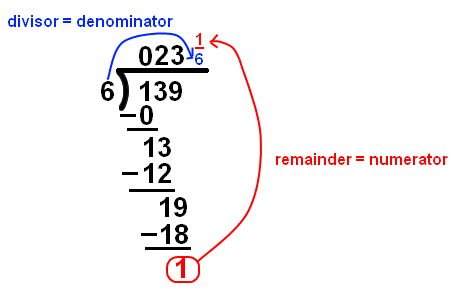
Notice that you do not use the r at all in front of your remainder when you’re turning it into a fraction. However, you do still write the fraction as part of the quotient (answer to your division problem).

Also, you would check this division problem the same way as a normal division problem; multiply the quotient (23) by the divisor (6) and then add the remainder (1). Do not do anything with the fraction in order to check this problem.

Long Division with Remainders as Decimals

Another way you may be asked to express a remainder is in the form of a decimal. When you’re asked to express your remainder as a decimal, you first complete division as usual, until you get to the point you usually end at, where you have nothing else to bring down. Instead of stopping here, however, you are going to keep going with division. You will add a decimal point (.) after the last number given in the dividend, and you will also place a decimal point in the quotient after the number you have so far. After the decimal in the dividend, you will add a zero (0) and continue division. You will keep adding zeroes until your subtraction step results in an answer of 0 as well. Follow along with this example:

Notice that we added a decimal after the 6 in the dividend, as well as a decimal after the 5 in our quotient. Then, we started adding zeroes to the dividend. This time, it only took us one added zero before our remainder was zero.

Now, let’s look at a problem where you’d have to add more than one zero to the dividend:

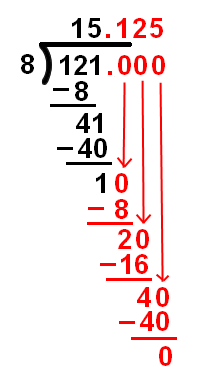
When you have your quotient with a decimal, you check the answer differently than if it had a remainder as a fraction or just a remainder written with r. Instead of adding the remainder separately, you just multiply the quotient (including decimal) by the divisor, like this:

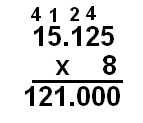
www.wyzant.com