المرجع الألكتروني للمعلوماتية
القرآن الكريم وعلومه
العقائد الإسلامية
الفقه وأصوله 
الرجال والحديث 
سيرة الرسول وآله 
علوم اللغة العربية 
الأدب العربي 
الأسرة والمجتمع 
الأخلاق والأدعية 
التاريخ 
الادارة والاقتصاد 
علم الفيزياء 
علم الكيمياء 
علم الأحياء 
الرياضيات 
الهندسة المدنية 
الزراعة 
الجغرافية 
القانون 
اللغة الأنكليزية 
الأخبار 
الإعلام 
مكتبة الصور 
أقلام بمختلف الألوان 
إضاءات
مفتاح
أجوبة الإستفتاءات الشرعية
وثائقيات
تاريخ الرياضيات
الرياضيات في الحضارات المختلفة
الرياضيات المتقطعة
الجبر
الهندسة
المعادلات التفاضلية و التكاملية
التحليل
علماء الرياضيات | Fox H-Function |
 1537
 06:06 مساءً
date: 15-6-2019 |
Author : Al-Musallam, F. A. and Tuan, V. K 
Book or Source : "H-Function with Complex Parameters I: Existence." Int. J. Math. Math. Sci. 25, 
Page and Part : ...|
Read More
Date: 20-6-2019
1369
Date: 19-9-2018
1577
Date: 2-10-2019
1210
|
The Fox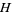 
-function is a very general function defined by
![H(z)=H_(p,q)^(m,n)[z|(a_1,alpha_1),...,(a_p,alpha_p); (b_1,beta_1),...,(b_p,beta_p)]
=1/(2pii)int_C(product_(j=1)^(m)Gamma(b_j-beta_is)product_(j=1)^(n)Gamma(1-a_j+alpha_js))/(product_(j=n+1)^(p)Gamma(a_j+alpha_js)product_(j=m+1)^(q)Gamma(1-b_j+beta_js))z^sds,](http://mathworld.wolfram.com/images/equations/FoxH-Function/NumberedEquation1.gif) |
where 
, 
, 
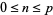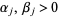, and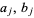 
are complex numbers such that no pole of 
for 
, 2, ...,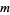 
coincides with any pole of 
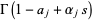for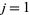 
, 2, ..., 
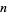(Prudnikov et al. 1990, p. 626). In addition 
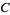, is a contour in the complex 
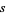-plane from 
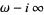to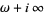 
such that 
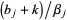and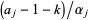 
lie to the right and left of 
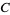, respectively.
A. Kilbas has derived a complete description for the asymptotic expansion of the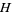 
-function.
Special cases of the Fox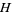 
-function include
![H_(2P+1)^1(z^i,c|(0,i); (1-a_j,i)_(P+1),(b_j-i)_P)=(_(p+1)F_P((a)_(P+1);(b)_P;(-1)^Pz))/(isgn(I[z])product_(j=1)^(P+1)Gamma(1-a_j)product_(j=1)^(P)Gamma(b)_j),](http://mathworld.wolfram.com/images/equations/FoxH-Function/NumberedEquation2.gif) |
for 
, ..., 
, 
, ..., 
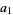complex number such that ![sum_(j=1)^(P+1)R[a_j]<sum_(j=1)^(P)Re[b_j]](http://mathworld.wolfram.com/images/equations/FoxH-Function/Inline25.gif)
, 
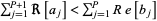, ![sgn(c)=sgn(I[z])](http://mathworld.wolfram.com/images/equations/FoxH-Function/Inline27.gif)
, and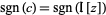 
is a generalized hypergeometric function (Al-Musallam et al. 2001b).
REFERENCES:
Al-Musallam, F. A. and Tuan, V. K. "
-Function with Complex Parameters I: Existence." Int. J. Math. Math. Sci. 25, 571-586, 2001a.
Al-Musallam, F. A. and Tuan, V. K. "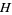
-Function with Complex Parameters II: Evaluation." Int. J. Math. Math. Sci. 25, 727-743, 2001b.
Buschman, R. G. "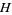
-Functions of Two Variables, I." Indian J. Math. 20, 139-153, 1978.
Buschman, R. G. "Analytic Domains for Multivariable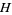 
-Functions." Pure Appl. Math. Sci. 16, 23-27, 1982.
Carter, B. D. and Springer, M. D. "The Distribution of Products, Quotients, and Powers of Independent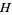 
-Functions." SIAM J. Appl. Math. 33, 542-558, 1977.
Fox, C. "The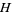 
and 
-Functions as Symmetrical Fourier Kernels." Trans. Amer. Math. Soc. 98, 395-429, 1961.
Hai, N.; Marichev, O. I.; and Buschman, R. G. "Theory of the General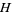 
-Function of Two Variables." Rocky Mtn. J. Math. 22, 1317-1327, 1992.
Mathai, A. M. and Saxena, R. K. The 
-Function with Applications in Statistics and Other Disciplines.0470263806 New Delhi, India: Wiley, 1978.
Prudnikov, A. P.; Brychkov, Yu. A.; and Marichev, O. I. "Evaluation of Integrals and the Mellin Transform." Itogi Nauki i Tekhniki, Seriya Matemat. Analiz 27, 3-146, 1989.
Prudnikov, A. P.; Marichev, O. I.; and Brychkov, Yu. A. "The Fox 
-Function ![H_(pq)^(mn)[z|[a_p,A_p]; [b_p,B_p]]](http://mathworld.wolfram.com/images/equations/FoxH-Function/Inline39.gif)
." §8.3 in Integrals and Series, Vol. 3: More Special Functions. Newark, NJ: Gordon and Breach, pp. 626-629, 1990.
Srivastava, H. M.; Gupta, K. C.; and Goyal, S. P. The 
-Function of One and Two Variables with Applications. New Delhi, India: South Asian Publ., 1982.
Yakubovich, S. B. and Luchko, Y. F. The Hypergeometric Approach to Integral Transforms and Convolutions. Amsterdam, Netherlands: Kluwer, 1994.
|
|
|
|
إدارة الغذاء والدواء الأميركية تقرّ عقارا جديدا للألزهايمر
|
|
|
|
|
|
|
شراء وقود الطائرات المستدام.. "الدفع" من جيب المسافر
|
|
|
|
|
|
|
بالصور: الامين العام للعتبة الحسينية المقدسة يجري جولة ميدانية للوقوف على آخر الاستعدادات الخاصة بمراسيم تبديل راية الإمام الحسين (ع)
|
|
|
