Wigner 3j-Symbol

The Wigner 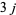-symbols 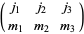, also known as " 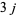symbols" (Messiah 1962, p. 1056) or Wigner coefficients (Shore and Menzel 1968, p. 275) are quantities that arise in considering coupled angular momenta in two quantum systems.

They are returned by the Wolfram Language function ThreeJSymbol[j1, m1, j2, m2, j3, m3].

The parameters of the 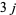 symbol  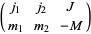(where  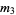has been written as 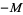) are either integers or half-integers. Additionally, they satisfy the follow selection rules (Messiah 1962, pp. 1054-1056; Shore and Menzel 1968, p. 272).

1. , , and .

2. .

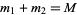3. The triangular inequalities .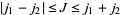

4. Integer perimeter rule: 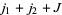 is an integer.

Note that not all these rules are independent, since rule (4) is implied by the other three. If these conditions are not satisfied, .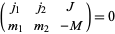

The Wigner 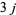-symbols have the symmetries

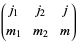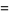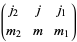			(1)
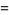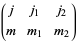			(2)
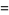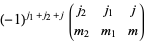			(3)
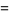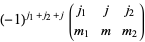			(4)
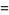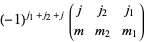			(5)
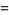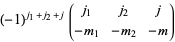			(6)

(Messiah 1962, p. 1056).

The 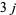-symbols can be computed using the Racah formula

(7)

where 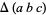 is a triangle coefficient,

(8)

and the sum is over all integers 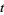 for which the factorials in  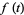all have nonnegative arguments (Messiah 1962, p. 1058; Shore and Menzel 1968, p. 273). In particular, the number of terms is equal to , where  is the smallest of the nine numbers

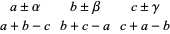(9)

(Messiah 1962, p. 1058).

The symbols obey the orthogonality relations

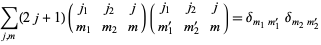(10)

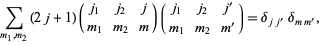(11)

where  is the Kronecker delta.

General formulas are very complicated, but some specific cases are

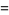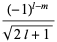			(12)
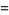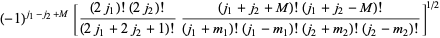			(13)
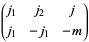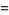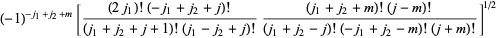			(14)
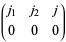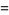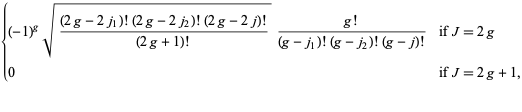			(15)

for  (Condon and Shortley 1951, pp. 76-77; Messiah 1962, pp. 1058-1060; Shore and Menzel 1968, p. 275; Abramowitz and Stegun 1972, pp. 1006-1010).

For spherical harmonics ,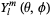

(16)

For values of  obeying the triangle condition ,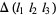

(17)

and

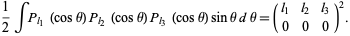(18)

They can be expressed using the related Clebsch-Gordan coefficients 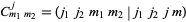 (Condon and Shortley 1951, pp. 74-75; Wigner 1959, p. 206), or Racah V-coefficients .

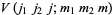Connections among the Wigner 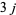-, Clebsch-Gordan, and Racah 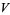-symbols are given by

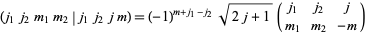(19)

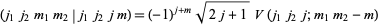(20)

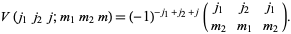(21)

REFERENCES:

Abramowitz, M. and Stegun, I. A. (Eds.). "Vector-Addition Coefficients." §27.9 in Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, pp. 1006-1010, 1972.

Biedenharn, L. C. and Louck, J. D. The Racah-Wigner Algebra in Quantum Theory. Reading, MA: Addison-Wesley, 1981.

Biedenharn, L. C. and Louck, J. D. Angular Momentum in Quantum Physics: Theory and Applications. Reading, MA: Addison-Wesley, 1981.

Condon, E. U. and Shortley, G. The Theory of Atomic Spectra. Cambridge, England: Cambridge University Press, 1951.

de Shalit, A. and Talmi, I. Nuclear Shell Theory. New York: Academic Press, 1963.

Edmonds, A. R. Angular Momentum in Quantum Mechanics, 2nd ed., rev. printing. Princeton, NJ: Princeton University Press, 1968.

Gordy, W. and Cook, R. L. Microwave Molecular Spectra, 3rd ed. New York: Wiley, pp. 804-811, 1984.

Messiah, A. "Clebsch-Gordan (C.-G.) Coefficients and '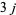' Symbols." Appendix C.I in Quantum Mechanics, Vol. 2. Amsterdam, Netherlands: North-Holland, pp. 1054-1060, 1962.

Racah, G. "Theory of Complex Spectra. II." Phys. Rev. 62, 438-462, 1942.

Rose, M. E. Elementary Theory of Angular Momentum. New York: Dover, 1995.

Rotenberg, M.; Bivens, R.; Metropolis, N.; and Wooten, J. K. The 3j and 6j Symbols. Cambridge, MA: MIT Press, 1959.

Shore, B. W. and Menzel, D. H. Principles of Atomic Spectra. New York: Wiley, pp. 275-276, 1968.

Sobel'man, I. I. "Angular Momenta." Ch. 4 in Atomic Spectra and Radiative Transitions, 2nd ed. Berlin: Springer-Verlag, 1992.

Wigner, E. P. Group Theory and Its Application to the Quantum Mechanics of Atomic Spectra, expanded and improved ed. New York: Academic Press, 1959.