

تاريخ الرياضيات

الاعداد و نظريتها

تاريخ التحليل

تار يخ الجبر

الهندسة و التبلوجي


الرياضيات في الحضارات المختلفة

العربية

اليونانية

البابلية

الصينية

المايا

المصرية

الهندية


الرياضيات المتقطعة

المنطق

اسس الرياضيات

فلسفة الرياضيات

مواضيع عامة في المنطق


الجبر

الجبر الخطي

الجبر المجرد

الجبر البولياني

مواضيع عامة في الجبر

الضبابية

نظرية المجموعات

نظرية الزمر

نظرية الحلقات والحقول

نظرية الاعداد

نظرية الفئات

حساب المتجهات

المتتاليات-المتسلسلات

المصفوفات و نظريتها

المثلثات


الهندسة

الهندسة المستوية

الهندسة غير المستوية

مواضيع عامة في الهندسة

التفاضل و التكامل


المعادلات التفاضلية و التكاملية

معادلات تفاضلية

معادلات تكاملية

مواضيع عامة في المعادلات


التحليل

التحليل العددي

التحليل العقدي

التحليل الدالي

مواضيع عامة في التحليل

التحليل الحقيقي

التبلوجيا

نظرية الالعاب

الاحتمالات و الاحصاء

نظرية التحكم

بحوث العمليات

نظرية الكم

الشفرات

الرياضيات التطبيقية

نظريات ومبرهنات


علماء الرياضيات

500AD

500-1499

1000to1499

1500to1599

1600to1649

1650to1699

1700to1749

1750to1779

1780to1799

1800to1819

1820to1829

1830to1839

1840to1849

1850to1859

1860to1864

1865to1869

1870to1874

1875to1879

1880to1884

1885to1889

1890to1894

1895to1899

1900to1904

1905to1909

1910to1914

1915to1919

1920to1924

1925to1929

1930to1939

1940to the present

علماء الرياضيات

الرياضيات في العلوم الاخرى

بحوث و اطاريح جامعية

هل تعلم

طرائق التدريس

الرياضيات العامة

نظرية البيان
Curvature
المؤلف:
Casey, J
المصدر:
Exploring Curvature. Wiesbaden, Germany: Vieweg, 1996.
الجزء والصفحة:
...
29-9-2018
3447
Curvature
In general, there are two important types of curvature: extrinsic curvature and intrinsic curvature. The extrinsic curvature of curves in two- and three-space was the first type of curvature to be studied historically, culminating in the Frenet formulas, which describe a space curve entirely in terms of its "curvature," torsion, and the initial starting point and direction.
After the curvature of two- and three-dimensional curves was studied, attention turned to the curvature of surfaces in three-space. The main curvatures that emerged from this scrutiny are the mean curvature, Gaussian curvature, and the shape operator. Mean curvature was the most important for applications at the time and was the most studied, but Gauss was the first to recognize the importance of the Gaussian curvature.
Because Gaussian curvature is "intrinsic," it is detectable to two-dimensional "inhabitants" of the surface, whereas mean curvature and the shape operator are not detectable to someone who can't study the three-dimensional space surrounding the surface on which he resides. The importance of Gaussian curvature to an inhabitant is that it controls the surface area of spheres around the inhabitant.
Riemann and many others generalized the concept of curvature to sectional curvature, scalar curvature, the Riemann tensor, Ricci curvature tensor, and a host of other intrinsic and extrinsic curvatures. General curvatures no longer need to be numbers, and can take the form of a map, group, groupoid, tensor field, etc.
The simplest form of curvature and that usually first encountered in calculus is an extrinsic curvature. In two dimensions, let a plane curve be given by Cartesian parametric equations 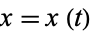 and
and 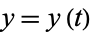 . Then the curvature
. Then the curvature  , sometimes also called the "first curvature" (Kreyszig 1991, p. 47), is defined by
, sometimes also called the "first curvature" (Kreyszig 1991, p. 47), is defined by
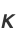 |
 |
 |
(1) |
 |
 |
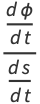 |
(2) |
 |
 |
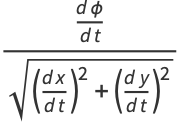 |
(3) |
 |
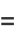 |
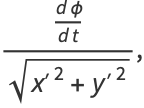 |
(4) |
where 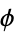 is the tangential angle and
is the tangential angle and  is the arc length. As can readily be seen from the definition, curvature therefore has units of inverse distance. The
is the arc length. As can readily be seen from the definition, curvature therefore has units of inverse distance. The 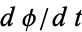 derivative in the above equation can be found using the identity
derivative in the above equation can be found using the identity
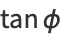 |
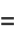 |
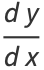 |
(5) |
 |
 |
 |
(6) |
 |
 |
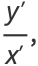 |
(7) |
so
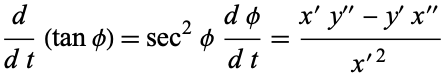 |
(8) |
and
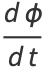 |
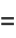 |
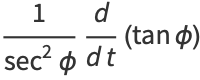 |
(9) |
 |
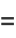 |
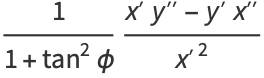 |
(10) |
 |
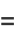 |
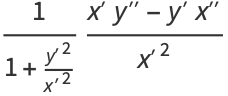 |
(11) |
 |
 |
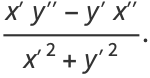 |
(12) |
Combining equations (◇), 3), (10), and (12) then gives
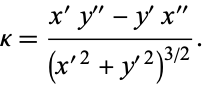 |
(13) |
For a two-dimensional curve written in the form 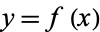 , the equation of curvature becomes
, the equation of curvature becomes
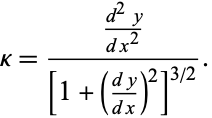 |
(14) |
If the two-dimensional curve is instead parameterized in polar coordinates, then
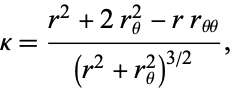 |
(15) |
where  (Gray 1997, p. 89). In pedal coordinates, the curvature is given by
(Gray 1997, p. 89). In pedal coordinates, the curvature is given by
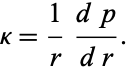 |
(16) |
The curvature for a two-dimensional curve given implicitly by  is given by
is given by
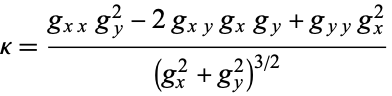 |
(17) |
(Gray 1997).
Now consider a parameterized space curve 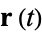 in three dimensions for which the tangent vector
in three dimensions for which the tangent vector 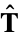 is defined as
is defined as
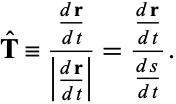 |
(18) |
Therefore,
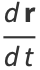 |
 |
 |
(19) |
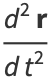 |
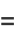 |
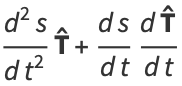 |
(20) |
 |
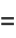 |
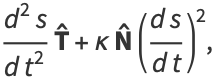 |
(21) |
where 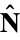 is the normal vector. But
is the normal vector. But
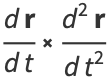 |
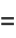 |
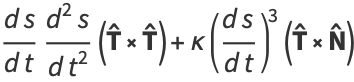 |
(22) |
 |
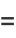 |
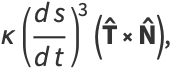 |
(23) |
so taking norms of both sides gives
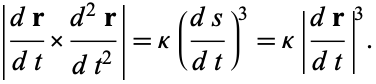 |
(24) |
Solving for  then gives
then gives
 |
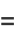 |
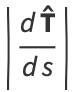 |
(25) |
 |
 |
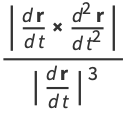 |
(26) |
 |
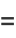 |
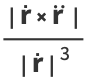 |
(27) |
(Gray 1997, p. 192).
The curvature of a two-dimensional curve is related to the radius of curvature of the curve's osculating circle. Consider a circlespecified parametrically by
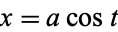 |
(28) |
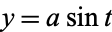 |
(29) |
which is tangent to the curve at a given point. The curvature is then
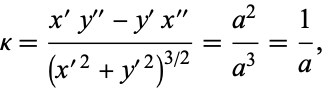 |
(30) |
or one over the radius of curvature. The curvature of a circle can also be repeated in vector notation. For the circle with 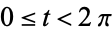 , the arc length is
, the arc length is
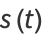 |
 |
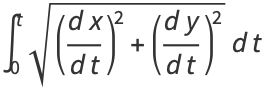 |
(31) |
 |
 |
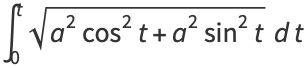 |
(32) |
 |
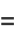 |
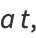 |
(33) |
so 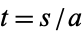 and the equations of the circle can be rewritten as
and the equations of the circle can be rewritten as
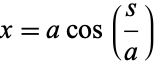 |
(34) |
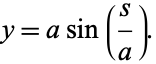 |
(35) |
The radius vector is then given by
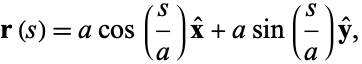 |
(36) |
and the tangent vector is
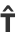 |
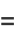 |
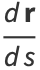 |
(37) |
 |
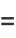 |
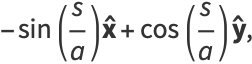 |
(38) |
so the curvature is related to the radius of curvature  by
by
 |
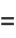 |
 |
(39) |
 |
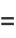 |
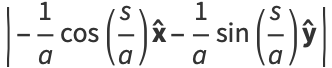 |
(40) |
 |
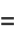 |
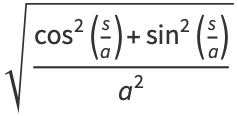 |
(41) |
 |
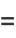 |
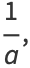 |
(42) |
as expected.
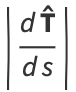
Four very important derivative relations in differential geometry related to the Frenet formulas are
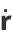 |
 |
 |
(43) |
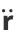 |
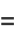 |
 |
(44) |
 |
 |
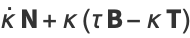 |
(45) |
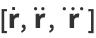 |
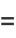 |
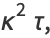 |
(46) |
where 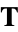 is the tangent vector,
is the tangent vector,  is the normal vector,
is the normal vector, 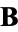 is the binormal vector, and
is the binormal vector, and  is the torsion (Coxeter 1969, p. 322).
is the torsion (Coxeter 1969, p. 322).
The curvature at a point on a surface takes on a variety of values as the plane through the normal varies. As 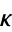 varies, it achieves a minimum and a maximum (which are in perpendicular directions) known as the principal curvatures. As shown in Coxeter (1969, pp. 352-353),
varies, it achieves a minimum and a maximum (which are in perpendicular directions) known as the principal curvatures. As shown in Coxeter (1969, pp. 352-353),
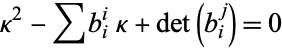 |
(47) |
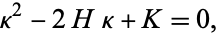 |
(48) |
where  is the Gaussian curvature,
is the Gaussian curvature, 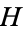 is the mean curvature, and det denotes the determinant.
is the mean curvature, and det denotes the determinant.
The curvature 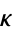 is sometimes called the first curvature and the torsion
is sometimes called the first curvature and the torsion 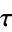 the second curvature. In addition, a third curvature(sometimes called total curvature)
the second curvature. In addition, a third curvature(sometimes called total curvature)
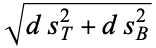 |
(49) |
is also defined. A signed version of the curvature of a circle appearing in the Descartes circle theorem for the radius of the fourth of four mutually tangent circles is called the bend.
REFERENCES:
Casey, J. Exploring Curvature. Wiesbaden, Germany: Vieweg, 1996.
Coxeter, H. S. M. Introduction to Geometry, 2nd ed. New York: Wiley, 1969.
Fischer, G. (Ed.). Plates 79-85 in Mathematische Modelle aus den Sammlungen von Universitäten und Museen, Bildband. Braunschweig, Germany: Vieweg, pp. 74-81, 1986.
Gray, A. "Curvature of Curves in the Plane," "Drawing Plane Curves with Assigned Curvature," and "Drawing Space Curves with Assigned Curvature." §1.5, 6.4, and 10.2 in Modern Differential Geometry of Curves and Surfaces with Mathematica, 2nd ed. Boca Raton, FL: CRC Press, pp. 14-17, 140-146, and 222-224, 1997.
Kreyszig, E. "Principal Normal, Curvature, Osculating Circle." §12 in Differential Geometry. New York: Dover, pp. 34-36, 1991.
Yates, R. C. "Curvature." A Handbook on Curves and Their Properties. Ann Arbor, MI: J. W. Edwards, pp. 60-64, 1952.
 الاكثر قراءة في التفاضل و التكامل
الاكثر قراءة في التفاضل و التكامل
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة

الآخبار الصحية















 قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة
قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة "المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة
"المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة (نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)


















