Lerch Transcendent
المؤلف:
Guillera, J. and Sondow, J.
المصدر:
"Double Integrals and Infinite Products for Some Classical Constants Via Analytic Continuations of Lerch,s Transcendent." 16 June 2005
الجزء والصفحة:
...
21-8-2018
2140
Lerch Transcendent
The Lerch transcendent is generalization of the Hurwitz zeta function and polylogarithm function. Many sums of reciprocal powers can be expressed in terms of it. It is classically defined by
 |
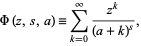(1)
|
for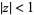 
and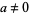 
, 
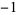, .... It is implemented in this form as HurwitzLerchPhi[z, s, a] in the Wolfram Language.
The slightly different form
![Phi^*(z,s,a)=sum_(k=0)^infty(z^k)/([(a+k)^2]^(s/2))](http://mathworld.wolfram.com/images/equations/LerchTranscendent/NumberedEquation2.gif) |
(2)
|
sometimes also denoted 
, for 
(or 
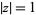and ![R[s]>1](http://mathworld.wolfram.com/images/equations/LerchTranscendent/Inline7.gif)
) and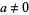 
, 
, 
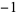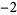, ..., is implemented in the Wolfram Language as LerchPhi[z, s, a]. Note that the two are identical only for ![R[a]>0](http://mathworld.wolfram.com/images/equations/LerchTranscendent/Inline11.gif)
.
A series formula for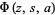 
valid on a larger domain in the complex 
-plane is given by
 |
(3)
|
which holds for all complex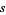 
and complex 
with ![R[z]<1/2](http://mathworld.wolfram.com/images/equations/LerchTranscendent/Inline16.gif)
(Guillera and Sondow 2005).
The Lerch transcendent can be used to express the Dirichlet beta function
A special case is given by
 |
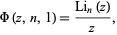(6)
|
(Guillera and Sondow 2005), where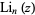 
is the polylogarithm.
Special cases giving simple constants include
where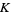 
is Catalan's constant, 
is Apéry's constant, and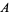 
is the Glaisher-Kinkelin constant (Guillera and Sondow 2005).
It gives the integrals of the Fermi-Dirac distribution
where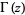 
is the gamma function and 
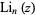is the polylogarithm and Bose-Einstein distribution
Double integrals involving the Lerch transcendent include
![int_0^1int_0^1(x^(u-1)y^(v-1))/(1-xyz)[-ln(xy)]^sdxdy
=Gamma(s+1)(Phi(z,s+1,v)-Phi(z,s+1,u))/(u-v)
int_0^1int_0^1((xy)^(u-1))/(1-xyz)[-ln(xy)]^sdxdy
=Gamma(s)Phi(z,s+2,u),](http://mathworld.wolfram.com/images/equations/LerchTranscendent/NumberedEquation5.gif) |
(15)
|
where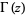 
is the gamma function. These formulas lead to a variety of beautiful special cases of unit square integrals (Guillera and Sondow 2005).
It also can be used to evaluate Dirichlet L-series.
REFERENCES:
Erdélyi, A.; Magnus, W.; Oberhettinger, F.; and Tricomi, F. G. "The Function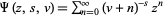 
." §1.11 in Higher Transcendental Functions, Vol. 1. New York: Krieger, pp. 27-31, 1981.
Gradshteyn, I. S. and Ryzhik, I. M. "The Lerch Function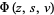 
." §9.55 in Tables of Integrals, Series, and Products, 6th ed. San Diego, CA: Academic Press, p. 1029, 2000.
Guillera, J. and Sondow, J. "Double Integrals and Infinite Products for Some Classical Constants Via Analytic Continuations of Lerch's Transcendent." 16 June 2005 http://arxiv.org/abs/math.NT/0506319.
الاكثر قراءة في التفاضل و التكامل
اخر الاخبار
اخبار العتبة العباسية المقدسة
