Min   Max       Re       Im

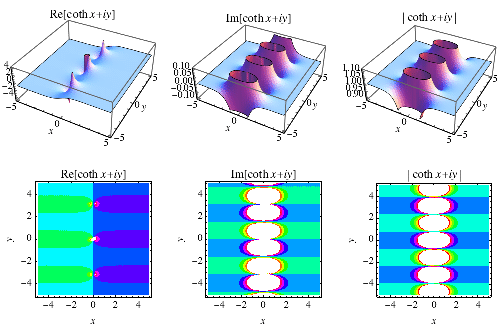The hyperbolic cotangent is defined as

(1)

The notation  is sometimes also used (Gradshteyn and Ryzhik 2000, p. xxix). It is implemented in the Wolfram Language as Coth[z].

The hyperbolic cotangent satisfies the identity

(2)

where  is the hyperbolic cosecant.

It has a unique real fixed point where

(3)

at  (OEIS A085984), which is related to the Laplace limit in the solution of Kepler's equation.

The derivative is given by

(4)

where  is the hyperbolic cosecant, and the indefinite integral by

(5)

where  is a constant of integration.

The Laurent series of  is given by

			(6)
			(7)

(OEIS A002431 and A036278), where  is a Bernoulli number and  is a Bernoulli polynomial. An asymptotic series about infinity on the real line is given by

(8)

REFERENCES:

Abramowitz, M. and Stegun, I. A. (Eds.). "Hyperbolic Functions." §4.5 in Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, pp. 83-86, 1972.

Gradshteyn, I. S. and Ryzhik, I. M. Tables of Integrals, Series, and Products, 6th ed. San Diego, CA: Academic Press, 2000.

Jeffrey, A. "Hyperbolic Identities." §2.5 in Handbook of Mathematical Formulas and Integrals, 2nd ed. Orlando, FL: Academic Press, pp. 117-122, 2000.

Sloane, N. J. A. Sequences A002431/M0124 and A036278 in "The On-Line Encyclopedia of Integer Sequences."

Spanier, J. and Oldham, K. B. "The Hyperbolic Tangent  and Cotangent  Functions." Ch. 30 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 279-284, 1987.

Zwillinger, D. (Ed.). "Hyperbolic Functions." §6.7 in CRC Standard Mathematical Tables and Formulae. Boca Raton, FL: CRC Press, pp. 476-481 1995.

Sloane, N. J. A. Sequences A010050 and A085984 in "The On-Line Encyclopedia of Integer Sequences."