

تاريخ الرياضيات

الاعداد و نظريتها

تاريخ التحليل

تار يخ الجبر

الهندسة و التبلوجي


الرياضيات في الحضارات المختلفة

العربية

اليونانية

البابلية

الصينية

المايا

المصرية

الهندية


الرياضيات المتقطعة

المنطق

اسس الرياضيات

فلسفة الرياضيات

مواضيع عامة في المنطق


الجبر

الجبر الخطي

الجبر المجرد

الجبر البولياني

مواضيع عامة في الجبر

الضبابية

نظرية المجموعات

نظرية الزمر

نظرية الحلقات والحقول

نظرية الاعداد

نظرية الفئات

حساب المتجهات

المتتاليات-المتسلسلات

المصفوفات و نظريتها

المثلثات


الهندسة

الهندسة المستوية

الهندسة غير المستوية

مواضيع عامة في الهندسة

التفاضل و التكامل


المعادلات التفاضلية و التكاملية

معادلات تفاضلية

معادلات تكاملية

مواضيع عامة في المعادلات


التحليل

التحليل العددي

التحليل العقدي

التحليل الدالي

مواضيع عامة في التحليل

التحليل الحقيقي

التبلوجيا

نظرية الالعاب

الاحتمالات و الاحصاء

نظرية التحكم

بحوث العمليات

نظرية الكم

الشفرات

الرياضيات التطبيقية

نظريات ومبرهنات


علماء الرياضيات

500AD

500-1499

1000to1499

1500to1599

1600to1649

1650to1699

1700to1749

1750to1779

1780to1799

1800to1819

1820to1829

1830to1839

1840to1849

1850to1859

1860to1864

1865to1869

1870to1874

1875to1879

1880to1884

1885to1889

1890to1894

1895to1899

1900to1904

1905to1909

1910to1914

1915to1919

1920to1924

1925to1929

1930to1939

1940to the present

علماء الرياضيات

الرياضيات في العلوم الاخرى

بحوث و اطاريح جامعية

هل تعلم

طرائق التدريس

الرياضيات العامة

نظرية البيان
Heaviside Step Function
المؤلف:
Abramowitz, M. and Stegun, I. A.
المصدر:
Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, 1972.
الجزء والصفحة:
...
26-9-2019
2117
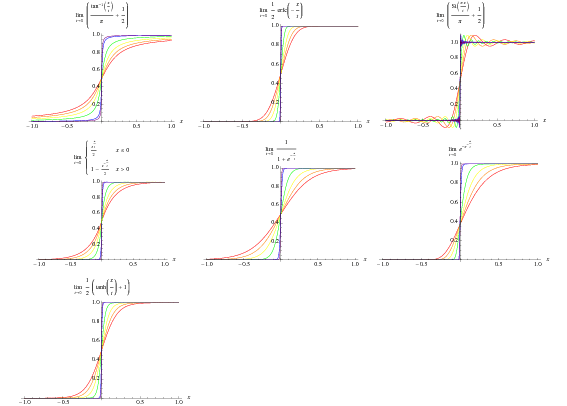
The Heaviside step function is a mathematical function denoted  , or sometimes
, or sometimes 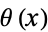 or
or 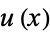 (Abramowitz and Stegun 1972, p. 1020), and also known as the "unit step function." The term "Heaviside step function" and its symbol can represent either a piecewise constant function or a generalized function.
(Abramowitz and Stegun 1972, p. 1020), and also known as the "unit step function." The term "Heaviside step function" and its symbol can represent either a piecewise constant function or a generalized function.
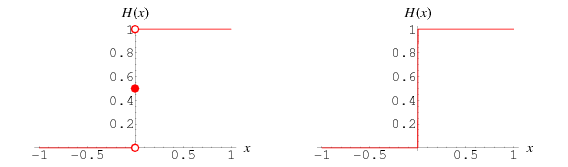
When defined as a piecewise constant function, the Heaviside step function is given by
|
(1) |
(Abramowitz and Stegun 1972, p. 1020; Bracewell 2000, p. 61). The plot above shows this function (left figure), and how it would appear if displayed on an oscilloscope (right figure).
When defined as a generalized function, it can be defined as a function 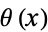 such that
such that
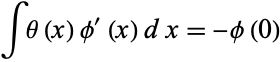 |
(2) |
for 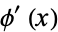 the derivative of a sufficiently smooth function
the derivative of a sufficiently smooth function 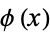 that decays sufficiently quickly (Kanwal 1998).
that decays sufficiently quickly (Kanwal 1998).
The Wolfram Language represents the Heaviside generalized function as HeavisideTheta, while using UnitStep to represent the piecewise function Piecewise[{" src="http://mathworld.wolfram.com/images/equations/HeavisideStepFunction/Inline7.gif" style="height:15px; width:5px" />
{" src="http://mathworld.wolfram.com/images/equations/HeavisideStepFunction/Inline8.gif" style="height:15px; width:5px" />1, x >= 0
}" src="http://mathworld.wolfram.com/images/equations/HeavisideStepFunction/Inline9.gif" style="height:15px; width:5px" />
}" src="http://mathworld.wolfram.com/images/equations/HeavisideStepFunction/Inline10.gif" style="height:15px; width:5px" />] (which, it should be noted, adopts the convention
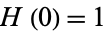 instead of the conventional definition
instead of the conventional definition 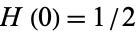 ).
).
The shorthand notation
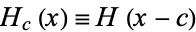 |
(3) |
is sometimes also used.
The Heaviside step function is related to the boxcar function by
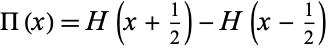 |
(4) |
and can be defined in terms of the sign function by
![H(x)=1/2[1+sgn(x)].](http://mathworld.wolfram.com/images/equations/HeavisideStepFunction/NumberedEquation5.gif) |
(5) |
The derivative of the step function is given by
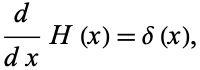 |
(6) |
where 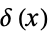 is the delta function (Bracewell 2000, p. 97).
is the delta function (Bracewell 2000, p. 97).
The Heaviside step function is related to the ramp function  by
by
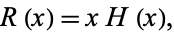 |
(7) |
and to the derivative of 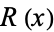 by
by
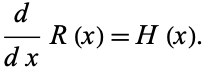 |
(8) |
The two are also connected through
 |
(9) |
where 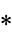 denotes convolution.
denotes convolution.
Bracewell (2000) gives many identities, some of which include the following. Letting 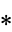 denote the convolution,
denote the convolution,
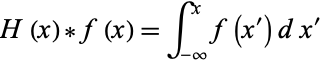 |
(10) |
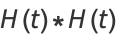 |
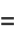 |
 |
(11) |
 |
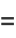 |
 |
(12) |
 |
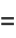 |
 |
(13) |
 |
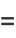 |
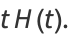 |
(14) |
In addition,
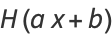 |
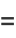 |
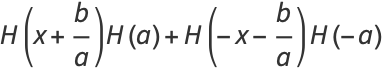 |
(15) |
 |
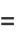 |
(16) |
The Heaviside step function can be defined by the following limits,
 |
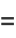 |
![lim_(t->0)[1/2+1/pitan^(-1)(x/t)]](http://mathworld.wolfram.com/images/equations/HeavisideStepFunction/Inline38.gif) |
(17) |
 |
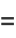 |
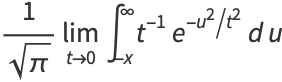 |
(18) |
 |
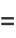 |
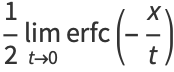 |
(19) |
 |
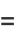 |
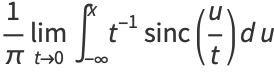 |
(20) |
 |
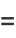 |
 |
(21) |
 |
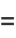 |
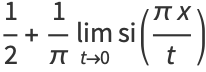 |
(22) |
 |
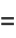 |
(23) |
|
 |
 |
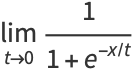 |
(24) |
 |
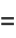 |
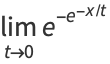 |
(25) |
 |
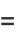 |
![1/2lim_(t->0)[1+tanh(x/t)]](http://mathworld.wolfram.com/images/equations/HeavisideStepFunction/Inline65.gif) |
(26) |
 |
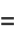 |
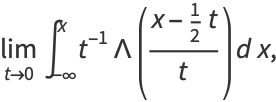 |
(27) |
where 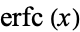 is the erfc function,
is the erfc function, 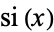 is the sine integral,
is the sine integral,  is the sinc function, and
is the sinc function, and  is the one-argument triangle function. The first four of these are illustrated above for
is the one-argument triangle function. The first four of these are illustrated above for 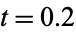 , 0.1, and 0.01.
, 0.1, and 0.01.
Of course, any monotonic function with constant unequal horizontal asymptotes is a Heaviside step function under appropriate scaling and possible reflection. The Fourier transform of the Heaviside step function is given by
![F[H(x)]](http://mathworld.wolfram.com/images/equations/HeavisideStepFunction/Inline74.gif) |
 |
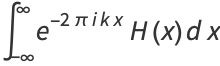 |
(28) |
 |
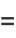 |
![1/2[delta(k)-i/(pik)],](http://mathworld.wolfram.com/images/equations/HeavisideStepFunction/Inline79.gif) |
(29) |
where 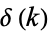 is the delta function.
is the delta function.
REFERENCES:
Abramowitz, M. and Stegun, I. A. (Eds.). Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, 1972.
Bracewell, R. "Heaviside's Unit Step Function, 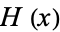 ." The Fourier Transform and Its Applications, 3rd ed. New York: McGraw-Hill, pp. 61-65, 2000.
." The Fourier Transform and Its Applications, 3rd ed. New York: McGraw-Hill, pp. 61-65, 2000.
Kanwal, R. P. Generalized Functions: Theory and Technique, 2nd ed. Boston, MA: Birkhäuser, 1998.
Spanier, J. and Oldham, K. B. "The Unit-Step 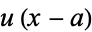 and Related Functions." Ch. 8 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 63-69, 1987.
and Related Functions." Ch. 8 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 63-69, 1987.