An algorithm which finds a polynomial recurrence for terminating hypergeometric identities of the form

where 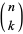 is a binomial coefficient, 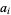, , 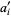, , 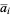,  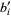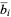are constant integers 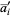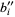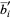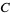and , , , , , , and 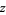 are complex numbers (Zeilberger 1990). The method was called creative telescoping by van der Poorten (1979), and led to the development of the amazing machinery of Wilf-Zeilberger pairs.

The also exists a 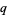-analog of the algorithm, called the q-Zeilberger algorithm.

REFERENCES:

Graham, R. L.; Knuth, D. E.; and Patashnik, O. Concrete Mathematics: A Foundation for Computer Science, 2nd ed. Reading, MA: Addison-Wesley, 1994.

Koepf, W. "Algorithms for 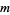-fold Hypergeometric Summation." J. Symb. Comput. 20, 399-417, 1995.

Koepf, W. "Zeilberger's Algorithm." Ch. 7 in Hypergeometric Summation: An Algorithmic Approach to Summation and Special Function Identities. Braunschweig, Germany: Vieweg, pp. 93-123, 1998.

Krattenthaler, C. "HYP and HYPQ: The Mathematica Package HYP." http://radon.mat.univie.ac.at/People/kratt/hyp_hypq/hyp.html.

Paule, P. "The Paule/Schorn Implementation of Gosper's and Zeilberger's Algorithms." http://www.risc.uni-linz.ac.at/research/combinat/risc/software/PauleSchorn/.

Paule, P. and Riese, A. "A Mathematica 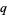-Analogue of Zeilberger's Algorithm Based on an Algebraically Motivated Approach to 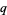-Hypergeometric Telescoping." In Special Functions, 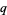-Series and Related Topics, Fields Institute Communications 14, 179-210, 1997.

Paule, P. and Schorn, M. "A Mathematica Version of Zeilberger's Algorithm for Proving Binomial Coefficient Identities." J. Symb. Comput. 20, 673-698, 1995.

Petkovšek, M.; Wilf, H. S.; and Zeilberger, D. "Zeilberger's Algorithm." Ch. 6 in A=B. Wellesley, MA: A K Peters, pp. 101-119, 1996. http://www.cis.upenn.edu/~wilf/AeqB.html.

Riese, A. "A Generalization of Gosper's Algorithm to Bibasic Hypergeometric Summation." Electronic J. Combinatorics 1, No. 1, R19, 1-16, 1996. http://www.combinatorics.org/Volume_1/Abstracts/v1i1r19.html.

van der Poorten, A. "A Proof that Euler Missed... Apéry's Proof of the Irrationality of 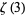." Math. Intel. 1, 196-203, 1979.

Wegschaider, K. Computer Generated Proofs of Binomial Multi-Sum Identities. Diploma Thesis, RISC. Linz, Austria: J. Kepler University, May 1997. http://www.risc.uni-linz.ac.at/research/combinat/risc/software/MultiSum/.

Zeilberger, D. "Doron Zeilberger's Maple Packages and Programs: EKHAD." http://www.math.temple.edu/~zeilberg/programs.html.

Zeilberger, D. "A Fast Algorithm for Proving Terminating Hypergeometric Series Identities." Discrete Math. 80, 207-211, 1990.

Zeilberger, D. "A Holonomic Systems Approach to Special Function Identities." J. Comput. Appl. Math. 32, 321-368, 1990.

Zeilberger, D. "The Method of Creative Telescoping." J. Symb. Comput. 11, 195-204, 1991.