

تاريخ الرياضيات

الاعداد و نظريتها

تاريخ التحليل

تار يخ الجبر

الهندسة و التبلوجي


الرياضيات في الحضارات المختلفة

العربية

اليونانية

البابلية

الصينية

المايا

المصرية

الهندية


الرياضيات المتقطعة

المنطق

اسس الرياضيات

فلسفة الرياضيات

مواضيع عامة في المنطق


الجبر

الجبر الخطي

الجبر المجرد

الجبر البولياني

مواضيع عامة في الجبر

الضبابية

نظرية المجموعات

نظرية الزمر

نظرية الحلقات والحقول

نظرية الاعداد

نظرية الفئات

حساب المتجهات

المتتاليات-المتسلسلات

المصفوفات و نظريتها

المثلثات


الهندسة

الهندسة المستوية

الهندسة غير المستوية

مواضيع عامة في الهندسة

التفاضل و التكامل


المعادلات التفاضلية و التكاملية

معادلات تفاضلية

معادلات تكاملية

مواضيع عامة في المعادلات


التحليل

التحليل العددي

التحليل العقدي

التحليل الدالي

مواضيع عامة في التحليل

التحليل الحقيقي

التبلوجيا

نظرية الالعاب

الاحتمالات و الاحصاء

نظرية التحكم

بحوث العمليات

نظرية الكم

الشفرات

الرياضيات التطبيقية

نظريات ومبرهنات


علماء الرياضيات

500AD

500-1499

1000to1499

1500to1599

1600to1649

1650to1699

1700to1749

1750to1779

1780to1799

1800to1819

1820to1829

1830to1839

1840to1849

1850to1859

1860to1864

1865to1869

1870to1874

1875to1879

1880to1884

1885to1889

1890to1894

1895to1899

1900to1904

1905to1909

1910to1914

1915to1919

1920to1924

1925to1929

1930to1939

1940to the present

علماء الرياضيات

الرياضيات في العلوم الاخرى

بحوث و اطاريح جامعية

هل تعلم

طرائق التدريس

الرياضيات العامة

نظرية البيان
Absolute Value
المؤلف:
Sloane, N. J. A
المصدر:
Sequences A000217/M2535, A116419, and A116420 in "The On-Line Encyclopedia of Integer Sequences."
الجزء والصفحة:
...
13-8-2018
1869
|
Foundations of Mathematics |
Absolute Value
The absolute value of a real number
where
The absolute value of a complex number
This form is implemented in the Wolfram Language as Abs[z] and is illustrated above for complex Note that the derivative (read: complex derivative)
As a result of the fact that computer algebra languages such as the Wolfram Language generically deal with complex variables (i.e., the definition of derivative always means complex derivative), Note that the notation The notations for the floor function The unit square integral of the absolute value of the difference of two variables taken to the power
for Similarly, for
giving the first few values for REFERENCES: Milton, K. A. "The Casimir Effect: Physical Manifestations of Zero-Point Energy." 4 Jan 1999. http://arxiv.org/abs/hep-th/9901011. Milton, K. A. and Ng, J. "Observability of the Bulk Casimir Effect: Can the Dynamical Casimir Effect be Relevant to Sonoluminescence?" Phys. Rev. E 57, 5504-5510, 1998. Sloane, N. J. A. Sequences A000217/M2535, A116419, and A116420 in "The On-Line Encyclopedia of Integer Sequences." |
 الاكثر قراءة في التفاضل و التكامل
الاكثر قراءة في التفاضل و التكامل
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة

الآخبار الصحية















 is denoted
is denoted 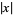 and defined as the "unsigned" portion of
and defined as the "unsigned" portion of  ,
,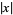
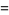


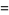
 is the sign function. The absolute value is therefore always greater than or equal to 0. The absolute value of
is the sign function. The absolute value is therefore always greater than or equal to 0. The absolute value of  for real
for real  is plotted above.
is plotted above.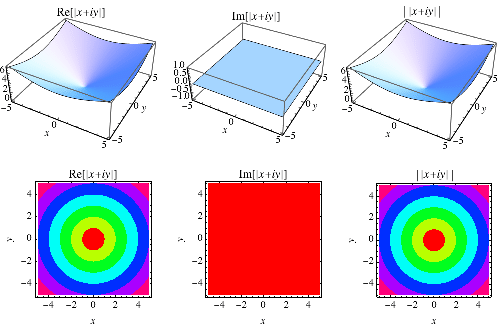
 , also called the complex modulus, is defined as
, also called the complex modulus, is defined as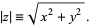
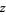 .
.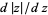 does not exist because at every point in the complex plane, the value of the derivative of
does not exist because at every point in the complex plane, the value of the derivative of 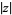 depends on the direction in which the derivative is taken (so the Cauchy-Riemann equationscannot and do not hold). However, the real derivative (i.e., restricting the derivative to directions along the real axis) can be defined for points other than
depends on the direction in which the derivative is taken (so the Cauchy-Riemann equationscannot and do not hold). However, the real derivative (i.e., restricting the derivative to directions along the real axis) can be defined for points other than 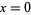 as
as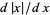 correctly returns unevaluated by such software.
correctly returns unevaluated by such software. is commonly used to denote the complex modulus, p-adic norm, or general valuation. In this work, the norm of a vector
is commonly used to denote the complex modulus, p-adic norm, or general valuation. In this work, the norm of a vector  is also denoted
is also denoted 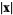 , although the notation
, although the notation 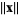 is also in common use.
is also in common use. , nearest integer function
, nearest integer function ![[x]](http://mathworld.wolfram.com/images/equations/AbsoluteValue/Inline24.gif) , and ceiling function
, and ceiling function ![[x]](http://mathworld.wolfram.com/images/equations/AbsoluteValue/Inline25.gif) are similar to that used for the absolute value.
are similar to that used for the absolute value.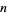 is given by
is given by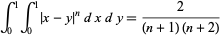
![R[n]>-1](http://mathworld.wolfram.com/images/equations/AbsoluteValue/Inline27.gif) , which has values for
, which has values for 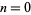 , 1, ... of 1, 1/3, 1/6, 1/10, 1/15, 1/21, ..., i.e., one over the triangular numbers(OEIS A000217), for
, 1, ... of 1, 1/3, 1/6, 1/10, 1/15, 1/21, ..., i.e., one over the triangular numbers(OEIS A000217), for 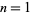 , 2, .... This sort of integral arises in the study of the Casimir effect (Milton and Ng 1998, eqn. 3.15; Milton 1999, p. 32, eqn. 3.33).
, 2, .... This sort of integral arises in the study of the Casimir effect (Milton and Ng 1998, eqn. 3.15; Milton 1999, p. 32, eqn. 3.33).![R[n]>-2](http://mathworld.wolfram.com/images/equations/AbsoluteValue/Inline30.gif) ,
,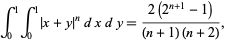
 , 1, ... of 1, 1, 7/6, 3/2, 31, 15, 3, ... (OEIS A116419 and A116420).
, 1, ... of 1, 1, 7/6, 3/2, 31, 15, 3, ... (OEIS A116419 and A116420).
 "المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة
"المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة (نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام) قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى)
قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى)


















